A chain of length 'L' and mass 'M' is hanging by fixing its upper end to a rigid support. The tension in the chain at a distance 'x' from the rigid support is
Zero
Mg
Mg(L−x)L
Mg(L−x)x
The correct Answer is:C
To find the tension in a chain of length L and mass M hanging from a rigid support at a distance x from the support, we can follow these steps:
1. Understanding the Setup:
- We have a chain of length L and mass M hanging vertically from a rigid support.
- We need to find the tension T at a point P which is at a distance x from the support.
2. Identifying the Length Below Point P:
- The length of the chain that is hanging below point P is L−x. This is because the total length of the chain is L and the distance from the support to point P is x.
3. Calculating the Weight of the Chain Segment:
- The weight of the segment of the chain that is hanging below point P can be calculated using the formula:
Weight=mass×gravity=(M/L)×g×(L−x)
- Here, M/L is the mass per unit length of the chain, and g is the acceleration due to gravity.
4. Finding the Tension at Point P:
- The tension T at point P is equal to the weight of the segment of the chain that is hanging below it. Therefore:
T=Weight of the segment below P=(ML)×g×(L−x)
5. Final Expression for Tension:
- Thus, the tension in the chain at a distance x from the rigid support is given by:
T=M⋅g⋅(L−x)L
Summary:
The tension in the chain at a distance x from the rigid support is:
T=M⋅g⋅(L−x)L
- We have a chain of length L and mass M hanging vertically from a rigid support.
- We need to find the tension T at a point P which is at a distance x from the support.
- The length of the chain that is hanging below point P is L−x. This is because the total length of the chain is L and the distance from the support to point P is x.
- The weight of the segment of the chain that is hanging below point P can be calculated using the formula:
Weight=mass×gravity=(M/L)×g×(L−x)
- Here, M/L is the mass per unit length of the chain, and g is the acceleration due to gravity.
- The tension T at point P is equal to the weight of the segment of the chain that is hanging below it. Therefore:
T=Weight of the segment below P=(ML)×g×(L−x)
- Thus, the tension in the chain at a distance x from the rigid support is given by:
T=M⋅g⋅(L−x)L
The tension in the chain at a distance x from the rigid support is:
T=M⋅g⋅(L−x)L
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - II
136 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
10 Videos
- LOGIC GATESBOOK - AAKASH SERIESCHAPTER - LOGIC GATESEXERCISE - Exercise (Very Short Answer)
10 Videos
Similar Questions
A chain of mass M and length L is held vertical by fixing its upper end to a rigid support. The tension in the chain at a distance y from the rigid support is:
View SolutionA chain of mass M and length L is held vertical by fixing its upper end to a rigid support. The tension in the chain at a distance y from the rigid support is:
View Solution
Knowledge Check
A cord of mass m length L, area of cross section A and Young's modulus y is hanging from a ceiling with the help of a rigid support. The elogation developed in the wire due to its own weight is
Aμgl2YABμgl22YAC2μgl2YAD2μgl23YA
A vertical hanging bar of length l and mass m per unit length carries a load of mass M at lower end, its upper end is clamped to a rigid support.The tensile stress a distance x from support is (A→ area of cross - section of bar)
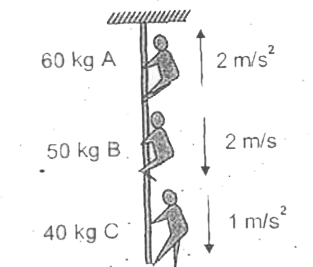
View SolutionTension in the rope at the rigid support is (g=10m/s2)
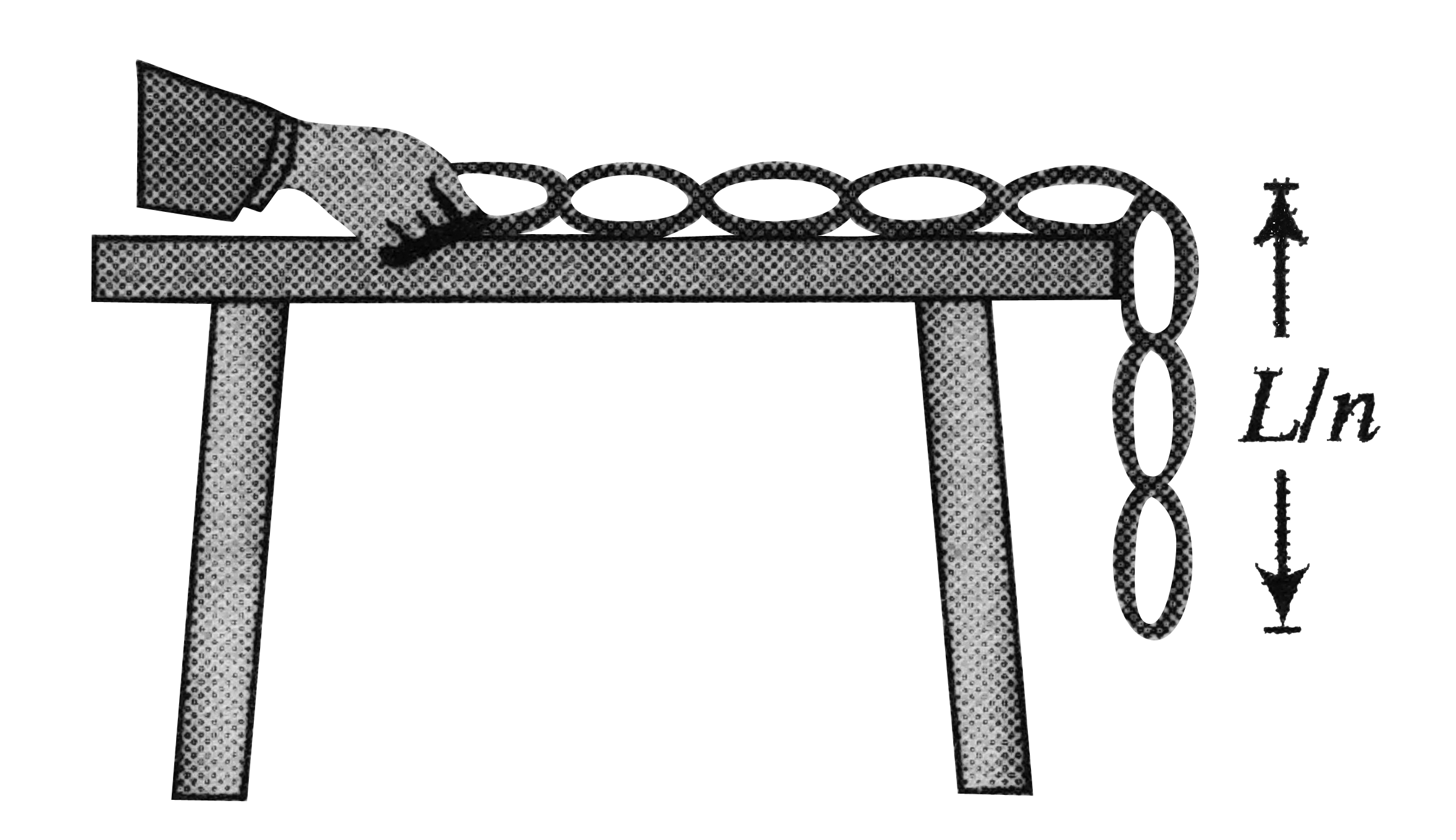
View SolutionA chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
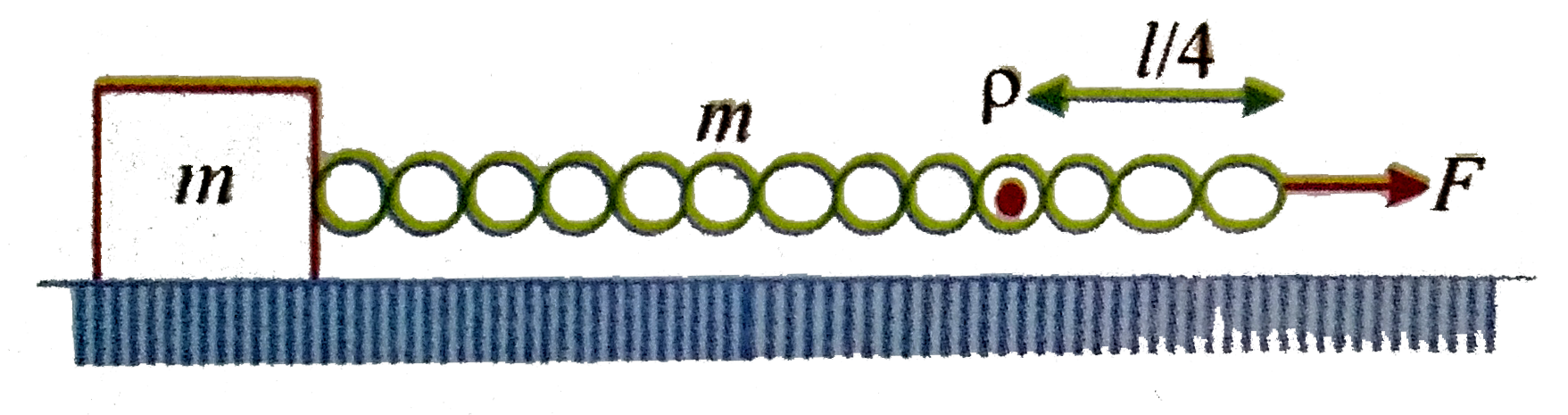
View SolutionA block of mass m is pulled by a uniform chain of mass m tied to it by applying a force F at the other end of the chain.The tension at a point P which is at a distance of quarter of the length of the chain from the free end,will be
View SolutionA wave is reflected from a rigid support. The change in phase on reflection will be
View SolutionA wire of length L,area of cross section A is hanging from a fixed support. The length of the wire changes to L1 when mass M is suspended from its free end. The expression for Young's modulus is:
View SolutionA flexible chain of weight W hangs between two fixed points A and B at the same level. The inclination of the chain with the horizontal at the two points of support is θ. Calculated the tension of the chain at end points and also at the lower most point .
[Hints : Consider equilibrium of each half of the chain. ]
View Solution

