In the above problem, what force should the man exert on the rope to get his correct weight on the machine?
To solve the problem of what force the man should exert on the rope to get his correct weight on the machine, we need to analyze the forces acting on him.
1. Understanding the Situation:
The man is standing on a weighing machine, and he is pulling on a rope. When he pulls the rope, he exerts a force that affects the reading on the weighing machine.
2. Identify Forces:
The forces acting on the man include:
- His weight (W = mg, where m is his mass and g is the acceleration due to gravity).
- The upward force exerted by the weighing machine (N).
- The force exerted by the man on the rope (F).
3. Applying Newton's Second Law:
When the man pulls on the rope, he creates a tension (T) in the rope. According to Newton's second law, the net force acting on him can be expressed as:
N−W+F=0
Rearranging gives:
N=W−F
4. Correct Weight on the Machine:
To read his correct weight on the machine, the normal force (N) should equal his actual weight (W). Therefore, we set:
N=W
Substituting this into the previous equation gives:
W=W−F
5. Solving for the Force (F):
Rearranging the equation:
F=0
This means that to get his correct weight on the machine, the man should not exert any force on the rope.
Conclusion:
The man should exert a force of 0 N on the rope to get his correct weight on the machine.
The man is standing on a weighing machine, and he is pulling on a rope. When he pulls the rope, he exerts a force that affects the reading on the weighing machine.
The forces acting on the man include:
- His weight (W = mg, where m is his mass and g is the acceleration due to gravity).
- The upward force exerted by the weighing machine (N).
- The force exerted by the man on the rope (F).
When the man pulls on the rope, he creates a tension (T) in the rope. According to Newton's second law, the net force acting on him can be expressed as:
N−W+F=0
Rearranging gives:
N=W−F
To read his correct weight on the machine, the normal force (N) should equal his actual weight (W). Therefore, we set:
N=W
Substituting this into the previous equation gives:
W=W−F
Rearranging the equation:
F=0
This means that to get his correct weight on the machine, the man should not exert any force on the rope.
The man should exert a force of 0 N on the rope to get his correct weight on the machine.
Similar Questions
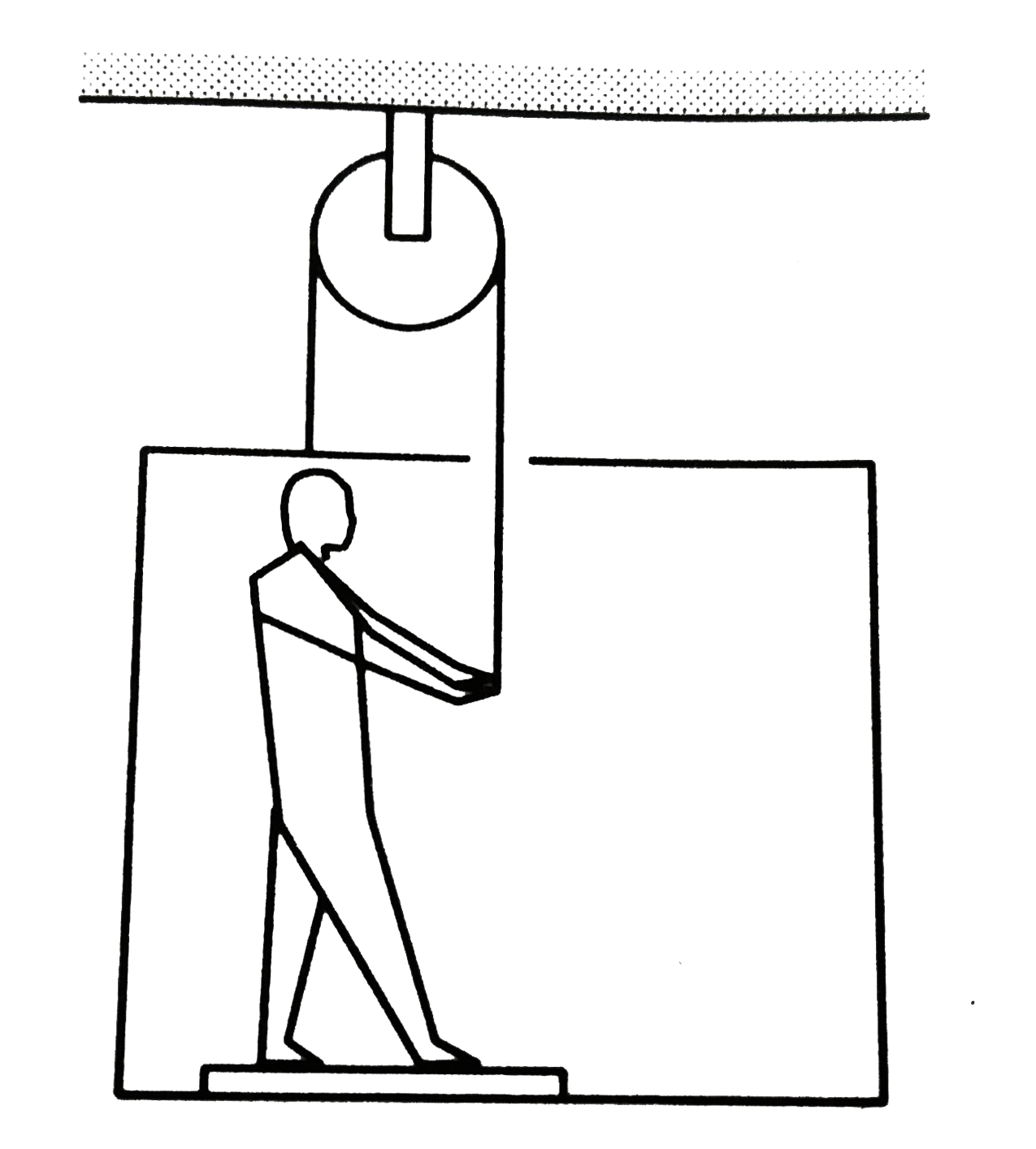
Figure shows a man of mass 60 kg standing on a light weighting machine kept in a box of mass 30 kg. The box is hanging from a pulley fixed to the ceiling through a light rope, the other end of which is held by the man himself. If the man manages to keep the box at rest, what is the weight shown by the machine? What force should he exert on the rope to get his correct weight on the machine?
View SolutionIn the above problem, what is the angle of projection with horizontal ?
View SolutionIn the above problem further choose the correct options.
View SolutionThe friction coefficient between the board and the floor shown in figure is μ Find the maximum force that the man can exert on the rope so that the board does not slip on the floor
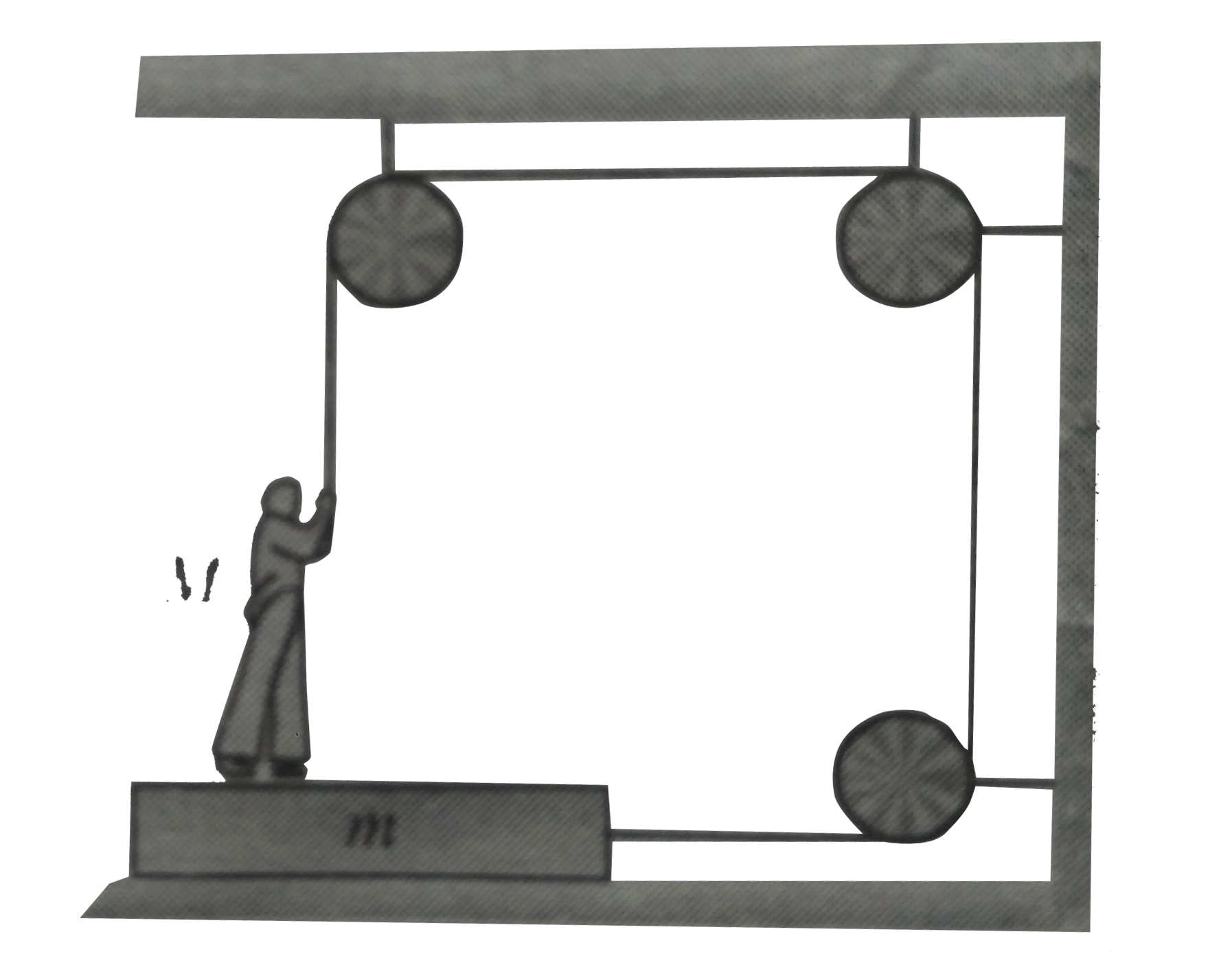
View SolutionThe friction coefficient between the board and the floor shown in figure is μ Find the maximum force that the man can exert on the rope so that the board does not slip on the floor

View SolutionIn the above problem, direction of friction force is
View SolutionIn the above problem, when the man has walked once around the platform, so that he is at his original position on it, what is his angular displacement relative to ground?
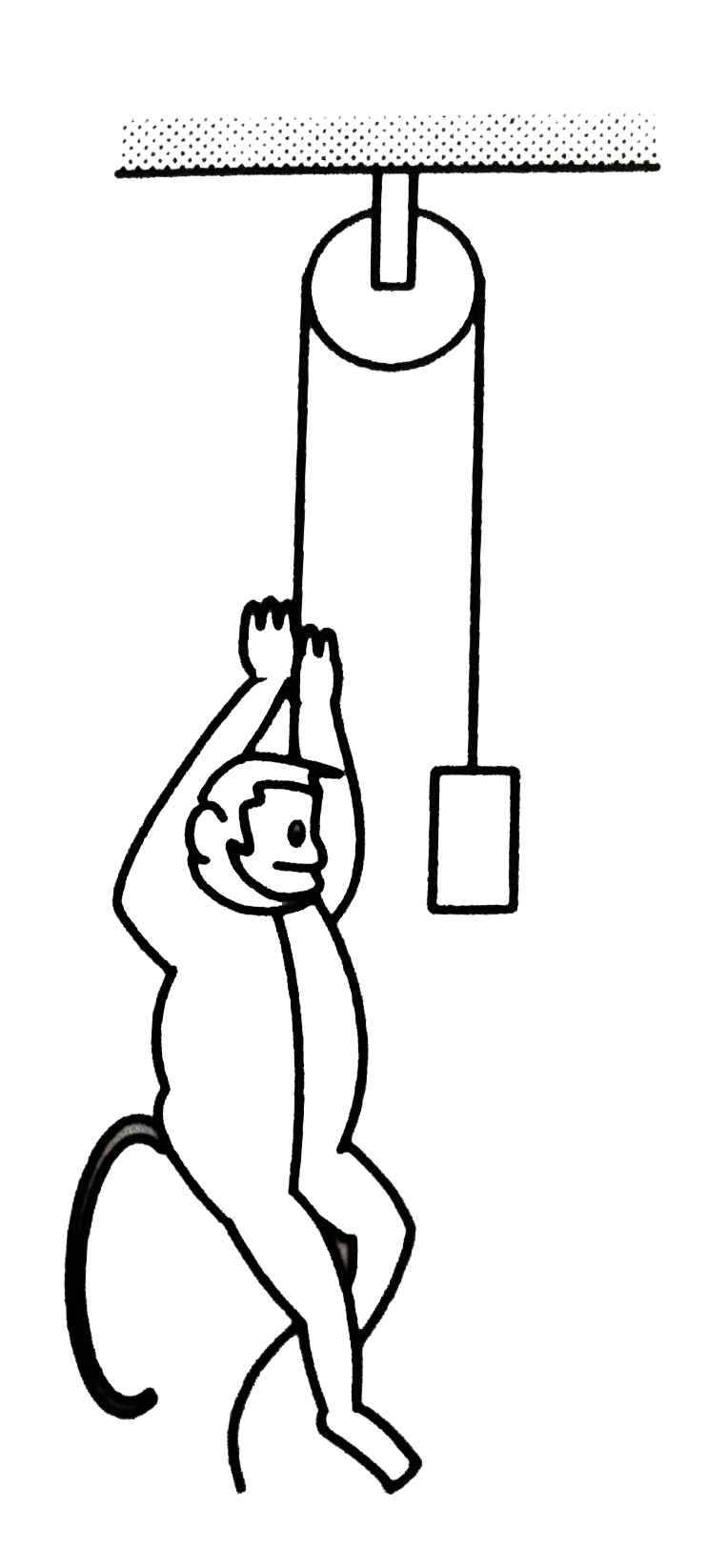
View SolutionA Monkey is Climbing on a Rope that Goes Over a Smooth Light Pulley and Supports a Block of Equal Mass at the Other End in the Following
View SolutionForce exerted on unit area of a surface by the weight of the air above it
View SolutionThe friction coeficient bettween the board and the floor shownin filgure is μ. Find the maximum force that the man can exert on the rope so tht the board does not slipon the floor.

View Solution
