Give the magnitude and direction of the net force acting on
a high - speed electron in space far from all material objects, and free of electric and magnetic field .
To solve the problem, we need to analyze the situation of a high-speed electron in space, far from any material objects, and free from electric and magnetic fields. Here’s a step-by-step breakdown of the solution:
Step 1: Identify the Forces Acting on the Electron
In the given scenario, the electron is in space, which means it is not influenced by any external forces. The forces we typically consider are:
- Gravitational force (due to mass)
- Electric force (due to electric fields)
- Magnetic force (due to magnetic fields)
Step 2: Analyze the Absence of Forces
Since the problem states that the electron is far from all material objects and is free from electric and magnetic fields, we can conclude:
- Electric Force (F_e): There are no electric fields acting on the electron, so the electric force is zero.
- Magnetic Force (F_m): There are no magnetic fields present, so the magnetic force is also zero.
- Gravitational Force (F_g): In space, especially far from any massive objects, the gravitational force can be considered negligible.
Step 3: Calculate the Net Force
Since all the forces acting on the electron are zero, we can calculate the net force (F_net) as follows:
Fnet=Fe+Fm+Fg
Given that Fe=0, Fm=0, and Fg≈0:
Fnet=0+0+0=0
Step 4: Conclusion
The magnitude of the net force acting on the high-speed electron is:
Magnitude=0N
And since there is no net force, the direction is also undefined.
Final Answer
The magnitude of the net force acting on the high-speed electron is 0 N, and the direction is undefined.
---
In the given scenario, the electron is in space, which means it is not influenced by any external forces. The forces we typically consider are:
- Gravitational force (due to mass)
- Electric force (due to electric fields)
- Magnetic force (due to magnetic fields)
Since the problem states that the electron is far from all material objects and is free from electric and magnetic fields, we can conclude:
- Electric Force (F_e): There are no electric fields acting on the electron, so the electric force is zero.
- Magnetic Force (F_m): There are no magnetic fields present, so the magnetic force is also zero.
- Gravitational Force (F_g): In space, especially far from any massive objects, the gravitational force can be considered negligible.
Since all the forces acting on the electron are zero, we can calculate the net force (F_net) as follows:
Fnet=Fe+Fm+Fg
Given that Fe=0, Fm=0, and Fg≈0:
Fnet=0+0+0=0
The magnitude of the net force acting on the high-speed electron is:
Magnitude=0N
And since there is no net force, the direction is also undefined.
The magnitude of the net force acting on the high-speed electron is 0 N, and the direction is undefined.
Similar Questions
Give the magnitude and direction of the net force acting on
a Cork of mass 10 g floating on water
View SolutionGive the magnitude and direction of the net force acting on
(a) a drop of rain falling down with a constant speed
(b) a cork of mass 10 g floating on water
(c) a kite skillfully held stationary in the sky
(d) a car moving with a constant velocity of 30kh/h on a rough road
(e) a high speed electron in space free from all gravitational objects and free of electric and magnetic fields.
View SolutionGive the magnitude and direction of the net force acting on
a kite skillfully held stationary in the sky
View SolutionGive the magnitude and direction of the net force acting on
a drop of rain falling down with a constant speed
View SolutionGive the magnitude and direction of the net force acting on
a car moving with a constant velocity of 30km/h on a rough road
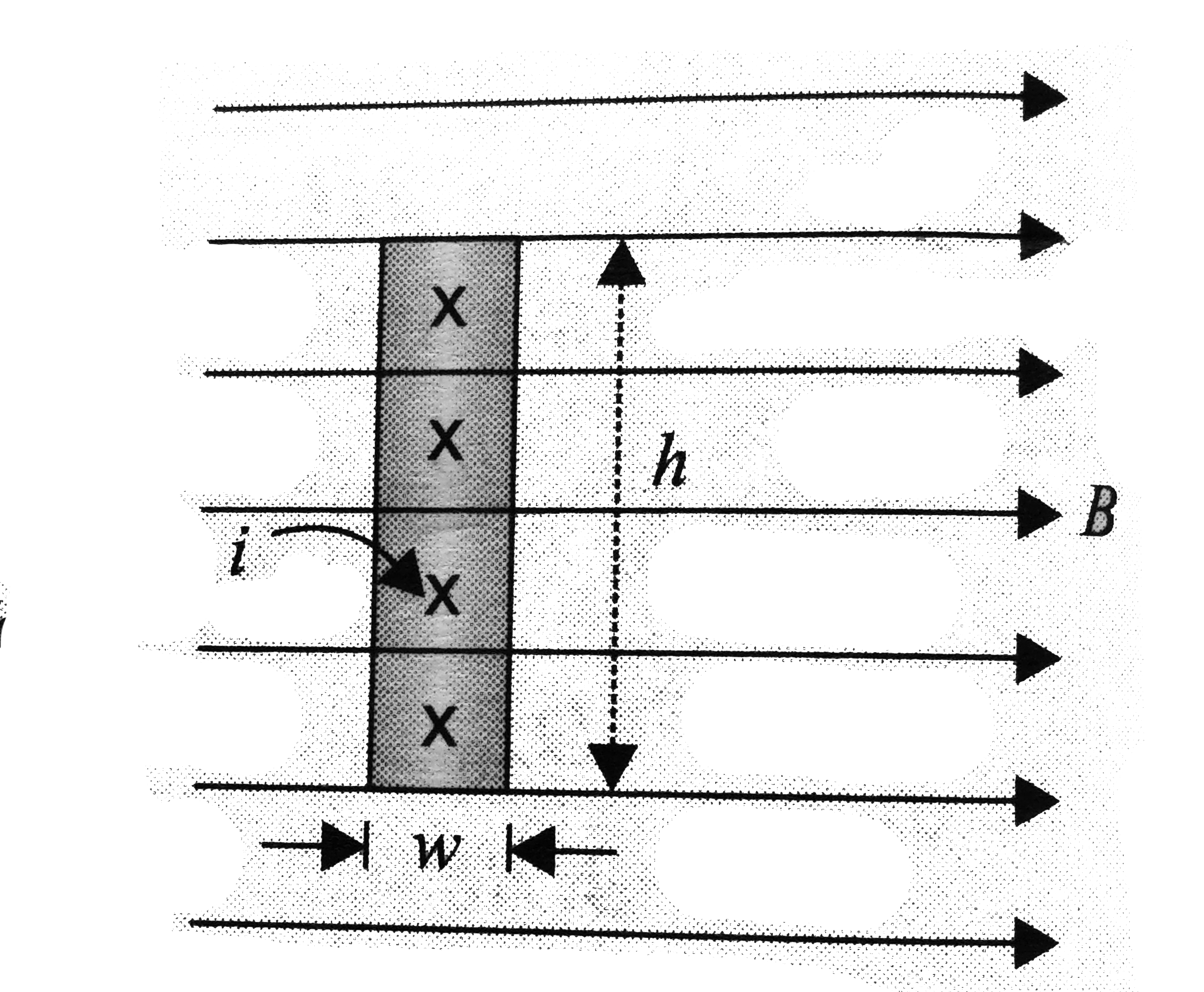
View SolutionA current i, indicated by the crosses in figure, is established in a strip of copper of height h and width w. A uniform field of magnetic induction B is applied at right angle to the strip.
(a) Calculate the drift velocity vd of the electrons.
(b) What are the magnitude and direction of the magnetic force F acting on the electrons?
(c) What should the magnitude and direction of a homogeneous electric field E be in order to counterbalance the effect of mangetic field?
(d) Calculate voltage V necessary between two sides of the conductor in order to creat this field E Between which sides of the conductor would this voltage have to be applied?
(e) If no electric field is applied from the outside, the electrons will be pushed somewhat to one side and therefore will give rise to a uniform electric field EH across the conductor until the forces of this electrostatic field EH balance the magnetic forces encountered in part (b). What will be the magnitude and direction of field EH?
Assume that n, the number of conductor electrons per unit volume is 1.1×1029m−3,h=0.02m,w=0.1cm,i=50A,andB=2T.
View SolutionThe cube as shown in Fig. 1.61, 75.0 cm on a side, is placed in a uniform magnetic field of 0.860 T parallel to the x-axis. The wire abcdef carries a current of 6.58 A in the direction indicated.
(a) Determine the magnitude and direction of the force acting on the segment ab.
(b) Determine the magnitude and direction of the force acting on the sement bc.
(c) Determine the magnitude and direction of the force acting on the segment cd.
(d) Determine the magnitude and direction of the force acting on the segment de.
(e) Determine the magnitude and direction of the force acting on the segment ef.
(f) What are the magnitude and direction of the total force on the wire ?

View SolutionFind the magnitude and direction of force on following current carrying wires. Direction of magnetic field is indicated in each case.
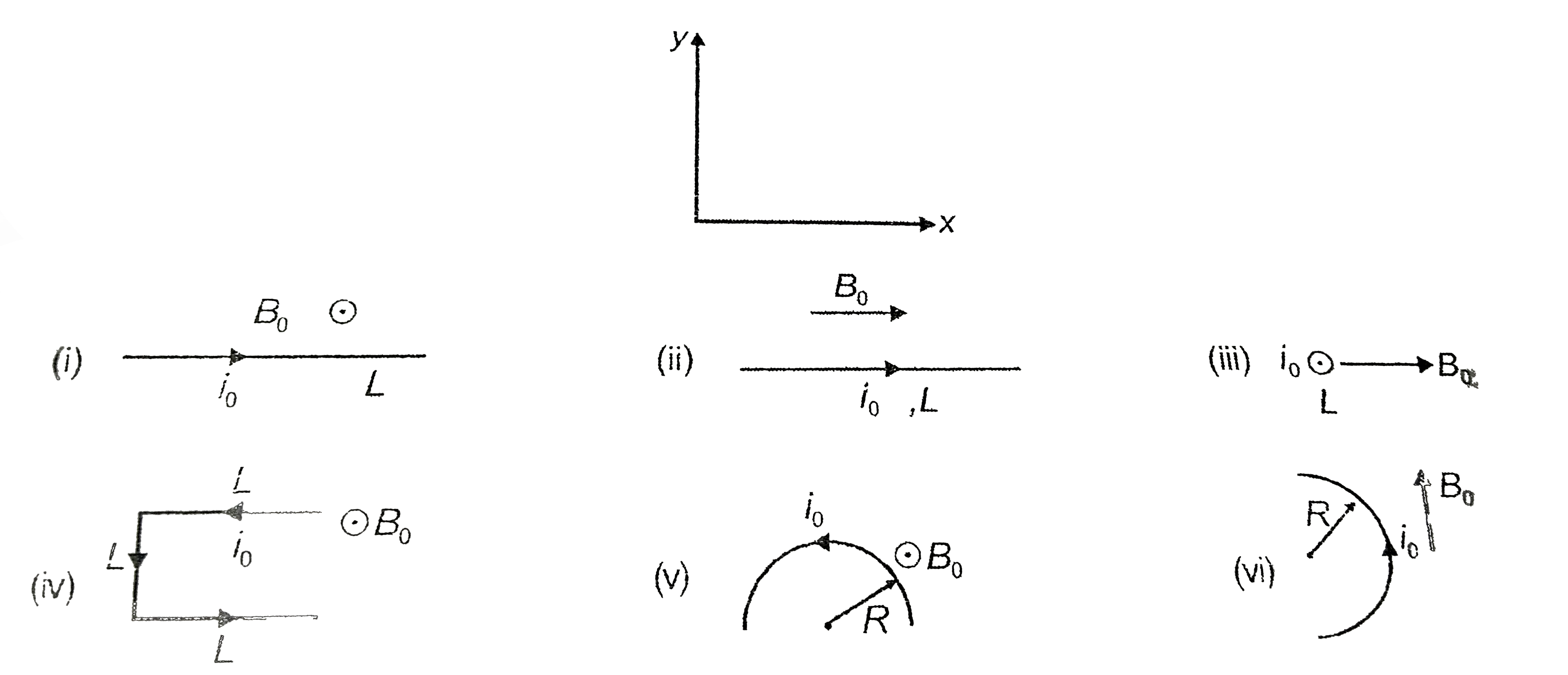
View SolutionWhat is the direction of the force acting on a charged particle q, moving with a velocity →v a uniform magnetic field →B ?
View SolutionAn electron at point A in figure has a speed v0=1.41×106ms. Find
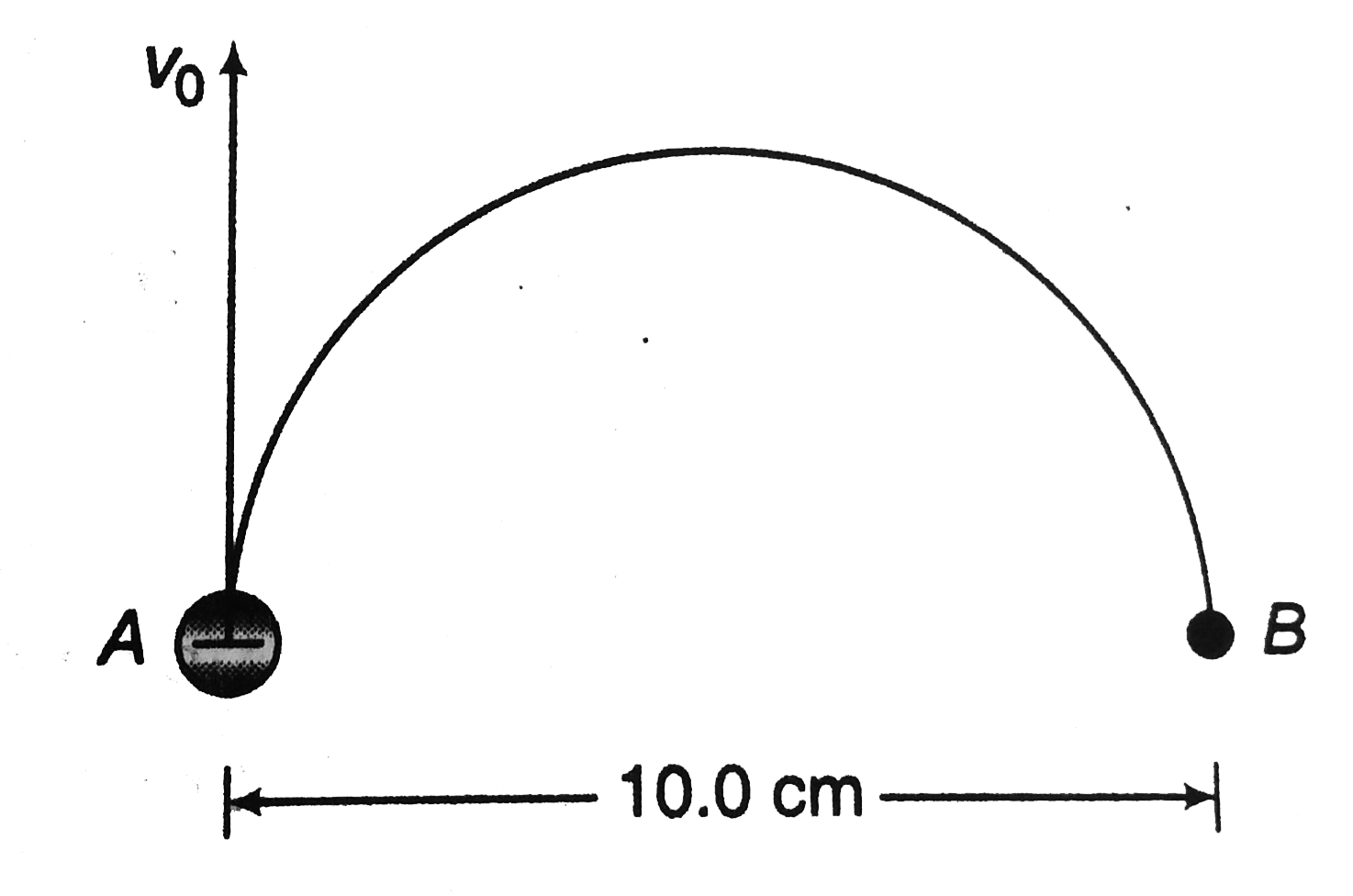
(a) the magnitude and direction of the magnetic field that will cause the electron to follow the semicircular path from A to B,
(b) the time required for the electron to move from A to B.
View Solution
