When a motor cyclist takes a U-turn in 4s what is the average angular velocity of the motor cyclist?
To find the average angular velocity of the motorcyclist taking a U-turn in 4 seconds, we can follow these steps:
1. Understand the U-turn:
- A U-turn can be visualized as the motorcyclist moving along a semicircular path.
- The angle covered during a U-turn is π radians (180 degrees).
2. Identify the time taken:
- The time taken for the U-turn is given as 4 seconds.
3. Use the formula for average angular velocity:
- The average angular velocity (ω) can be calculated using the formula:
ω=ΔθΔt
- Where Δθ is the change in angular displacement (in radians) and Δt is the change in time (in seconds).
4. Substitute the values:
- Here, Δθ=π radians and Δt=4 seconds.
- So, substituting the values into the formula gives:
ω=π4
5. Calculate the numerical value:
- Using π≈3.14:
ω=3.144≈0.785 radians per second
6. Conclusion:
- The average angular velocity of the motorcyclist is approximately 0.785 radians per second.
- A U-turn can be visualized as the motorcyclist moving along a semicircular path.
- The angle covered during a U-turn is π radians (180 degrees).
- The time taken for the U-turn is given as 4 seconds.
- The average angular velocity (ω) can be calculated using the formula:
ω=ΔθΔt
- Where Δθ is the change in angular displacement (in radians) and Δt is the change in time (in seconds).
- Here, Δθ=π radians and Δt=4 seconds.
- So, substituting the values into the formula gives:
ω=π4
- Using π≈3.14:
ω=3.144≈0.785 radians per second
- The average angular velocity of the motorcyclist is approximately 0.785 radians per second.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - SHORT ANSWER TYPE QUESTIONS
6 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
10 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Circular Motion)
7 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
Similar Questions
When a constant torque is applied, a wheel is turned from rest through 200 radians in 10 s. What is its angular acceleration. If the same torque continues to act what is the angular velocity of the wheel after 15 s from the start ?
View SolutionA rotating, rigid body makes one complete revolution in 2s. What is its average angular velocity?
View Solution
Knowledge Check
When a cyclist turns on a turn, he
Abends outwardBbends inwardsCbends forwardDdoes not bend at allA cyclist bends while taking turn to
Areduce frictionBgenerate required centripetal forceCreduce apparent weightDreduce speedA motor cyclist is going in a vertical circle what is the necessary condition so that he may not fall down ?
Athe force of gravity disappears.Bhe loses weight some how.Che is kept in this path due to the force exerted by surrounding air.Dthe frictional force of the wall balances his weight
The motor of an engine is rotating about its axis with an angular velocity of 100 rpm. It comes to rest in 15 s after being switched off. Assuming constant angular deceleration, calculate the number of revolution made by it before coming to rest.
View SolutionAn object moves 10 m in 4 s then turns left and moves 20 m in next 5 s. What is the average speed of the object?
View SolutionThe motor of an engine is rotating about its axis with an angular velocity of 100 rev/minute. It comes to rest in 15 s, after being switched off. Assuming constant angular deceleration, calculate the number of revolutions made by it before coming to rest.
View SolutionA man moves on his motorbike with 54km/h and then takes a U-turn and containues to move with same velocity The time of U-turn is 10s. Find the magnitude of average acceleration during U-turn.
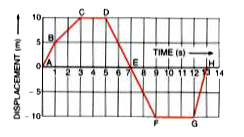
View SolutionFrom the displacement-time graph of a cyclist, given in Fig. 2.39, find :
(i) the average velocity in the first 4 s,
(ii) the displacement from the initial position at the end of 10 s,
(iii) the time after which he reaches the starting point.
View Solution
