Two object A and B are moving each with velocities 10 m/s. A is moving towards East and B is moving towards North from the same point as shown. Find velocity of A relative to B (→VAB)
To find the velocity of object A relative to object B, we can follow these steps:
Step 1: Define the velocities of A and B
- Object A is moving towards the East with a velocity of →VA=10m/s^i.
- Object B is moving towards the North with a velocity of →VB=10m/s^j.
Step 2: Write the formula for relative velocity
The velocity of A relative to B is given by:
→VAB=→VA−→VB
Step 3: Substitute the velocities into the formula
Substituting the values of →VA and →VB:
→VAB=(10^i)−(10^j)
This can be rewritten as:
→VAB=10^i−10^j
Step 4: Find the magnitude of the relative velocity
To find the magnitude of →VAB, we use the Pythagorean theorem:
|→VAB|=√(10)2+(−10)2=√100+100=√200=10√2m/s
Step 5: Determine the direction of the relative velocity
The direction can be found using the tangent function:
tan(θ)=|opposite||adjacent|=1010=1
Thus, θ=45∘ from the East towards the North-West direction.
Final Result
The velocity of A relative to B is:
→VAB=10√2m/sat an angle of 45∘ from the East towards the North-West.
---
- Object A is moving towards the East with a velocity of →VA=10m/s^i.
- Object B is moving towards the North with a velocity of →VB=10m/s^j.
The velocity of A relative to B is given by:
→VAB=→VA−→VB
Substituting the values of →VA and →VB:
→VAB=(10^i)−(10^j)
This can be rewritten as:
→VAB=10^i−10^j
To find the magnitude of →VAB, we use the Pythagorean theorem:
|→VAB|=√(10)2+(−10)2=√100+100=√200=10√2m/s
The direction can be found using the tangent function:
tan(θ)=|opposite||adjacent|=1010=1
Thus, θ=45∘ from the East towards the North-West direction.
The velocity of A relative to B is:
→VAB=10√2m/sat an angle of 45∘ from the East towards the North-West.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - SHORT ANSWER TYPE QUESTIONS
6 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
10 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Circular Motion)
7 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
Similar Questions
Out of the two cars A and B car A is moving towards east with velocity of 10 m/s whereas B is moving towards north with a velocity 20 m/s, then velocity of A w.r.t B is ( nearly )
View SolutionAn object A is moving with 5 m/s and B is moving with 20 m/s in the direction . (positive x-axis )
find velocity of A with respect to B .
View SolutionTwo cars A and B start moving from the same point with same velocity v=5km/minute. Car A moves towards North and car B in moving towards East. What is the relative velocity of B with respect to A ?
View SolutionA plane mirror and a person are moving towards each other with same velocity v. Then the velocity of the image is
View SolutionAn object A is moving with 5 m/s and B is moving with 20 m/s in the same direction. (Positive x-axis). Find a) velocity of B relative to A.
b) Velocity of A relative to B
View SolutionA car is moving with velocity →vc towards north and a train is moving towards east with velocity →vt . Find the direction of relative velocity of car with respect to train.
View SolutionSystem is shown in fig. and wedge is moving toward left with speed 2ms−1. Find the velocity of the block B.
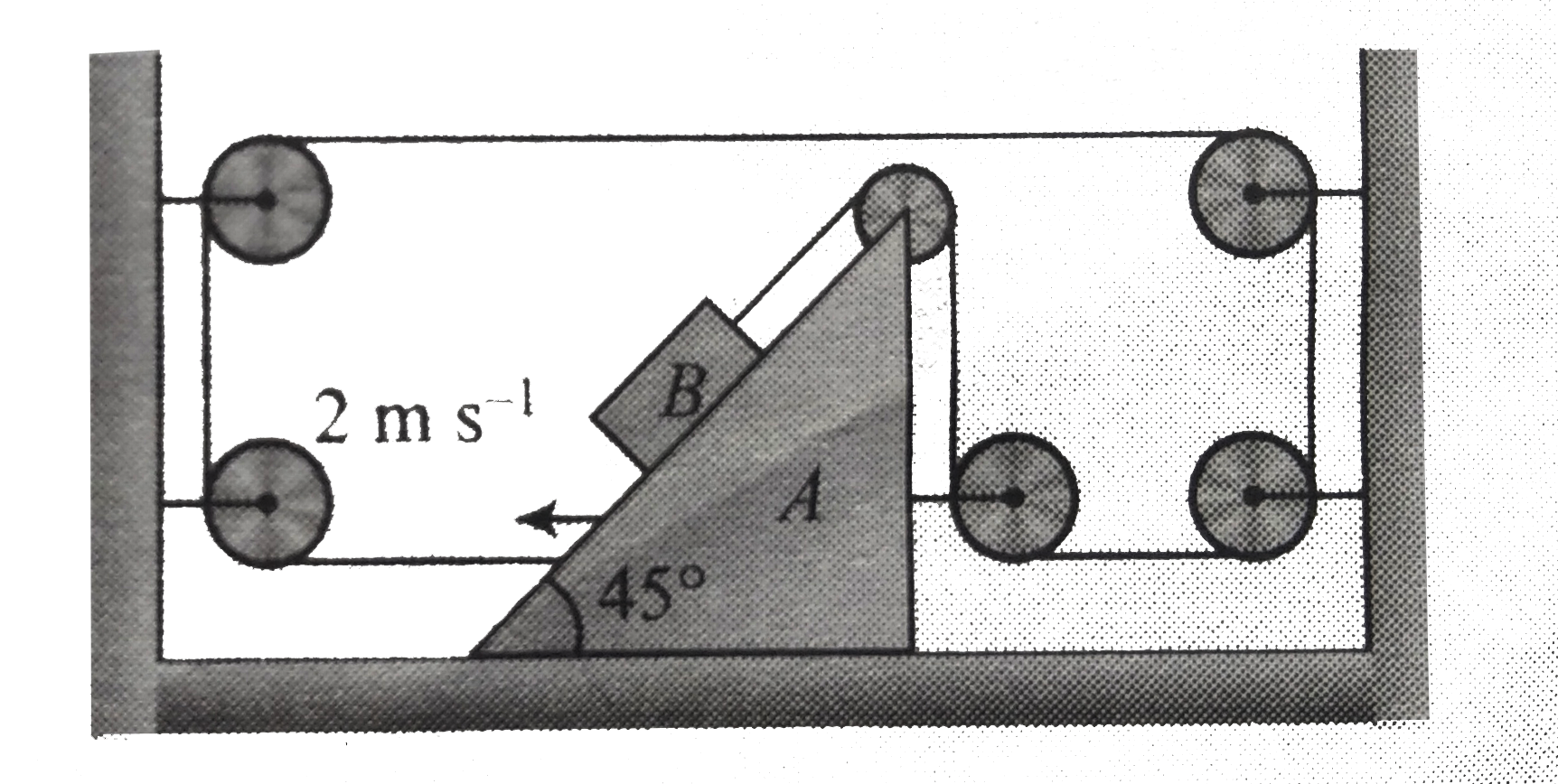
View SolutionTwo particles A and B moving in a plane have velocities as shown in figure .Relative velocity of A w.r.t B
View SolutionAn object is moving towards a convex mirror with a constant velocity. P is the pole of mirror.
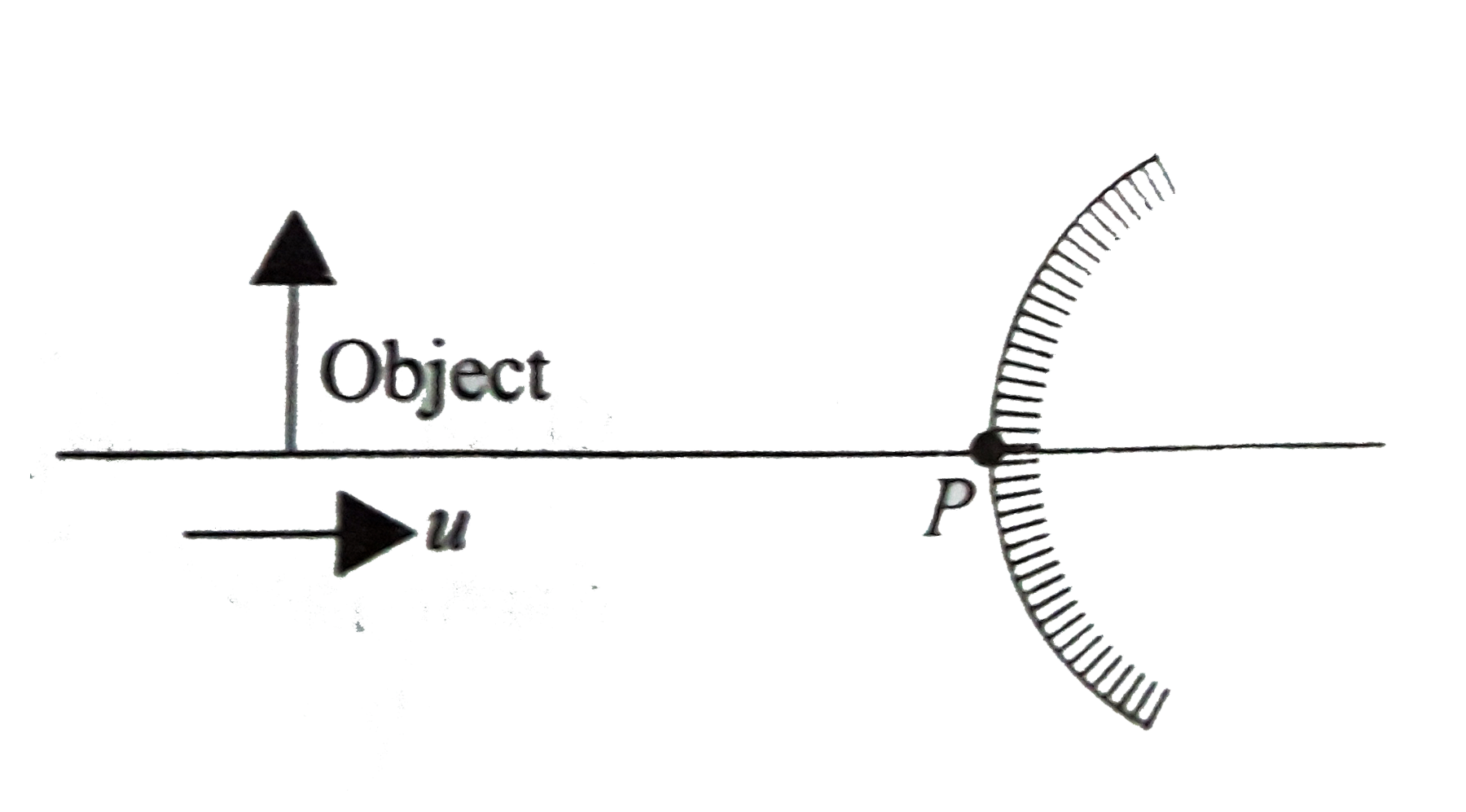
View SolutionFind the velocity of the image when a plane mirror and a person are moving towards each other with same velocity v.
View Solution
