A projectile shot at angles of 45∘ above the horizontal strikes a building 30 m away at a point 15 m above the point of projection. Find : (a) the speed of projection . (b) the magnitude and direction of velocity of projectile when it strikes the building .
To solve the problem step by step, we will break it down into two parts: (a) finding the speed of projection and (b) finding the magnitude and direction of the velocity of the projectile when it strikes the building.
1. Equation of Trajectory:
The equation for the trajectory of a projectile is given by:
y=xtanθ−gx22u2cos2θ
Substituting the known values:
15=30tan(45∘)−9.8⋅3022u2cos2(45∘)
2. Calculating tan(45∘) and cos(45∘):
Since tan(45∘)=1 and cos(45∘)=1√2:
15=30⋅1−9.8⋅9002u2⋅(1√2)2
3. Simplifying the Equation:
15=30−9.8⋅900u2
Rearranging gives:
9.8⋅900u2=30−15
9.8⋅900u2=15
4. Solving for u2:
u2=9.8⋅90015
u2=882015=588
5. Finding u:
u=√588≈24.2m/s
Part (b): Finding the Magnitude and Direction of Velocity
1. Horizontal Component of Velocity:
The horizontal component of velocity vx is given by:
vx=ucos(45∘)=24.2⋅1√2≈17.11m/s
2. Vertical Component of Velocity:
We can find the vertical component of velocity vy using the following kinematic equation:
v2y=u2y+2aysy
Where:
- uy=usin(45∘)=24.2⋅1√2
- ay=−g=−9.8m/s2
- sy=15m
Substituting the values:
v2y=(24.2⋅1√2)2−2⋅9.8⋅15
v2y=5882−294
v2y=294−294=0
Thus, vy=0.
3. Magnitude of Velocity:
The magnitude of the velocity v when it strikes the building is:
v=√v2x+v2y=√(17.11)2+02=17.11m/s
4. Direction of Velocity:
The direction of the velocity can be found using:
tan(θ)=vyvx=017.11=0
This indicates that the projectile is moving horizontally when it strikes the building.
Final Answers:
(a) The speed of projection u≈24.2m/s
(b) The magnitude of velocity when it strikes the building is 17.11m/s and the direction is horizontal.
The equation for the trajectory of a projectile is given by:
y=xtanθ−gx22u2cos2θ
Substituting the known values:
15=30tan(45∘)−9.8⋅3022u2cos2(45∘)
Since tan(45∘)=1 and cos(45∘)=1√2:
15=30⋅1−9.8⋅9002u2⋅(1√2)2
15=30−9.8⋅900u2
Rearranging gives:
9.8⋅900u2=30−15
9.8⋅900u2=15
u2=9.8⋅90015
u2=882015=588
u=√588≈24.2m/s
The horizontal component of velocity vx is given by:
vx=ucos(45∘)=24.2⋅1√2≈17.11m/s
We can find the vertical component of velocity vy using the following kinematic equation:
v2y=u2y+2aysy
Where:
- uy=usin(45∘)=24.2⋅1√2
- ay=−g=−9.8m/s2
- sy=15m
v2y=(24.2⋅1√2)2−2⋅9.8⋅15
v2y=5882−294
v2y=294−294=0
Thus, vy=0.
The magnitude of the velocity v when it strikes the building is:
v=√v2x+v2y=√(17.11)2+02=17.11m/s
The direction of the velocity can be found using:
tan(θ)=vyvx=017.11=0
This indicates that the projectile is moving horizontally when it strikes the building.
(a) The speed of projection u≈24.2m/s
(b) The magnitude of velocity when it strikes the building is 17.11m/s and the direction is horizontal.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - SHORT ANSWER TYPE QUESTIONS
6 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
10 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Circular Motion)
7 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
Similar Questions
A projectile is fired at an angle of 45∘ with the horizontal. Elevation angle of the projection at its highest point as seen from the point of projection is
View SolutionA projectile, thrown with velocity v0 at an angle α to the horizontal, has a range R. it will strike a vertical wall at a distance R/2 from the point of projection with a speed of
View Solution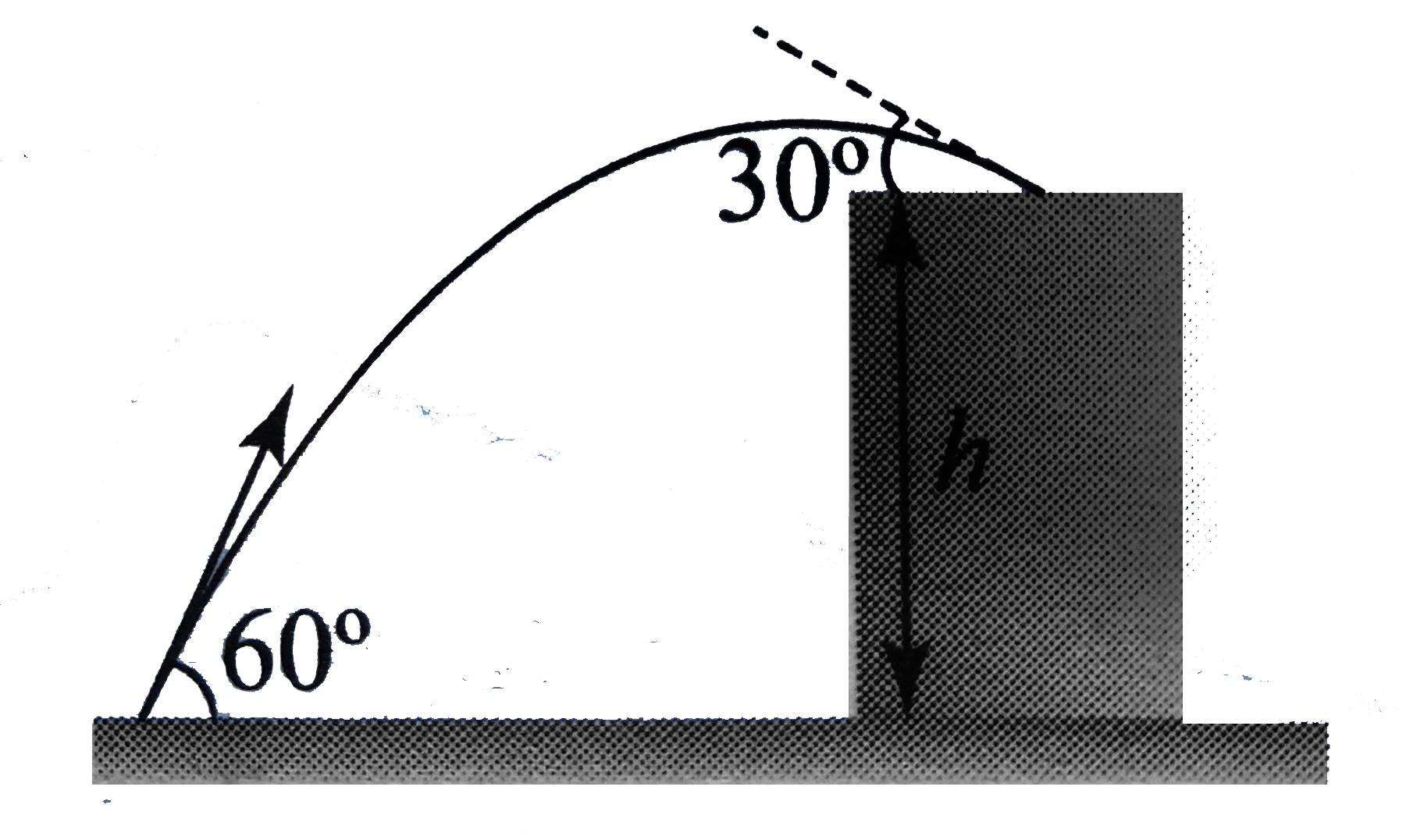
A stone projected at an angle of 60∘ from the ground level strikes at an angle of 30∘ on the roof of a building of height h. Then the speed of projection of the stone is:
View Solution
A stone projected at an angle of 60∘ from the ground level strikes at an angle of 30∘ on the roof of a building of height h. Then the speed of projection of the stone is:
View SolutionThe ratio of the speed of a projectile at the point of projection to the speed at the top of its trajectory is x. The angle of projection with the horizontal is
View SolutionA ball is projected from a point on the floor wilth a speed of 15 m/s at an angle of 600 with the horizontal. Will ilt hit a vertical wall 5 m away from the point of projection and perpendiculaer to the plane of projection without and perpendicular to the plane of projection without hitting the floor? will the answer differ if the wall is 22 m away?
View SolutionA ball is projected with 20√2m/s at angle 45∘ with horizontal.The angular velocity of the particle at highest point of its journey about point of projection is
View SolutionA ball is projected at an angle of 30∘ above with the horizontal from the top of a tower and strikes the ground in 5s at an angle of 45∘ with the horizontal. Find the height of the tower and the speed with which it was projected.
View SolutionA projectile of mass 3m is projected from ground with velocity 20√2m/s at 45∘. At highest point it explodes into two pieces. One of mass 2m and the other of mass m. Both the pieces fly off horizontally in opposite directions. Mass 2m falls at a distance of 100m from point of projection. Find the distance of second mass from point of projection where it strikes the ground.
(g=10m/s2)
View SolutionA body is projected with a velocity of 30m/s to have to horizontal range of 45m. Find the angle of projection .
View Solution
