Pick the rational number from the given options
e
To determine which of the given options is a rational number, we will analyze each option step by step.
Step 1: Understand the definition of rational numbers
A rational number is defined as any number that can be expressed as the quotient or fraction pq, where p and q are integers and q≠0. Rational numbers can be either terminating or repeating decimals.
Step 2: Analyze the options
1. Option E (e): The value of e is approximately 2.71828... which is a non-terminating, non-repeating decimal. Therefore, e is an irrational number.
2. Option π (pi): The value of π is approximately 3.14159... which is also a non-terminating, non-repeating decimal. Hence, π is an irrational number.
3. Option √3: The value of √3 is approximately 1.732... which is again a non-terminating, non-repeating decimal. Thus, √3 is an irrational number.
4. Option √64: The value of √64 is exactly 8, which can be expressed as 81. Since it is a whole number, it is a rational number.
Step 3: Conclusion
From the analysis above, we find that the only rational number among the options is:
Option 4: √64 = 8
Final Answer
The correct answer is Option 4: √64.
---
A rational number is defined as any number that can be expressed as the quotient or fraction pq, where p and q are integers and q≠0. Rational numbers can be either terminating or repeating decimals.
1. Option E (e): The value of e is approximately 2.71828... which is a non-terminating, non-repeating decimal. Therefore, e is an irrational number.
2. Option π (pi): The value of π is approximately 3.14159... which is also a non-terminating, non-repeating decimal. Hence, π is an irrational number.
From the analysis above, we find that the only rational number among the options is:
The correct answer is Option 4: √64.
Similar Questions
Knowledge Check
Which is the rational number from the following ?
A√7B√8C√9D√10If one third ofa number is subtracted from three times the number, then the result is 800, find the number.
A300B400C200D600Pick the extensive property from the given options
AViscosityBRefractive indexCDensityDHeat capacity
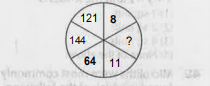
In the following question, select the missing number from the given options.
View SolutionIn the following question, select the odd number from the given options.
View SolutionGiven two different prime numbers P and Q, find the number of divisors of PQ.
View SolutionPick the odd one out from the following option.
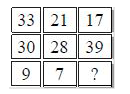
View SolutionFind the missing number from the options given below
View Solution