From a point Q, the length of the tangent to a circle is 40 cm and the distance of Q from the center is 41 cm. The radius of the circle is ______ cm
9
7
8
10
To solve the problem, we can use the Pythagorean theorem. The scenario describes a right triangle formed by the radius of the circle, the length of the tangent from point Q to the circle, and the distance from point Q to the center of the circle.
1. Identify the Given Values:
- Length of the tangent (T) = 40 cm
- Distance from point Q to the center of the circle (d) = 41 cm
- Radius of the circle (R) = ?
2. Apply the Pythagorean Theorem:
In the right triangle formed, we have:
d2=R2+T2
where:
- d is the distance from point Q to the center of the circle,
- R is the radius of the circle, and
- T is the length of the tangent.
3. Substitute the Known Values:
Substitute the values of d and T into the equation:
412=R2+402
4. Calculate the Squares:
- Calculate 412:
412=1681
- Calculate 402:
402=1600
5. Rewrite the Equation:
Now substitute these values back into the equation:
1681=R2+1600
6. Isolate R2:
To find R2, subtract 1600 from both sides:
R2=1681−1600
R2=81
7. Calculate R:
Now take the square root of both sides to find R:
R=√81
R=9 cm
Final Answer:
The radius of the circle is 9 cm.
- Length of the tangent (T) = 40 cm
- Distance from point Q to the center of the circle (d) = 41 cm
- Radius of the circle (R) = ?
In the right triangle formed, we have:
d2=R2+T2
where:
- d is the distance from point Q to the center of the circle,
- R is the radius of the circle, and
- T is the length of the tangent.
Substitute the values of d and T into the equation:
412=R2+402
- Calculate 412:
412=1681
- Calculate 402:
402=1600
Now substitute these values back into the equation:
1681=R2+1600
To find R2, subtract 1600 from both sides:
R2=1681−1600
R2=81
Now take the square root of both sides to find R:
R=√81
R=9 cm
The radius of the circle is 9 cm.
Similar Questions
From a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. The radius of the circle is
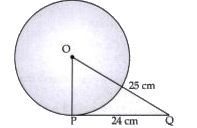
View SolutionFrom a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. The radius of circle is :
View Solution
Knowledge Check
From a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm, the radius of the circle is
A7 cmB12 cmC15 cmD24.5 cmFrom a point Q, the length of the tangent to a circle is 12 cm and the distance of Q from the centre is 13 cm. The radius of the circle is 13 cm. The radius of the circle is :
A12 cmB13 cmC5 cmDNone of theseFrom a point A, the length of the tangent to ta circle is 24 cm . And the distance of a from the centre is 25 cm . The radius of the circle is
A7 cmB12 cmC15 cmD24.5 cm
From a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. Find the radius of the circle.
View SolutionFrom a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. The radius of the circle is
(A) 7 cm (B) 12 cm (C) 15 cm (D) 24.5 cm
View SolutionFrom a point Q, the length of the tangent to a circle is 12 cm and distance of d from the centre is 13 cm. Find the radius of the circle.
View SolutionFrom a point Q , the length of the tangent to a circle is 24cm and the distance of Q from the centre is 25cm. The radius of the circle is (a) 7cm (b) 12cm (c) 15cm (d) 24.5cm
View SolutionFrom an external point Q, the length of the tangents to a circle is 5 cm and the distance of Q from the centre is 8 cm. The radius of the circle is:
View Solution
