Question

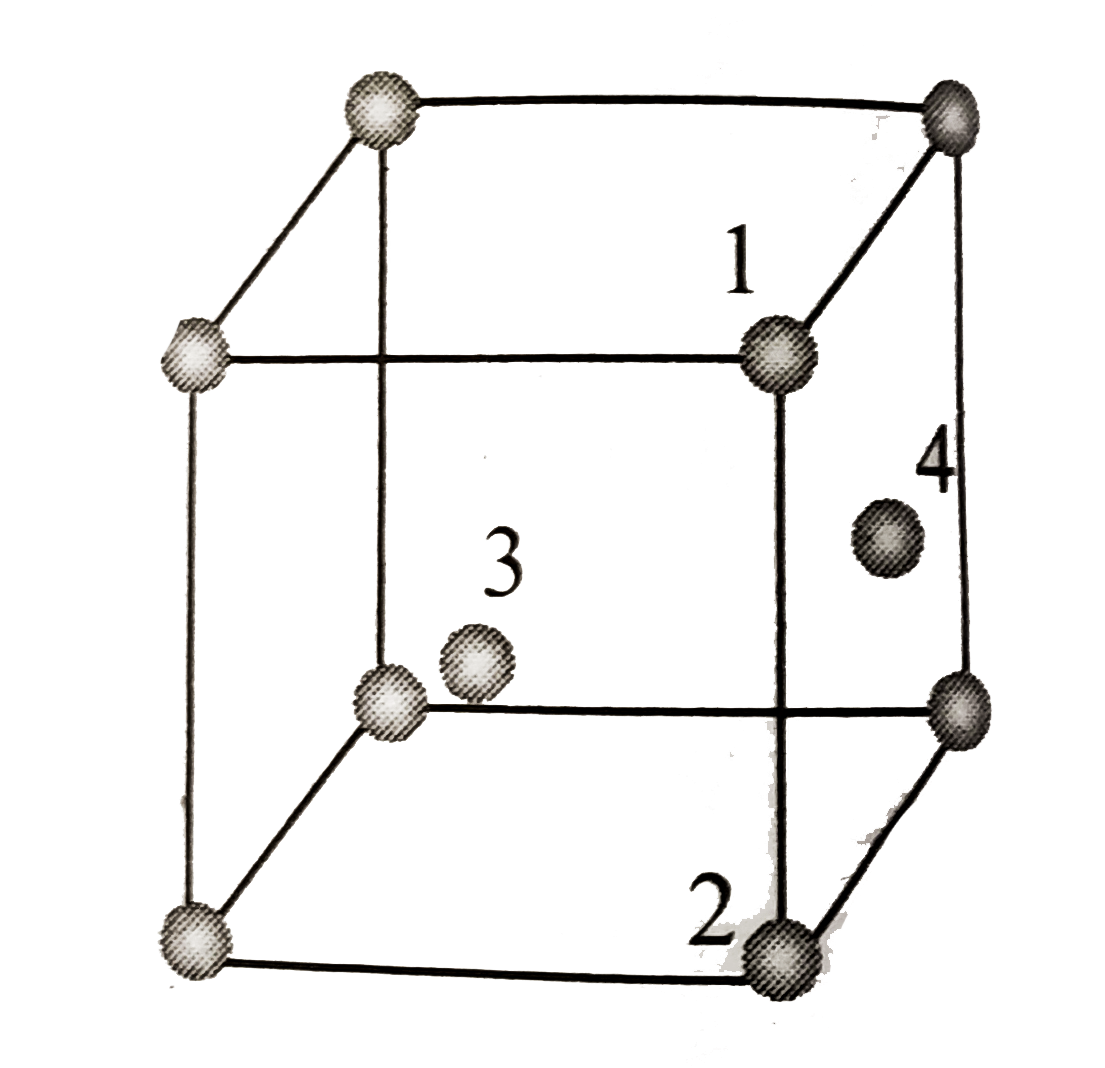
In fcc unit cell, atoms are numbered as shown below.
The atoms not touching each other are (Atom numbered ${\displaystyle 3}$ is face centre of front face)

Options

A. `3` and `4`
B. `1` and `3`
C. `1` and `2`
D. `2` and `4`

Answer

C

Explanation

In fcc structure, corner atoms do not touch each other (atom `1` and `2`), but every face centre aton touches corners. Moreover, every face center atom touches every other face centre atom in an fcc unit cell.
i. Atoms `3` and `4` are `4` are touching each other where centre-to-centre distance `= (a)/(sqrt(2))`
ii. Atoms `1` and `2` are not touching each other.
iii. Atoms `2` and `4` are touching each other where centre-to-centre distance `= (a)/(sqrt(2))`