The corner points of the feasible region of a system of linear inequations are (0, 0), (5, 0), (6,5), (6, 8), (4, 10) and (0,8). If Z = 3x - 4y, then the minimum value of Z occurs at:
(0,8)
(6,5)
(6,8)
(4, 10)
The correct Answer is:A
To find the minimum value of Z=3x−4y at the given corner points of the feasible region, we will evaluate Z at each corner point.
1. List the Corner Points: The corner points of the feasible region are:
- (0,0)
- (5,0)
- (6,5)
- (6,8)
- (4,10)
- (0,8)
2. Evaluate Z at Each Corner Point:
- For the point (0,0):
Z=3(0)−4(0)=0
- For the point (5,0):
Z=3(5)−4(0)=15
- For the point (6,5):
Z=3(6)−4(5)=18−20=−2
- For the point (6,8):
Z=3(6)−4(8)=18−32=−14
- For the point (4,10):
Z=3(4)−4(10)=12−40=−28
- For the point (0,8):
Z=3(0)−4(8)=0−32=−32
3. Compare the Values of Z:
- Z(0,0)=0
- Z(5,0)=15
- Z(6,5)=−2
- Z(6,8)=−14
- Z(4,10)=−28
- Z(0,8)=−32
4. Determine the Minimum Value:
The minimum value of Z occurs at the point (0,8) where Z=−32.
Final Answer:
The minimum value of Z occurs at the point (0,8) and the minimum value is −32.
---
- (0,0)
- (5,0)
- (6,5)
- (6,8)
- (4,10)
- (0,8)
- For the point (0,0):
Z=3(0)−4(0)=0
- For the point (5,0):
Z=3(5)−4(0)=15
- For the point (6,5):
Z=3(6)−4(5)=18−20=−2
- For the point (6,8):
Z=3(6)−4(8)=18−32=−14
- For the point (4,10):
Z=3(4)−4(10)=12−40=−28
- For the point (0,8):
Z=3(0)−4(8)=0−32=−32
- Z(0,0)=0
- Z(5,0)=15
- Z(6,5)=−2
- Z(6,8)=−14
- Z(4,10)=−28
- Z(0,8)=−32
The minimum value of Z occurs at the point (0,8) where Z=−32.
The minimum value of Z occurs at the point (0,8) and the minimum value is −32.
Topper's Solved these Questions
- SAMPLE PAPER 8Book:EDUCART PUBLICATIONChapter: SAMPLE PAPER 8Exercise:SECTION - C
Explore 9 Videos
- SAMPLE PAPER 8Book:EDUCART PUBLICATIONChapter: SAMPLE PAPER 8Exercise:SECTION - C
Explore 9 Videos
- CBSE TERM -1 SAMPLE PAPER 1Book:EDUCART PUBLICATIONChapter:CBSE TERM -1 SAMPLE PAPER 1Exercise:SECTION - C
Explore 4 Videos
Similar Questions
The corner points of the feasible region of a system of linear inequalities are (0, 0), (4,0), (3,9), (1, 5) and (0, 3). If the maximum value of objective function, Z = ax + by occurs at points (3, 9) and (1, 5), then the relation between a and b is:
View SolutionThe corner points of the feasible region of the system of linear inequations x+y≥2,x+y≤5,x−y≥0,x,y≥0 are :
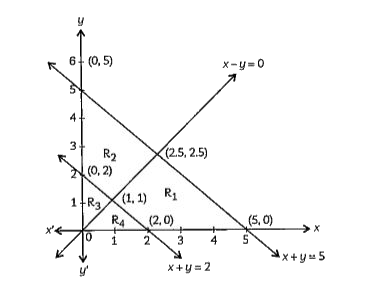
View Solution
Knowledge Check
The corner points of the feasible region determined by a system of linear inequations are (0,0),(4,0),(2,4) and (0,5). If the minimum value of Z=ax+by,a, b>0 occurs at (2,4) and (0,5) ,then :
Aa=2bB2a=bCa=bD3a=bthe corner points of the feasible region of a system of linear inequalities 3x+5y≤15,5x+2y≥10,x≥0,y≥0, are:
A(2,0),(2019,4519),(0,3)B(0,3),(2019,4519),(0,5)C(2,0),(2019,4519),(5,0)D(0,0),(2,0),(2019,4519),(0,3)Corner points of the feasible region for an LPP are (0,2),(3,0),(6,0),(6,8), and (0,5). Let F=4x+6y be the objective function. Determine the minimum value of F occurs at
Aonly (0,2)Bonly (3,0)Cthe mid point of the line segment joining the points (0,2) and (3,0)Dany point of the line segment joining the points (0,2) and (3,0)
The corner points of a feasible region determined by a system of linear inequations are (0,0),(4,0),(5,2),(2,2) and (0, 1). If the objective function is Z = x + y, then maximum of Z occurs at:
View SolutionThe corneer points of a feasible region, determined by a system of linear inequations, are (0,0),(1,0),(4,3),(2,1)and(0,1). If the objective function is Z=2x−3y, then the minimum value of Z is :
View SolutionFor the system for linear inequations x+y≤6,3x+5y≥15,x≥0,y≥0, If Z=3x+2y, then the maximum value of Z occurs at :
View SolutionThe corner points of a feasible region determined by a system of linear inequalities are (20,40),(50,100),(0,200) and (0,50). If the objective funtion Z=x+2y, then maximum of Z occurs at.
View SolutionThe corner points of the feasible region determined by the system of linear constraints are (0, 10), (5, 5) (15, 15), (0, 20). Let Z = px + qy , where p,q>0. Then, the condition on p and q so that the maximum of Z occurs at both the points (15, 15) and (0, 20), is
View Solution