IfpAandPB are the densities of the material of body and the liquid respectively then the body can float only when
pA=pB
pA<pB
pA>pB
pA=2PB
The correct Answer is:B
To determine the condition under which a body can float in a liquid, we can analyze the relationship between the densities of the body and the liquid. Here’s a step-by-step solution to the problem:
1. Understanding the Concept of Floatation:
- A body will float in a liquid if the buoyant force acting on it is equal to its weight. The buoyant force is determined by the density of the liquid and the volume of the liquid displaced by the body.
2. Defining Densities:
- Let PA be the density of the body (solid).
- Let PB be the density of the liquid.
3. Applying Archimedes' Principle:
- According to Archimedes' principle, the buoyant force Fb acting on the body is equal to the weight of the liquid displaced by the body. This can be expressed as:
Fb=PB⋅V⋅g
- Where V is the volume of the body submerged in the liquid and g is the acceleration due to gravity.
4. Setting Up the Condition for Floatation:
- For the body to float, the buoyant force must be equal to the weight of the body:
Fb=PA⋅V⋅g
- Therefore, we have:
PB⋅V⋅g=PA⋅V⋅g
5. Simplifying the Equation:
- Since g and V are common on both sides, we can simplify the equation to:
PB=PA
- This indicates that if the density of the liquid is equal to the density of the body, the body will float.
6. Considering Other Conditions:
- However, if the density of the liquid is greater than the density of the body, the body will also float. Thus, we can conclude that:
PB≥PA
Conclusion:
A body can float in a liquid if the density of the liquid PB is greater than or equal to the density of the body PA. Therefore, the condition for floatation is:
PB≥PA
- A body will float in a liquid if the buoyant force acting on it is equal to its weight. The buoyant force is determined by the density of the liquid and the volume of the liquid displaced by the body.
- Let PA be the density of the body (solid).
- Let PB be the density of the liquid.
- According to Archimedes' principle, the buoyant force Fb acting on the body is equal to the weight of the liquid displaced by the body. This can be expressed as:
Fb=PB⋅V⋅g
- Where V is the volume of the body submerged in the liquid and g is the acceleration due to gravity.
- For the body to float, the buoyant force must be equal to the weight of the body:
Fb=PA⋅V⋅g
- Therefore, we have:
PB⋅V⋅g=PA⋅V⋅g
- Since g and V are common on both sides, we can simplify the equation to:
PB=PA
- This indicates that if the density of the liquid is equal to the density of the body, the body will float.
- However, if the density of the liquid is greater than the density of the body, the body will also float. Thus, we can conclude that:
PB≥PA
A body can float in a liquid if the density of the liquid PB is greater than or equal to the density of the body PA. Therefore, the condition for floatation is:
PB≥PA
Similar Questions
What is the velocity v of a metallic ball of radius r falling in a tank of liquid at the instant when its acceleration is one-half that of a freely falling body ? (The densities of metal and of liquid are r and s respectively, and the viscosity of the liquid is η).
View SolutionThe buoyant force exerted by a liquid depends upon the nature of the liquid in which the object is immersed . (i) The body would float if the density of its material is ____ to or___ than the density of the liquid. (ii) The body would sink if the density of the material of the body is ___ than the density of the liquid.
View Solution
Knowledge Check
A stream-lined body falls through air from a height h on the surface of a liquid . Let d and D denote the densities of the materials of the body and the liquid respectively, if D>d, then the time after which the body will be intantaneously at rest, is:
A√2hgB√2hgDdC√2hgdDD√2hg(dD−d)In case of a hollow body, if ρB and ρS represent the densities of the body and the substance (of which it is made) respectively, then:
AρB=ρSBρB<ρSCρB>ρSDnone of theseA body is floating in a liquid. The upthrust on the body is
Aequal to weight of liquid displacedBzeroCless than the weight of liquid displacedDweight of body weight of liquid displaced
two cylinders A and B are of the same dimensions but of different materials. A alone flots in a liquid with half of its length immersed. When B is placed over A , the combination just floats in the same liquid . Compare the densities of the two cylinders A and B.
View SolutionA body is floating in a liquid. The upthurst on the body is
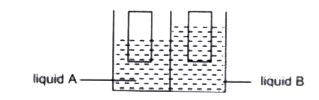
View SolutionTwo objects similar in all respects are floating in two liquids 'A' and 'B' as shown in the figure. If dAis the density of liquid A, dg is the density of liquid B, and dB is the density of the object, which among the following is the correct relation between the densities of the objects and the liquid?
View SolutionA body floats in a liquid if the buoyant force is
View SolutionA body floats in a liquid if the buoyant force is
View Solution
