A ball filled with air has a volume of 500cm3 calculate the minimum force applied by a child to put it completely inside the water (take g=10ms2)
To solve the problem of calculating the minimum force required by a child to completely submerge a ball filled with air that has a volume of 500cm3 in water, we can follow these steps:
Step 1: Understand the Concept of Buoyant Force
The buoyant force is the upward force exerted by a fluid on an object submerged in it. According to Archimedes' principle, the buoyant force is equal to the weight of the fluid displaced by the object.
Step 2: Calculate the Volume of the Ball in Cubic Meters
The volume of the ball is given as 500cm3. We need to convert this volume into cubic meters for standard SI units:
500cm3=500×10−6m3=5×10−4m3
Step 3: Calculate the Weight of the Water Displaced
The weight of the water displaced can be calculated using the formula:
Weight of water displaced=Volume×Density of water×g
Where:
- The density of water (ρ) is approximately 1000kg/m3.
- The acceleration due to gravity (g) is given as 10m/s2.
Substituting the values:
Weight of water displaced=(5×10−4m3)×(1000kg/m3)×(10m/s2)
=5×10−4×1000×10
=5N
Step 4: Determine the Minimum Force Required
To completely submerge the ball, the child must apply a force that at least equals the buoyant force (which is equal to the weight of the water displaced). Therefore, the minimum force F required is:
F=5N
Final Answer
The minimum force applied by the child to put the ball completely inside the water is 5N.
---
The buoyant force is the upward force exerted by a fluid on an object submerged in it. According to Archimedes' principle, the buoyant force is equal to the weight of the fluid displaced by the object.
The volume of the ball is given as 500cm3. We need to convert this volume into cubic meters for standard SI units:
500cm3=500×10−6m3=5×10−4m3
The weight of the water displaced can be calculated using the formula:
Weight of water displaced=Volume×Density of water×g
Where:
- The density of water (ρ) is approximately 1000kg/m3.
- The acceleration due to gravity (g) is given as 10m/s2.
Weight of water displaced=(5×10−4m3)×(1000kg/m3)×(10m/s2)
=5×10−4×1000×10
=5N
To completely submerge the ball, the child must apply a force that at least equals the buoyant force (which is equal to the weight of the water displaced). Therefore, the minimum force F required is:
F=5N
The minimum force applied by the child to put the ball completely inside the water is 5N.
Similar Questions
A ping-pong ball has a volume V and density (1/10) th of water. What force would be required to hold it completely submerged under water?
View SolutionA block of mass 5kg is falling downwards in air with an acceleration of 2ms^(-2) air resistance is present. The force applied by block on the earth will be (g=10m/s^(2))
View Solution
Knowledge Check
A sealed can of mass 700g has a volume of 500cm3 what is the density of this can?(density of water is 1gcm^-3)
A1.4gcm−3B1.0gcm−3C2.1gcm−3D2.5gcm−3A cylindrical tank has a hole of 2cm2 at its bottom if the water is allowed to flow into tank from a tube above it at the rate of 100cm3/s then find the maximum height upto which water can rise in the tank (take =g=10ms−2)
A2.5×10−2mB1.25×10−2mC5.5×10−2mD3.5×10−2mAssuming that the density of water to be 1g/cm3, calculate the volume occupied by one molecule of water.
A2.989×10−23mLB6.023×1023 cm3C0.288×10−3D1.66×109−2)
An object of mass 60g has a volume of 10cm3. Calculate the density of the object. If the density of water be g/cm3, state whether the object will float or sink in water.
View SolutionA block of mass 10 kg is kept on a rough inclined plane as shown in the figure. A force of 3 N is applied on the block. The coefficient of static friction between the plane and the block is 0.6 What should be the minimum value of force P, such that the block doesnot move downward? (take g=10ms−2)
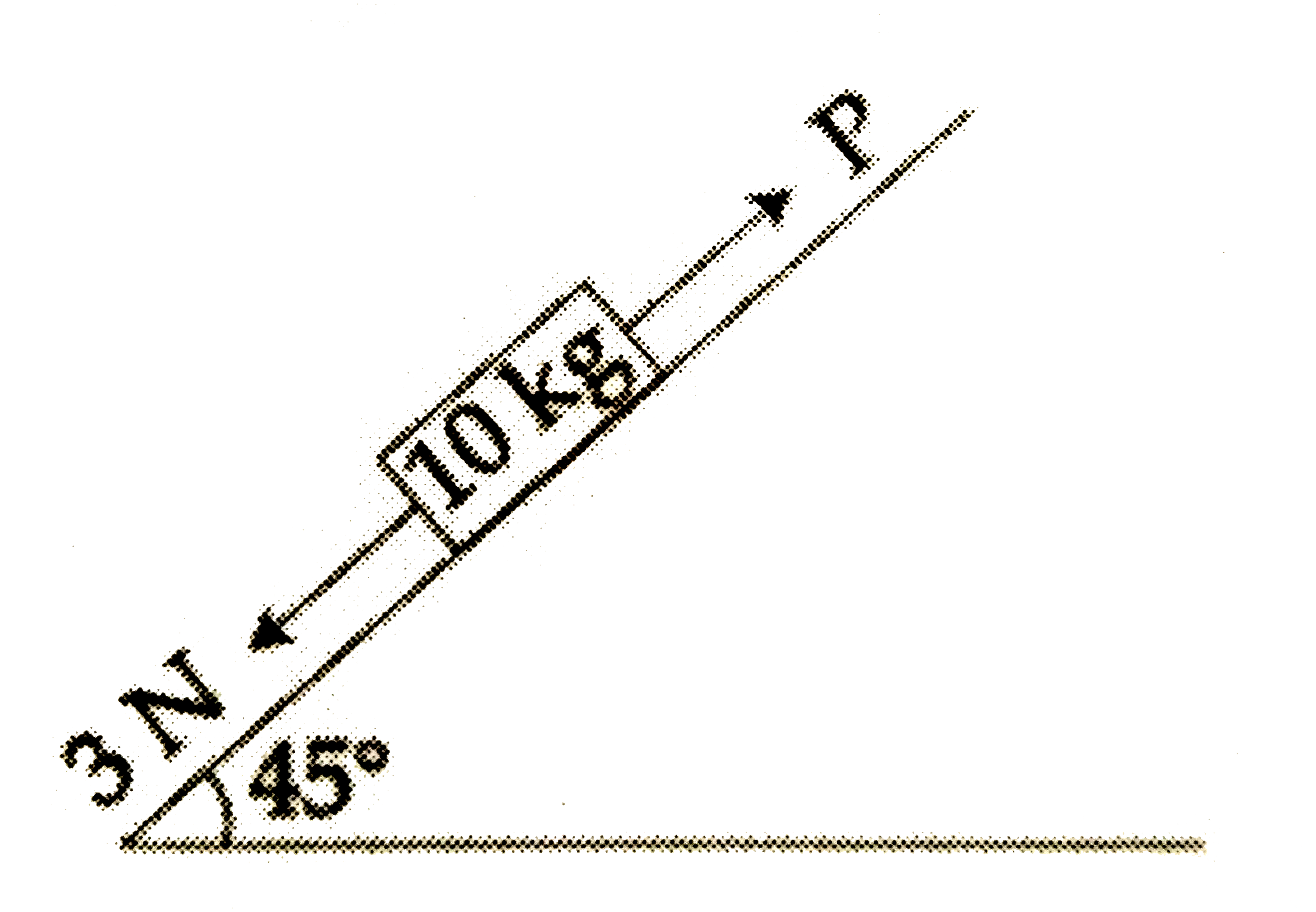
View SolutionA woman draws water from a well using a fixed pulley. The mass of bucket and water together is 6.0 kg the force applied by the woman is 70N. Calculate the mechanical advantage. (g = 10 ms−2)
View SolutionAn object of mass 50 g has a volume of 20cm3
Calculate the density of the object. If the density of water 1/gcm2, state whether the object will float or sink in water.
View SolutionA beaker of circular ceross sectionof radius 4 cm is filled with mercury up to a height of 10 cm. Find the force exerted by the mercury on the bottom of the beaker. The atmospheric pressure =105Nm−2. Density of mercury =13600kgm−3.Takeg10ms−2
View Solution
