For any circuit, number of independent equations containing emf's, resistance and current equals:
no. of junction
no. of branches
no. of branches + 1
no, of junction + number of branches
The correct Answer is:B
To determine the number of independent equations containing EMFs, resistance, and current in any circuit, we can use the following steps:
Step 1: Identify the Components of the Circuit
- Junctions: Points where two or more conductors meet.
- Branches: Paths in the circuit that can carry current.
Step 2: Apply Kirchhoff's Laws
- Kirchhoff's Current Law (KCL) states that the total current entering a junction must equal the total current leaving the junction.
- Kirchhoff's Voltage Law (KVL) states that the sum of the electromotive forces (emfs) and the potential drops (voltage across resistors) in any closed loop of a circuit must equal zero.
Step 3: Count the Junctions and Branches
- Let J be the number of junctions in the circuit.
- Let B be the number of branches in the circuit.
Step 4: Determine the Number of Independent Equations
- The number of independent equations can be calculated using the formula:
Number of independent equations=J+(B−1)
This is derived from the fact that you can write J equations from KCL and B−1 equations from KVL (since one loop can be derived from the others).
Conclusion
Thus, the number of independent equations containing EMFs, resistance, and current in any circuit equals the number of junctions plus the number of branches minus one.
- Junctions: Points where two or more conductors meet.
- Branches: Paths in the circuit that can carry current.
- Kirchhoff's Current Law (KCL) states that the total current entering a junction must equal the total current leaving the junction.
- Kirchhoff's Voltage Law (KVL) states that the sum of the electromotive forces (emfs) and the potential drops (voltage across resistors) in any closed loop of a circuit must equal zero.
- Let J be the number of junctions in the circuit.
- Let B be the number of branches in the circuit.
- The number of independent equations can be calculated using the formula:
Number of independent equations=J+(B−1)
This is derived from the fact that you can write J equations from KCL and B−1 equations from KVL (since one loop can be derived from the others).
Thus, the number of independent equations containing EMFs, resistance, and current in any circuit equals the number of junctions plus the number of branches minus one.
Topper's Solved these Questions
- SAMPLE PAPER 2BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 2EXERCISE - SECTION-B
24 Videos
- SAMPLE PAPER 2BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 2EXERCISE - SECTION-C
6 Videos
- SAMPLE PAPER 04 (SOLVED)BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 04 (SOLVED)EXERCISE - SECTION-C
6 Videos
- SAMPLE PAPER 3BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 3EXERCISE - SECNTION -C
6 Videos
Similar Questions
A battery of unknown emf and internal resistance is given to a student for the experiment. When student connects the battery to an external resistance of 10Ω then 1A current flows through the circuit and when the same is connected to 15Ω resistance then 0.75 A current flows through the circuit . Calculate the emf and internal resistance of the given battery.
View Solutionn' cells , each of emf 'e' and internal resistance 'r' are connected in a closed circuit so that the positive terminal of a cell is joined to the negative terminal of the next, as shown in figure. Any two points of the circuit are connected by an external resistance R. Find the current in R.
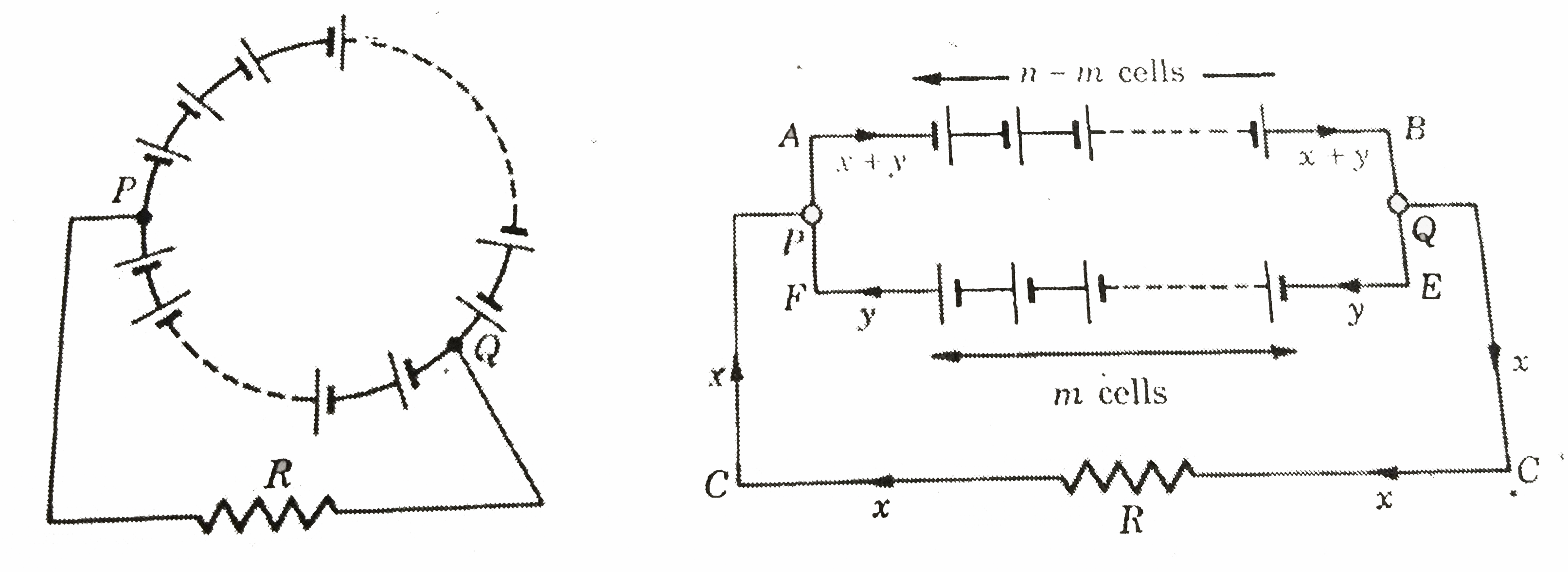
View Solution
Knowledge Check
In a simple A.C. circuit containing resistance, the current
Alags behind the e.m.f. by π/2Bis in phase with applied e.m.f.Cleads the applied e.m.f. by π/2Dnone of theseThe effective resistance of a circuit containing resistances in parallel is
Aequal to the sum of the individual resistancesBsmaller than any of the individual resistancesCgreater than any of the individual resistancesDsometimes greater and sometimes smaller than the individual resistancesn equal cell having emf E and internal resistance r, are connected in circuit of a resistance R. Same current flows in circuit either they connected in series or parallel, if:
AR=nrBR=rnCR=n2rDR=r
An alternating current of frequency 'f' is flowing in a circuit containing a resistance R and a choke L in series. The impedence of this circuit is
View SolutionAn e.m.f. of 15 volt is applied in a circuit containing 5 henry inductance and 10 ohm resistance. The ratio of the current at time t=∞ and at t=1 second is
View SolutionIn an LR-circuit, the inductive reactance is equal to the resistance R of the circuit. An e.m.f E=E0cos(ωt) applied to the circuit. The power consumed in the circuit is
View SolutionAn alternating current by frequency v is flowing in a circuit containing a resistance R and inductance L in series. The impedance of this circuit is
View SolutionIn a purely resistive ac circuit, the current
View Solution
