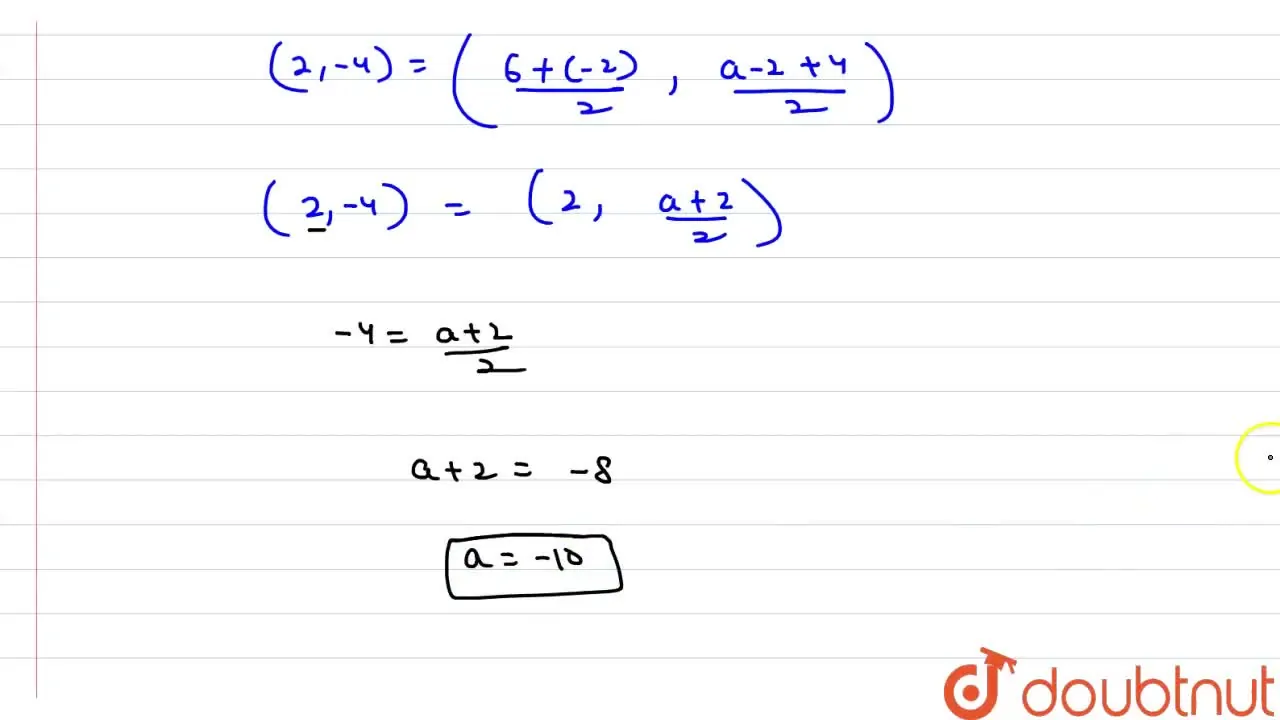
What is the value of a if the mid-point of the line segment joining the points P(6,a−2) and Q(−2,4) is (2,−4) ?
−10
10
0
7
Step by step video & image solution for What is the value of a if the mid-point of the line segment joining the points P(6,a-2) and Q(-2,4) is (2,-4) ? by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
- BOOK - EDUCART PUBLICATIONCHAPTER - CBSE TERM-1 SAMPLE PAPER 2EXERCISE - Section -B17videos
CBSE TERM-1 SAMPLE PAPER 2
- BOOK - EDUCART PUBLICATIONCHAPTER - CBSE TERM-1 SAMPLE PAPER 2EXERCISE - Section -C7videos
CBSE TERM-1 SAMPLE PAPER 2
- BOOK - EDUCART PUBLICATIONCHAPTER - CBSE PAPER (12 MARCH 2020)EXERCISE - Set III (Section - D)2videos
CBSE PAPER (12 MARCH 2020)
- BOOK - EDUCART PUBLICATIONCHAPTER - CIRCLESEXERCISE - LONG ANSWER Type Questions13videos
CIRCLES
Similar Questions
Find the mid-point of the line segment joining the points (2,-6) and (6,-4) .
View SolutionThe mid-point of the line segment joining the points (- 2, 4) and (6, 10) is
View SolutionFind the mid point of the line segment joining the points (−2,7)and(4,−3).
View SolutionThe co-ordinate of the mid-point of the line segment joining the points P(4,−6)andQ(−2,4) is…..
View SolutionFind the mid-point of the segment joining the points (-2,4) and (6,10)
View SolutionWrite coordinates of the mid point P of the line segment joining the point A(-2,-1) and B(4,5)
View SolutionIn which quadrant does the mid-point of the line segment joining the points (-1, 2) and (3, 4) Lies?
View SolutionFind the distance of the point (1, 2) from the mid-point of the line segment joining the points (6, 8) and (2, 4) .
View SolutionIn which quadrant does the mid-point of the Line segment joining the points (-1, 2) and (3, 4) lies?
View SolutionIf P(2,p) is the mid-point of the line segment joining the points A(6, -5) and B(-2, 11), find the value of p
View Solution