For the following frequency distribution, determine the mode and the median:
Class100−120120−140140−160160−180180−200Frequency12148610
The correct Answer is:125,138.57
To solve the problem, we need to determine the mode and the median for the given frequency distribution.
Given Data:
- Class Intervals: 100-120, 120-140, 140-160, 160-180, 180-200
- Frequencies: 12, 14, 8, 6, 10
Step 1: Calculate Cumulative Frequency
We first calculate the cumulative frequency for the given frequency distribution.
- For the class 100-120: Cumulative Frequency = 12
- For the class 120-140: Cumulative Frequency = 12 + 14 = 26
- For the class 140-160: Cumulative Frequency = 26 + 8 = 34
- For the class 160-180: Cumulative Frequency = 34 + 6 = 40
- For the class 180-200: Cumulative Frequency = 40 + 10 = 50
Cumulative Frequency Table:
ClassFrequencyCuμlativeFrequency100−1201212120−1401426140−160834160−180640180−2001050
Step 2: Determine the Mode
The mode is the value that appears most frequently in a data set. In this case, we look for the class with the highest frequency.
- The highest frequency is 14, which corresponds to the class interval 120-140.
Mode Formula:
\( \text{Mode} = L + \frac{f_1 - f_0}{(2f_1 - f_0 - f_2)} \times h \)
Where:
- \( L \) = lower limit of the modal class = 120
- \( f_1 \) = frequency of the modal class = 14
- \( f_0 \) = frequency of the class before the modal class = 12
- \( f_2 \) = frequency of the class after the modal class = 8
- \( h \) = width of the class interval = 20
Calculating the Mode:
\( \text{Mode} = 120 + \frac{14 - 12}{(2 \times 14 - 12 - 8)} \times 20 \)
\( = 120 + \frac{2}{(28 - 20)} \times 20 \)
\( = 120 + \frac{2}{8} \times 20 \)
\( = 120 + \frac{1}{4} \times 20 \)
\( = 120 + 5 \)
\( = 125 \)
Step 3: Determine the Median
To find the median, we need to locate the median class. The median class is the class where the cumulative frequency is greater than or equal to \( \frac{n}{2} \), where \( n \) is the total frequency.
- Total frequency \( n = 50 \)
- \( \frac{n}{2} = 25 \)
The cumulative frequency just greater than 25 is 26, which corresponds to the class interval 120-140.
Median Formula:
\( \text{Median} = L + \frac{\frac{n}{2} - CF}{f} \times h \)
Where:
- \( L \) = lower limit of the median class = 120
- \( CF \) = cumulative frequency of the class before the median class = 12
- \( f \) = frequency of the median class = 14
- \( h \) = width of the class interval = 20
Calculating the Median:
\( \text{Median} = 120 + \frac{25 - 12}{14} \times 20 \)
\( = 120 + \frac{13}{14} \times 20 \)
\( = 120 + \frac{260}{14} \)
\( = 120 + 18.57 \)
\( \approx 138.57 \)
Final Results:
- Mode: 125
- Median: 138.57
- Class Intervals: 100-120, 120-140, 140-160, 160-180, 180-200
- Frequencies: 12, 14, 8, 6, 10
We first calculate the cumulative frequency for the given frequency distribution.
- For the class 120-140: Cumulative Frequency = 12 + 14 = 26
- For the class 140-160: Cumulative Frequency = 26 + 8 = 34
- For the class 160-180: Cumulative Frequency = 34 + 6 = 40
- For the class 180-200: Cumulative Frequency = 40 + 10 = 50
ClassFrequencyCuμlativeFrequency100−1201212120−1401426140−160834160−180640180−2001050
The mode is the value that appears most frequently in a data set. In this case, we look for the class with the highest frequency.
\( \text{Mode} = L + \frac{f_1 - f_0}{(2f_1 - f_0 - f_2)} \times h \)
Where:
- \( L \) = lower limit of the modal class = 120
- \( f_1 \) = frequency of the modal class = 14
- \( f_0 \) = frequency of the class before the modal class = 12
- \( f_2 \) = frequency of the class after the modal class = 8
- \( h \) = width of the class interval = 20
\( \text{Mode} = 120 + \frac{14 - 12}{(2 \times 14 - 12 - 8)} \times 20 \)
\( = 120 + \frac{2}{(28 - 20)} \times 20 \)
\( = 120 + \frac{2}{8} \times 20 \)
\( = 120 + \frac{1}{4} \times 20 \)
\( = 120 + 5 \)
\( = 125 \)
To find the median, we need to locate the median class. The median class is the class where the cumulative frequency is greater than or equal to \( \frac{n}{2} \), where \( n \) is the total frequency.
- \( \frac{n}{2} = 25 \)
\( \text{Median} = L + \frac{\frac{n}{2} - CF}{f} \times h \)
Where:
- \( L \) = lower limit of the median class = 120
- \( CF \) = cumulative frequency of the class before the median class = 12
- \( f \) = frequency of the median class = 14
- \( h \) = width of the class interval = 20
\( \text{Median} = 120 + \frac{25 - 12}{14} \times 20 \)
\( = 120 + \frac{13}{14} \times 20 \)
\( = 120 + \frac{260}{14} \)
\( = 120 + 18.57 \)
\( \approx 138.57 \)
- Mode: 125
- Median: 138.57
Similar Questions
Knowledge Check
Consider the following frequency distribution

The upper limit of the median class inA17B17.5C18D18.5In the following frequency distribution, find the median class.

What is the value of median height ?A145.67B157.67C155.83D159.67
Consider the following frequency distribution
What is the median class?
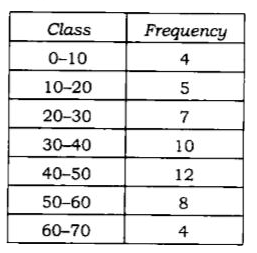
View SolutionConsider the following frequency distribution:
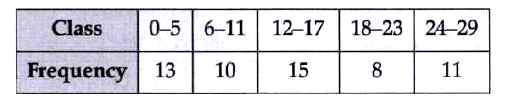
the upper limit of the median class is
View SolutionCalculate the arithmetic mean of the following frequency distribution by Direct Method:
Class Interval10−2020−4040−7070−120120−200Frequency 4 10 26 8 2
View SolutionCalculate the arithmetic mean of the following frequency distribution by Direct Method:
Class Interval10−2020−4040−7070−120120−200Frequency 4 10 26 8 2
View SolutionFind the mode of the following frequency distribution:
Class100−110110−120120−130130−140140−150150−160160−170Frequency4620323382
View SolutionIn a frequency distribution mode is 7.88 , mean is 8.32 .find the median
View Solution

