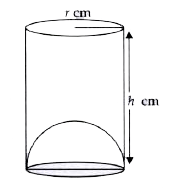
The capacity of a cylindrical vessesl with a hemispherical portion raised upwards at the bottom as shown in the given figure is πr23[3h−2r].
![The capacity of a cylindrical vessesl with a hemispherical portion raised upwards at the bottom as shown in the given figure is (pi r^(2))/(3) [3h - 2r].](https://d10lpgp6xz60nq.cloudfront.net/physics_images/AGP_EDG_MAT_X_STN_QB_C12_E01_025_Q01.png)
The correct Answer is:True
Step by step video, text & image solution for The capacity of a cylindrical vessesl with a hemispherical portion raised upwards at the bottom as shown in the given figure is (pi r^(2))/(3) [3h - 2r]. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SURFACE AREAS AND VOLUMESBOOK - EDUCART PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - OBJECTIVE TYPE QUESTIONS (VERY SHORT QUESTIONS)
6 Videos
- SURFACE AREAS AND VOLUMESBOOK - EDUCART PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - SHORT ANSWER (SA-I) TYPE QUESTIONS
11 Videos
- SURFACE AREAS AND VOLUMESBOOK - EDUCART PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - OBJECTIVE TYPE QUESTIONS (FILL IN THE BLANKS)
7 Videos
- STATISTICS AND PROBABILITYBOOK - EDUCART PUBLICATIONCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ANSWER TYPE QUESTIONS
19 Videos
- TRIANGLESBOOK - EDUCART PUBLICATIONCHAPTER - TRIANGLESEXERCISE - LONG ANSWER TYPE QUESTIONS
10 Videos
Similar Questions
Knowledge Check
Magnetic field in cylindrical region of radius R in inward direction is as shown in figure.
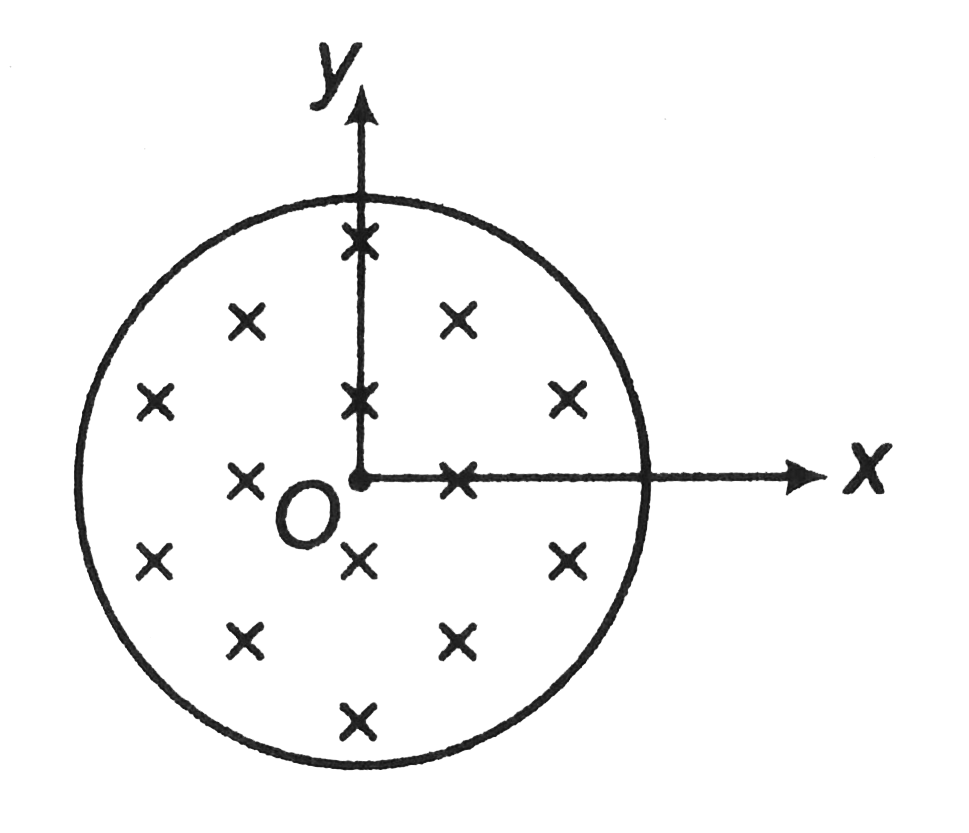 Aan electron will expereince no force kept is (2R,0,0) if magnetic field increases with timeBin the above situation, electron will experience the force in negative y-axisCif a proton is kept at (0,R2,0) and magnetic field is decreasing then it will experience the force in positive x-directionDif a proton is kept at (−R,0,0) and magnetic field is increasing then it will experience force in negative y-axis
Aan electron will expereince no force kept is (2R,0,0) if magnetic field increases with timeBin the above situation, electron will experience the force in negative y-axisCif a proton is kept at (0,R2,0) and magnetic field is decreasing then it will experience the force in positive x-directionDif a proton is kept at (−R,0,0) and magnetic field is increasing then it will experience force in negative y-axisFrom a solid sphere of mass M and radius R, a spherical portion of radius R/2 is removed, as shown in the figure Taking gravitational potential V=0atr=∞, the potential at (G = gravitational constatn)
 A−2GM3RB−2GMRC−GM2RD−GMR
A−2GM3RB−2GMRC−GM2RD−GMRIn the circuit shown in the given figure, the resistances R1andR2 are respectively
 A14Ωand40ΩB40Ωand44ΩC40Ωand30ΩD14Ωand30Ω
A14Ωand40ΩB40Ωand44ΩC40Ωand30ΩD14Ωand30Ω
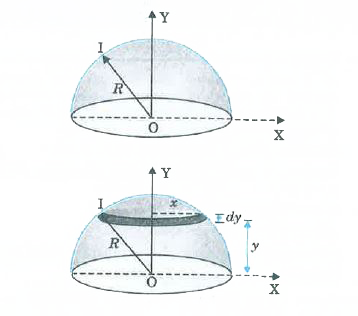
Locate the position of the centre of mass of a hemisphere of radius R as shown in the following figure:
View SolutionA water tank open at the top is hemispherical at the bottom and cylindrical above it. If radius of the hemisphere is 12 m and the total capacity of the tank is 3312π m3 , then the ratio of the surface areas of the hemispherical and the cylindrical portions is (a) 1 : 1 (b) 3 : 5 (c) 4 : 5 (d) 6 : 5
View SolutionA juice seller was serving his costumers using glasses as shown in the figure. The inner diameter of the cylindrical glass was 5 cm but bottom of the glass had a hemispherical raised portion which reduced the capacity of the glass. If the height of a glass was 10 cm, find the apparent and actual capacity of the glass. (Use π = 3.14)

View SolutionIf r and R and the respective radii of the smaller and the bigger semi-circles then the area of the shaded portion in the given figure is: (FIGURE) πr2 sq.units (b) πR2−πr2 sq.units (c) πR2+πr2 sq.units (d) πR2 sq.units
View SolutionWater is flowing out at the rate of 6m3min from a reservoir shaped like a hemispherical bowl of radius R=13m. The volume of water in the hemispherical bowl is given by V=π3.y2(3R−y)when the water is y meter deep. Find
View Solution