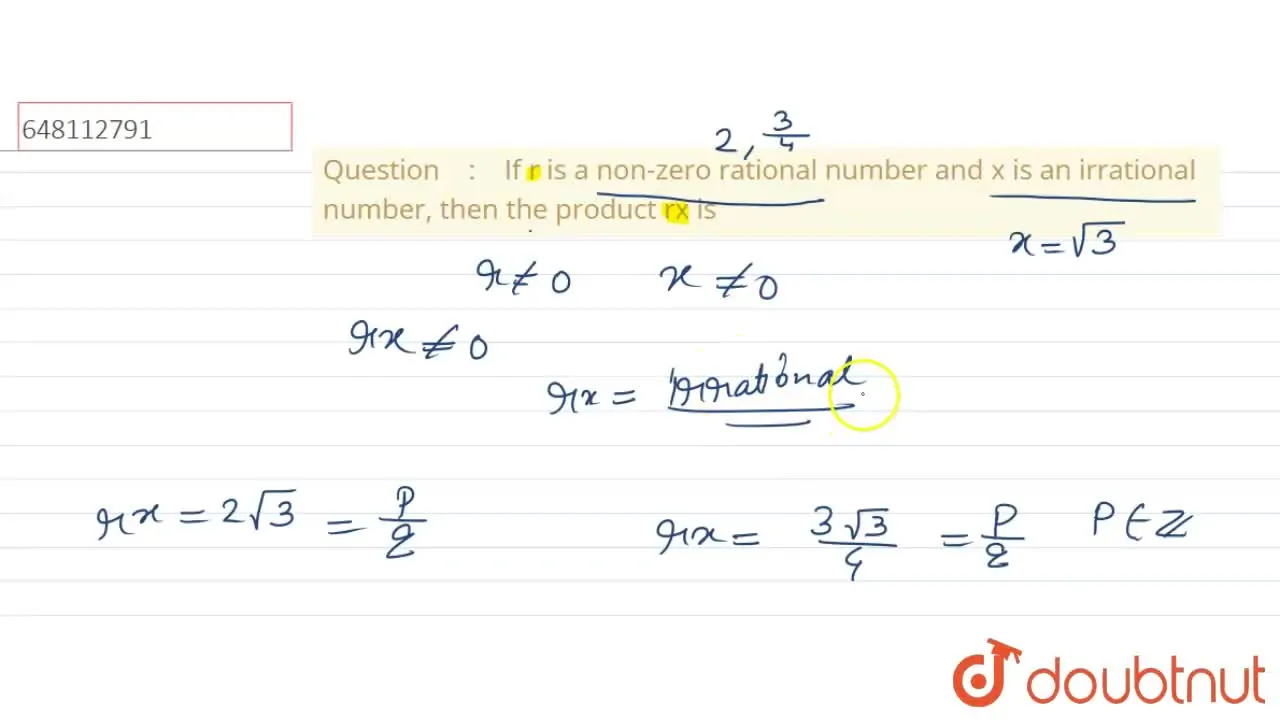
If r is a non-zero rational number and x is an irrational number, then the product rx is
a rational number
an integer
an irrational number
None of these
The correct Answer is:C
Step by step video, text & image solution for If r is a non-zero rational number and x is an irrational number, then the product rx is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ARIHANT PUBLICATION BIHARCHAPTER - NUMBER SYSTEMEXERCISE - EXAM BOOSTER (FOR CRACKING EXAM)25videos
NUMBER SYSTEM
- BOOK - ARIHANT PUBLICATION BIHARCHAPTER - MODEL SOLVED PAPER 2020EXERCISE - Section III Mathematics30videos
MODEL SOLVED PAPER 2020
- BOOK - ARIHANT PUBLICATION BIHARCHAPTER - QUADRATIC EQUATIONS EXERCISE - EXAM BOOSTER (FOR CRACKING EXAM)25videos
QUADRATIC EQUATIONS
Similar Questions
If x is a rational number and y is an irrational number, then both x + y and xy are necessarily rational both x + y and xy are necessarily irrational xy is necessarily irrational, but x + y can be either rational or irrational x + y is necessarily irrational, but xy can be either rational or irrational
View SolutionIf x be a rational number and y be an irrational number, then :
View SolutionThe product of a rational number and an irrational number is
View SolutionWrite a rational number and an irrational number between 1 and 2.
View SolutionIf Q is the set of rational numbers and P is the set of irrational numbers, then
View SolutionThe product of a non zero rational and an irrational number is
View SolutionThe square of an irrational number is always a rational number
View SolutionAre the following pairs of statements negations of each other:
(i) The number x is not a rational number. The number x is not an irrational number.
(ii) The number x is a rational number. The number x is an irrational number.View SolutionInsert on rational and an irrational number between 3 and 4.
View SolutionReciprocal of a non - zero rational number
View Solution