If it is possible to make a three-digit number which is the perfect square of a two-digit number from the second, the fourth and the eighth digits of the numbers 31792056, using each digit only once, what is the two-digits number ? If the perfect square cannot be formed, your answer is 'N' and if more than one such number can be formed, your answer is 'X'
1) 14
2) 16
3) 12
4) X
5) N
14
16
12
X
The correct Answer is:D
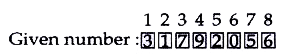
The second, fourth and eight digits de bed ste = 1 , 9 and 6 respectively.
The perfect squares that can be formed using
1,9,6 = 169, 196 and 961 i.e (13)2,(14)2and(31)2
Therefore, our answer is .X..
Topper's Solved these Questions
- BOOK - LUCENT PUBLICATIONCHAPTER - NUMBER PROBLEMS EXERCISE - Solved Examples (Type IV : Arrangement of Set of Numbers)2videos
NUMBER PROBLEMS
- BOOK - LUCENT PUBLICATIONCHAPTER - NUMBER PROBLEMS EXERCISE - Solved Examples (Type - V Resultant/Outcome of Row Based on Rules:)5videos
NUMBER PROBLEMS
- BOOK - LUCENT PUBLICATIONCHAPTER - NUMBER PROBLEMS EXERCISE - Solved Examples (Type II : Arrangement of Digits/Pair of Dogits/Number)4videos
NUMBER PROBLEMS
- BOOK - LUCENT PUBLICATIONCHAPTER - MULTI TIER SEQUENTIAL SERIES EXERCISE - Exercise12videos
MULTI TIER SEQUENTIAL SERIES
- BOOK - LUCENT PUBLICATIONCHAPTER - PASSAGE AND CONCLUSION EXERCISE - Exercise - 345videos
PASSAGE AND CONCLUSION
Similar Questions
If it is possible to form only one three digit number which is a perfect square of a two digit number from the second, the sixth and the seventh digits of the number 76483912 using each digit only once. The second digit of that number is your answer. If no such number can be formed, your answer is '0' and if more than one such number can be formed, your answer is '5'.
1) 5
2) 0
3) 1
4) 6
5) 9View SolutionIf it is possible to make a three-digit number from the third, the fifth and the sixth digits of the number 30651972, using each digit only once which is the square of an odd number, the last digit of that three-digit number is your answer. If no such number can be formed, your answer is 4 and if more than one such number can be formed, your answer is 8.'
1) 1
2) 9
3) 6
4) 4
5) 8View Solution
Knowledge Check
- Question 1 - Select One
The least 4 digit number which is a perfect square is
A1024B1016C1036D1044 - Question 2 - Select One
The smallest 4-digit number, which is a perfect square, is
A1000B1016C1024D1025 - Question 3 - Select One
If it is possible to form a number which is perfect square of a two digit add number using the second, the fourth and the seventh digits of the number 739142658 using each only once, which of following is the second digit of that two digit odd number?
A3B4C5DNone of these
The greatest 4 digit number which is a perfect square, is
View SolutionThe greatest 4 digit number which is a perfect square, is
View SolutionThe greatest 3 digit number which is a perfect square is
View SolutionThe greatest 3 digit number which is a perfect square is
View SolutionThe least six digit number which is a perfect square is
View Solution
