Two conductors A and B resistance 5ωand10ω respectively are first joined in parallel and then in series. In each case the voltage applied is 20 V.
In which arrangment will the current through A and b be the same ?
Series arrangment
Parallel arrangement
Both of the above
None of the above
The correct Answer is:A
To determine in which arrangement the current through conductors A and B will be the same, we need to analyze both the parallel and series configurations.
Step 1: Analyze the Parallel Arrangement
1. Resistance Values:
- Resistance of conductor A, RA=5Ω
- Resistance of conductor B, RB=10Ω
2. Voltage Applied:
- V=20V
3. Current Calculation:
- In a parallel circuit, the voltage across each resistor is the same. Therefore, the current through each resistor can be calculated using Ohm's Law:
IA=VRA=20V5Ω=4A
IB=VRB=20V10Ω=2A
4. Conclusion for Parallel Arrangement:
- The currents through A and B are different: IA=4A and IB=2A.
Step 2: Analyze the Series Arrangement
1. Total Resistance in Series:
- The total resistance Rtotal in series is the sum of the individual resistances:
Rtotal=RA+RB=5Ω+10Ω=15Ω
2. Current Calculation:
- In a series circuit, the same current flows through all components. The total current I can be calculated using Ohm's Law:
I=VRtotal=20V15Ω=43A≈1.33A
3. Conclusion for Series Arrangement:
- The current through both A and B is the same in the series arrangement, and it is approximately 1.33A.
Final Conclusion
- The current through conductors A and B will be the same in the series arrangement.
---
1. Resistance Values:
- Resistance of conductor A, RA=5Ω
- Resistance of conductor B, RB=10Ω
- V=20V
- In a parallel circuit, the voltage across each resistor is the same. Therefore, the current through each resistor can be calculated using Ohm's Law:
IA=VRA=20V5Ω=4A
IB=VRB=20V10Ω=2A
- The currents through A and B are different: IA=4A and IB=2A.
1. Total Resistance in Series:
- The total resistance Rtotal in series is the sum of the individual resistances:
Rtotal=RA+RB=5Ω+10Ω=15Ω
- In a series circuit, the same current flows through all components. The total current I can be calculated using Ohm's Law:
I=VRtotal=20V15Ω=43A≈1.33A
- The current through both A and B is the same in the series arrangement, and it is approximately 1.33A.
- The current through conductors A and B will be the same in the series arrangement.
Similar Questions
Two conductors A and B of resistances 5Ωand10Ω respectively are first joined in parallel and then in series. In each case the voltage applied is 20V.
(a) Draw the circuit diagram to show the combination of these conductors in each case.
(b) In which combination will the voltage across the conductors A and B be the same?
(c ) In which arrangement will the current through A and B is the same?
(d) Calculate the equivalent resistance for each arrangement.
View SolutionTwo resistors X and Y of resistances 2 ω and 3 ω respectively are first joined in parallel and then in series. In each case the voltage supplied is 5 V.
(i) Draw circuit diagrams to show the combination of resistors in each case.
(ii) Calculate the voltage across the 3 Q resistor in the series combination of resistors.
View Solution
Knowledge Check
Two conductors A and B resistance 5ωand10ω respectively are first joined in parallel and then in series. In each case the voltage applied is 20 V.
In which combination will the voltage across the conductors A and B be the same ?ASeries arrangmentBParallel arrangementCBoth of the aboveDNone of the aboveTwo conductors A and B resistance 5ωand10ω respectively are first joined in parallel and then in series. In each case the voltage applied is 20 V.
Equivalant resistance in parallel combination is:A15ωB3.33ωC0.3ωDNone of the aboveTwo conductors A and B resistance 5ωand10ω respectively are first joined in parallel and then in series. In each case the voltage applied is 20 V.
Equivalent resistance in series combination is:A15ωB3.33ωC0.3ωDNone of the above
Two cells, of voltage 10 V and 2 V and internal resistances 10Ω and 5Ω respectively, are connected in parallel as shown in Fig. Find the effective voltage and effective resistance of the combination.
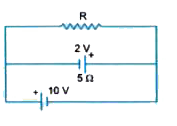
View SolutionThree resistors of 2Ω,3Ωand4Ω are connected in
(a) series
(b) parallel.
Find the equivalent resistance in each case.
View SolutionTwo cells of 1.5 V and 2 V , having internal resistances of 1Ω and 2Ω respectively, are connected in parallel so as to read the current in the same direction through an external resistance of 5Ω . The current in the external resistance will be
View SolutionTwo conductors A and B resistance 5ωand10ω respectively are first joined in parallel and then in series. In each case the voltage applied is 20 V.
Which of these circuit diagram shows the correct connection when A and B are joined in parallel?
View SolutionTwo cells of e.m.f. 2.5 V and 2.0 V having internal resistance of 1Ω and 2Ω respectively are connected in parallel with similar poles connected together so as to send the current in the same direction through an external resistance of 2Ω. The current in the external resistance is
View Solution
