O is the centre of a circle of radius 5cm. T is a point such that OT=13cm and OT intersects the circle at E, find the length AB.
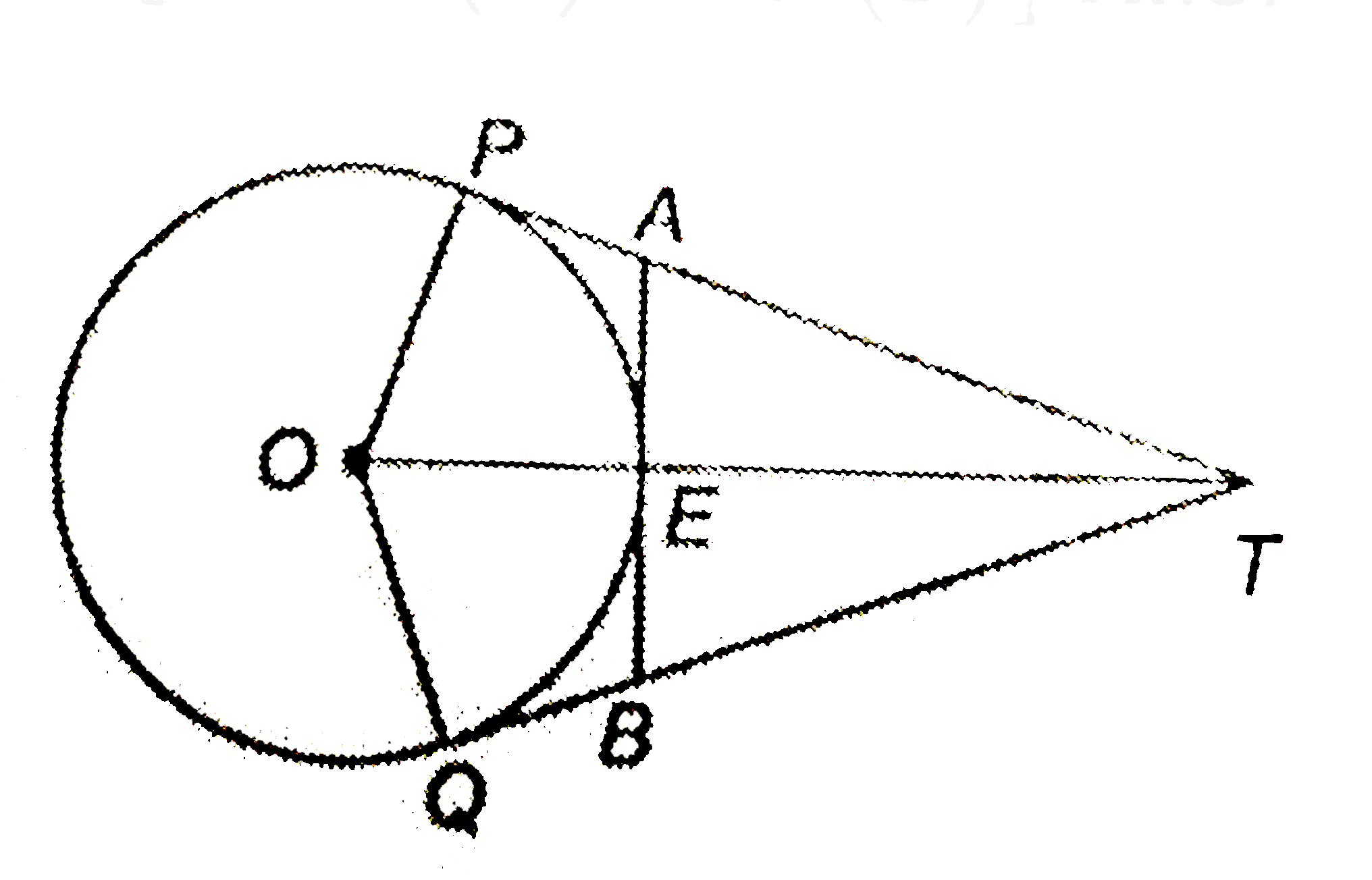
Step by step video solution for O is the centre of a circle of radius 5cm. T is a point such that OT=13cm and OT intersects the circle at E, find the length AB. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Knowledge Check
- Question 1 - Select One
Type V: O is the center of the circle of radius 5cm. T is a point such that OT=13cm and OT intersects the circle at E . If AB is the tangent to the circle at E; find the length of AB.
A103B203C163DCannot be determined - Question 1 - Select One
O is the centre of a circle of radius 5cm. T is a point such that OT=13cmandOT intersects the circle at E. If AB is the tangent to the circle at E, find length of AB.
A20 cmB203cmC10cmD25 cm - Question 1 - Select One
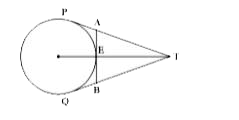
Tangents PT and QT are drawn on a circle of radius 5 cm from point T which is 13 cm away from the circle. What is the length (in cm) of AB?A103cmB203cmC403cmD163cm - Question 1 - Select One
PQ is a chord of length 8 cm, of a circle with centre O and of radius 5 cm. The tangents at P and Q intersect at a point T. The length of TP is
A203cmB214cmC103cmD154cm
Similar Questions
O is the centre of a circle of radius 5cm. T is a point such that OT=13cm and OT intersects the circle at E. If AB is the tangent to the circle at E, find length of AB.
View SolutionType V: O is the center of the circle of radius 5cm. T is a point such that OT=13cm and OT intersects the circle at E . If AB is the tangent to the circle at E; find the length of AB.
View SolutionPQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T . Find the length TP .
View SolutionAB is a chord of length 24cm of a circle of radius 13cm .The tangents at A and B intersect at a point C. Find the length AC
View SolutionPQ is a chord of length 8 cm, of a circle with centre O and of radius 5 cm. The tangents at P and Q intersect at a point T. The length of TP is
View SolutionO is the centre of a circle of radius 8 cm. The tangent at a point A on the circle cuts a line through O at B such that AB=15cm . Find OB .
View Solution

