What will come in the place of the question mark ‘?’ in the following question?
7, 14, 40, 103, 227,?
422
420
356
442
To solve the problem step by step, we will analyze the sequence: 7, 14, 40, 103, 227, ?.
Step 1: Find the differences between consecutive terms.
- First difference: 14−7=7
- Second difference: 40−14=26
- Third difference: 103−40=63
- Fourth difference: 227−103=124
So, the differences are: 7, 26, 63, 124.
Hint: Calculate the differences between each pair of consecutive numbers to identify a pattern.
Step 2: Analyze the differences.
Now, we will look at the differences we calculated: 7, 26, 63, 124.
Step 3: Find the differences of the differences.
- First difference of differences: 26−7=19
- Second difference of differences: 63−26=37
- Third difference of differences: 124−63=61
So, the second differences are: 19, 37, 61.
Hint: Calculate the differences between the differences to see if a pattern emerges.
Step 4: Analyze the second differences.
Now, we will look at the second differences: 19, 37, 61.
Step 5: Find the differences of the second differences.
- First difference of second differences: 37−19=18
- Second difference of second differences: 61−37=24
So, the third differences are: 18, 24.
Hint: Check the differences of the second differences to find a consistent pattern.
Step 6: Analyze the third differences.
Now, we will look at the third differences: 18, 24.
Step 7: Predict the next difference.
The difference between the third differences is 24−18=6. If we assume this pattern continues, the next difference in the second differences will be 61+6=67.
Hint: Use the pattern in the differences to predict the next term.
Step 8: Calculate the next second difference.
The next second difference is 124+67=191.
Step 9: Calculate the next first difference.
Now, we add this to the last first difference:
- Next first difference: 227+191=418.
Step 10: Calculate the next term in the original sequence.
Finally, we add this to the last term in the original sequence:
- Next term: 227+418=645.
Thus, the value that comes in place of the question mark ‘?’ is 645.
Final Answer: 645
- First difference: 14−7=7
- Second difference: 40−14=26
- Third difference: 103−40=63
- Fourth difference: 227−103=124
Now, we will look at the differences we calculated: 7, 26, 63, 124.
- First difference of differences: 26−7=19
- Second difference of differences: 63−26=37
- Third difference of differences: 124−63=61
Now, we will look at the second differences: 19, 37, 61.
- First difference of second differences: 37−19=18
- Second difference of second differences: 61−37=24
Now, we will look at the third differences: 18, 24.
The difference between the third differences is 24−18=6. If we assume this pattern continues, the next difference in the second differences will be 61+6=67.
The next second difference is 124+67=191.
Now, we add this to the last first difference:
- Next first difference: 227+191=418.
Finally, we add this to the last term in the original sequence:
- Next term: 227+418=645.
Similar Questions
Knowledge Check
What will come in place of the question mark (?) in the following questions ?
40%of ?=240A60B6000C960D600What will come in the place of question mark(?) in each of the following question ?
(0.¯6+0.¯7+0.¯5+0.¯4)=?A414B39C2D249What will come in the place of question mark(?) in each of the following question ?
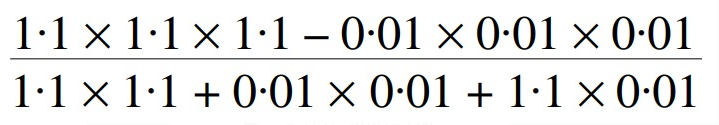 A2B2⋅09C2⋅05D1⋅09
A2B2⋅09C2⋅05D1⋅09
What will come in the place of the question mark ‘?’ in the following question? (152–114)÷3–40+23=?
View SolutionWhat will come in the place of the question mark ‘?’ in the following question?
48×7÷12+33–72=√?
View SolutionWhat will come in the place of the question mark ‘?’ in the following question? 25+41×(28÷7)–132=?
View SolutionWhat will come in place of the question mark (?) in the following questions ?
60=?% of 400
View SolutionWhat will come in place of the question mark (?) in the following questions ?
1400×?=1050
View Solution
