Direction: There are 1250 total students in 5 different classes in a school. The number of students in
class I is 50 less than the number of students in class II. The number of class IV students is 50 more
than the number of students in class II. The average number of students in class III and class V is 250. The
number of students in class III is 10% less than the number of students in class I.
The ratio of the total number of students of class V to the total number of students of class III is
To solve the problem step by step, we will define the number of students in each class based on the information provided.
Step 1: Define Variables
Let the number of students in Class I be m.
Then, the number of students in Class II will be m+50.
The number of students in Class IV will be m+100 (since it is 50 more than Class II).
Let’s denote the number of students in Class III as x and in Class V as y.
Step 2: Set Up the Equation
According to the problem, the total number of students across all classes is 1250. Therefore, we can write the equation:
m+(m+50)+(m+100)+x+y=1250
This simplifies to:
3m+150+x+y=1250
Thus, we can rearrange it to:
3m+x+y=1100(Equation 1)
Step 3: Average of Class III and Class V
The average number of students in Class III and Class V is given as 250. Therefore:
x+y2=250
This can be rearranged to:
x+y=500(Equation 2)
Step 4: Substitute Equation 2 into Equation 1
Now, we can substitute Equation 2 into Equation 1:
3m+500=1100
Solving for m:
3m=1100−500
3m=600
m=200
Step 5: Calculate Students in Each Class
Now that we have m, we can find the number of students in each class:
- Class I: m=200
- Class II: m+50=200+50=250
- Class IV: m+100=200+100=300
Step 6: Calculate Class III
The number of students in Class III is 10% less than Class I:
x=m−0.1m=0.9m=0.9×200=180
Step 7: Calculate Class V
Using Equation 2 to find Class V:
x+y=500⟹180+y=500⟹y=500−180=320
Step 8: Find the Ratio of Class V to Class III
Now we have:
- Class III: x=180
- Class V: y=320
The ratio of the total number of students in Class V to Class III is:
Ratio=yx=320180=3218=169
Final Answer
The ratio of the total number of students of Class V to the total number of students of Class III is 169.
---
Let the number of students in Class I be m.
Then, the number of students in Class II will be m+50.
The number of students in Class IV will be m+100 (since it is 50 more than Class II).
Let’s denote the number of students in Class III as x and in Class V as y.
According to the problem, the total number of students across all classes is 1250. Therefore, we can write the equation:
m+(m+50)+(m+100)+x+y=1250
This simplifies to:
3m+150+x+y=1250
Thus, we can rearrange it to:
3m+x+y=1100(Equation 1)
The average number of students in Class III and Class V is given as 250. Therefore:
x+y2=250
This can be rearranged to:
x+y=500(Equation 2)
Now, we can substitute Equation 2 into Equation 1:
3m+500=1100
Solving for m:
3m=1100−500
3m=600
m=200
Now that we have m, we can find the number of students in each class:
- Class I: m=200
- Class II: m+50=200+50=250
- Class IV: m+100=200+100=300
The number of students in Class III is 10% less than Class I:
x=m−0.1m=0.9m=0.9×200=180
Using Equation 2 to find Class V:
x+y=500⟹180+y=500⟹y=500−180=320
Now we have:
- Class III: x=180
- Class V: y=320
Ratio=yx=320180=3218=169
The ratio of the total number of students of Class V to the total number of students of Class III is 169.
Similar Questions
The total number of students in class A and class B is 96. The number of students in class A is 40% more than that of B. The average weight (in kg) of class B students is 50% more than that of class A students. If the average weight of all the students of class A and B is 58 km. Then what is the average weight of the students of class B?
View SolutionThe following bar graph shows the number of students in different classes of a school.
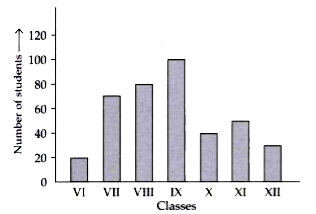
How many more students are there in Class IX than in Class VIII ?
View Solution42% of the total number of student passed in a class test. How many students failed, if the total number of students in the class is 1450 ?
View SolutionThe students of a class donated a sum of Rs 2, 209. If each student donated as many rupees as the number of students in the class, then the number of students in the class is
View SolutionThe students of a class donated a sum of Rs 2,809 to the Fund. Each student donated as many rupees as the number of students in the class. The number of students in the class is:
View SolutionDirection: There are 1250 total students in 5 different classes in a school. The number of students in class I is 50 less than the number of students in class II. The number of class IV students is 50 more than the number of students in class II. The average number of students in class III and class V is 250. The number of students in class III is 10% less than the number of students in class I.
The number of students of class I is -
View SolutionDirection: There are 1250 total students in 5 different classes in a school. The number of students in class I is 50 less than the number of students in class II. The number of class IV students is 50 more than the number of students in class II. The average number of students in class III and class V is 250. The number of students in class III is 10% less than the number of students in class I.
The total number of students of class IV and the number of students of class II is
View SolutionDirection: There are 1250 total students in 5 different classes in a school. The number of students in class I is 50 less than the number of students in class II. The number of class IV students is 50 more than the number of students in class II. The average number of students in class III and class V is 250. The number of students in class III is 10% less than the number of students in class I.
The number of students of class V is what percent of the total number of students?
View Solution