The angle of elevation of the top of a 36 m tall tower from the initial position of a person on the ground was 60∘. She walked away in a manner that the foot of the tower, her initial position and the final position were all in the same straight line. The angle of elevation of the top of the tower from her final position was 30∘. How much did she walk from her initial position?
24m
24√3m
12√3m
36√3m
To solve the problem step by step, we can use trigonometric principles involving angles of elevation and right triangles.
Step 1: Understand the Problem
We have a tower of height 36 m. The angle of elevation from the initial position of the person to the top of the tower is 60 degrees. After walking away from the tower, the angle of elevation from her final position is 30 degrees. We need to find the distance she walked away from her initial position.
Step 2: Set Up the Right Triangle for the Initial Position
From the initial position, we can form a right triangle where:
- The height of the tower (opposite side) = 36 m
- The angle of elevation = 60 degrees
- Let the distance from the base of the tower to the initial position be d1.
Using the tangent function:
tan(60∘)=OppositeAdjacent=36d1
Step 3: Solve for d1
We know that tan(60∘)=√3:
√3=36d1
Rearranging gives:
d1=36√3=12√3 meters
Step 4: Set Up the Right Triangle for the Final Position
After walking a distance x away from her initial position, the new distance from the base of the tower to her final position becomes d1+x. The angle of elevation from this position is 30 degrees.
Using the tangent function again:
tan(30∘)=36d1+x
We know that tan(30∘)=1√3:
1√3=36d1+x
Step 5: Solve for d1+x
Rearranging gives:
d1+x=36√3
Step 6: Substitute d1 into the Equation
We already found d1=12√3:
12√3+x=36√3
Step 7: Solve for x
Subtract 12√3 from both sides:
x=36√3−12√3=24√3 meters
Final Answer
The distance she walked from her initial position is 24√3 meters.
---
We have a tower of height 36 m. The angle of elevation from the initial position of the person to the top of the tower is 60 degrees. After walking away from the tower, the angle of elevation from her final position is 30 degrees. We need to find the distance she walked away from her initial position.
From the initial position, we can form a right triangle where:
- The height of the tower (opposite side) = 36 m
- The angle of elevation = 60 degrees
- Let the distance from the base of the tower to the initial position be d1.
tan(60∘)=OppositeAdjacent=36d1
We know that tan(60∘)=√3:
√3=36d1
Rearranging gives:
d1=36√3=12√3 meters
After walking a distance x away from her initial position, the new distance from the base of the tower to her final position becomes d1+x. The angle of elevation from this position is 30 degrees.
tan(30∘)=36d1+x
We know that tan(30∘)=1√3:
1√3=36d1+x
Rearranging gives:
d1+x=36√3
We already found d1=12√3:
12√3+x=36√3
Subtract 12√3 from both sides:
x=36√3−12√3=24√3 meters
The distance she walked from her initial position is 24√3 meters.
Similar Questions
The angle of elevation of the top of a tower at a point on the level ground is 30°. After walking a distance of I 00 m towards the foot of the tower along the horizontal line through the foot of the tower on the same level ground, the angle of elevation of the top of the tower is 60°. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point P on the ground is α. After walking a distance d meter towards the foot of the tower, angle of elevation is found to be β. Which angle of elevation is greater?
View Solution
Knowledge Check
The angle of elevation of top of the tower from a point P on the ground is 30∘ . If the points is 45 m away from the foot of the tower , then the height of the tower is
A45 mB15 mC15√3D20√3mThe angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower , is 30∘ . Then , the height ( in m) of the tower is .
A10√3B30√3C10√3D10The angle of elevation of the top of a tower at a point G on the ground is 30∘. On walking 20 m towards the tower the angle of elevation becomes 60∘. The height of the tower is equal to :
A10/√3mB20√3mC20/√3mD10√3m
The angle of elevation of the top of a tower from a point 40 m away from its foot is 60∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 25 m away from the foot of between the ship and tower.
View Solution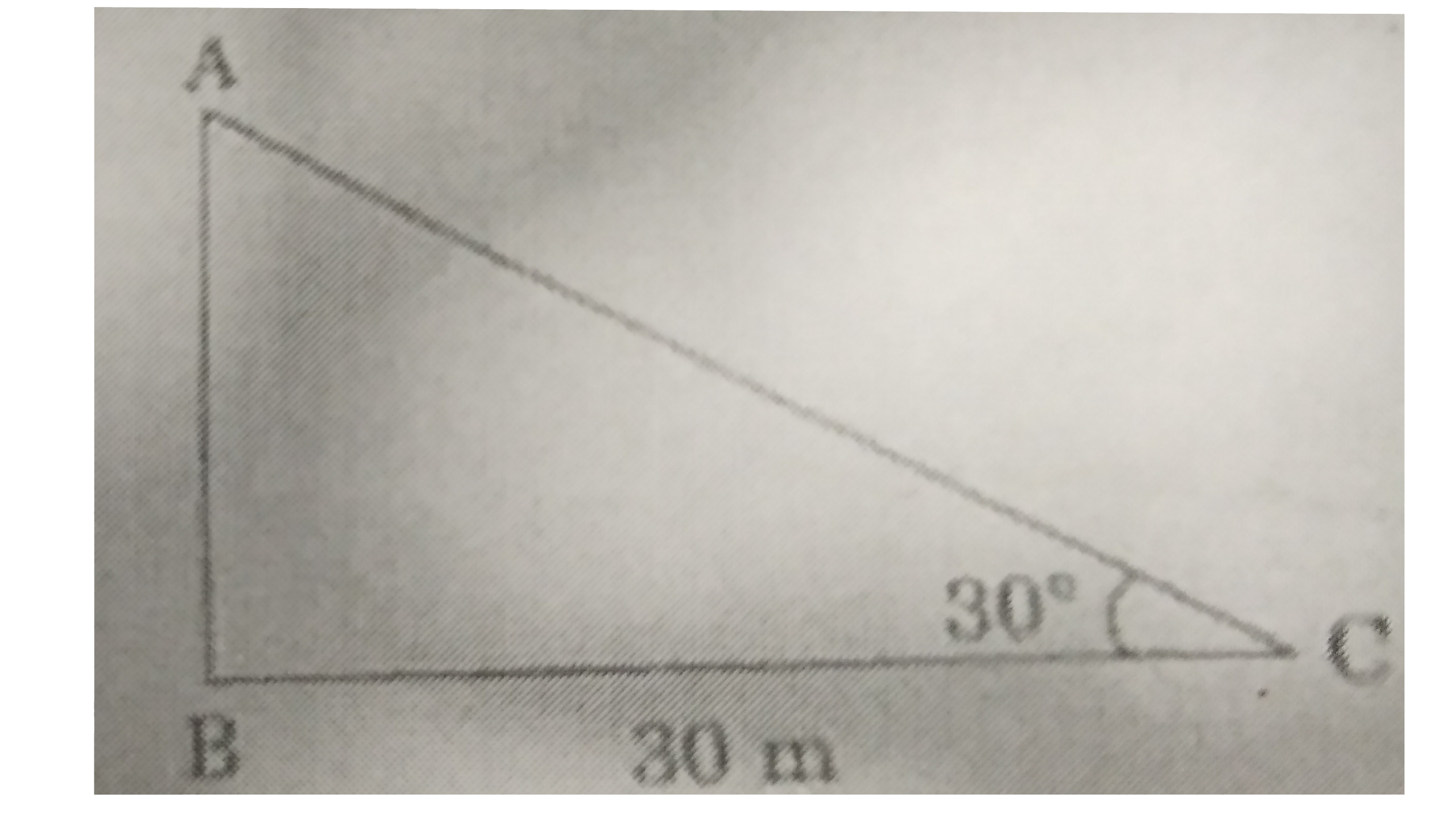
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower at a point on the ground is 30∘. If on walking 20 m toward the tower, the angle of elevation becomes 60∘, then the height of the tower is
View Solution
