Out of total of 120 musicians in a club, 5% can play all the three instruments-guitar, violin and flute. It so happens that the number of musicians who can play any two and only two of the above instruments is 30. The number of musicians who can play the guitar alone is 40. What is the total number of those who can play violin or flute alone ?
1. 30
2. 44
3. 38
4. 45
The correct Answer is:B
Let circles G, Vand F represent tlie fitasnians Wavi can play Guitar, Violin and Flute respectively.
According to the question,
Total no. of musicians in a club= n(G∪V∪F)
=a+b+c+d+e+f+g=120
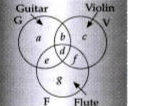
Number of musicians who can play all the three instruments
=n(G∩V∩F)=d=5%of120=5100×120=6
Number of musicians who can play any two and only two of the instruments
[n(G∩V)+n(G∩F)+n(F∩V)]=b+e+f=30
Number of musicians who can play guitar only
=n(G)=Q=40
As we know that
n(G∪V∪F)=n(G)+n(V)+n(F)+[n(G∩V)
+n(G∩F)+n(F∩V)]+n(G∩V∩F).
[∵ Formula of set theory]
Putting the values, we get
120=40+n(V)+11(F)+30+6
or, n(V)+n(F)=44.
Therefore, number of Musicians who can play violin alone or flute only
=n(V)+n(F)=c+g=44
Topper's Solved these Questions
- MATHEMATICAL ABILITY AND REASONINGBook:LUCENT PUBLICATIONChapter:MATHEMATICAL ABILITY AND REASONINGExercise:EXERCISE (Type-I: Calculation Based Problems)
Explore 47 Videos
- MATHEMATICAL ABILITY AND REASONINGBook:LUCENT PUBLICATIONChapter:MATHEMATICAL ABILITY AND REASONINGExercise:EXERCISE (Type-II: Figure Based Problems)
Explore 5 Videos
- LOGICAL VENN DIAGRAMS Book:LUCENT PUBLICATIONChapter:LOGICAL VENN DIAGRAMS Exercise:EXERCISE -4
Explore 3 Videos
- MATHEMATICAL OPERATIONS Book:LUCENT PUBLICATIONChapter:MATHEMATICAL OPERATIONS Exercise:EXERCISE-3
Explore 34 Videos
Similar Questions
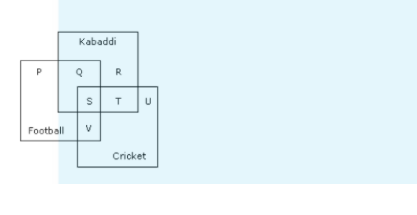
In the following Venn diagram the total number of students who play all the three games is:
View SolutionOut of a total of 85 children playing Badminton or Table Tennis or both, total number of girls in the group is 70% of the total number of boys in the group, the number of boys playing only Badminton is 50% of the number of boys and total number of boys playing Badminton is 60% of the total number of boys Number of children only playing table Tennis is 40% of the total number of children and a total of 12 children play Badminton and Table Tennis both. What is the number of girls playing only Badminton? 14 b. 16 c. 17 d. none of these
View Solution
Knowledge Check
Observe the following diagram and answer the question. Find the number of students who play any two of the three sports.
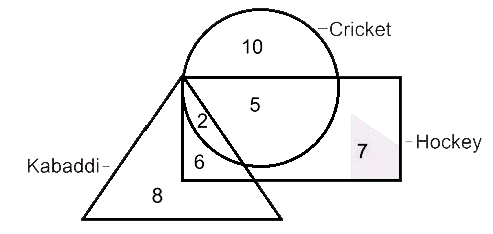 A9B15C11D13
A9B15C11D13In a class of 60 students, 40 students play cricket and only 30 students play football . The number of students who can play both cricket and football is
A10B23C33D34In a class of 100 students, 60 play cricket, 50 play Hockey and 30 play both. Then the number of students who play only one of the two game is
A80B50C30Dnone of these.
If the following pie-chart shows the preference of musical instruments of 60,000 people surveyed over wholw India.
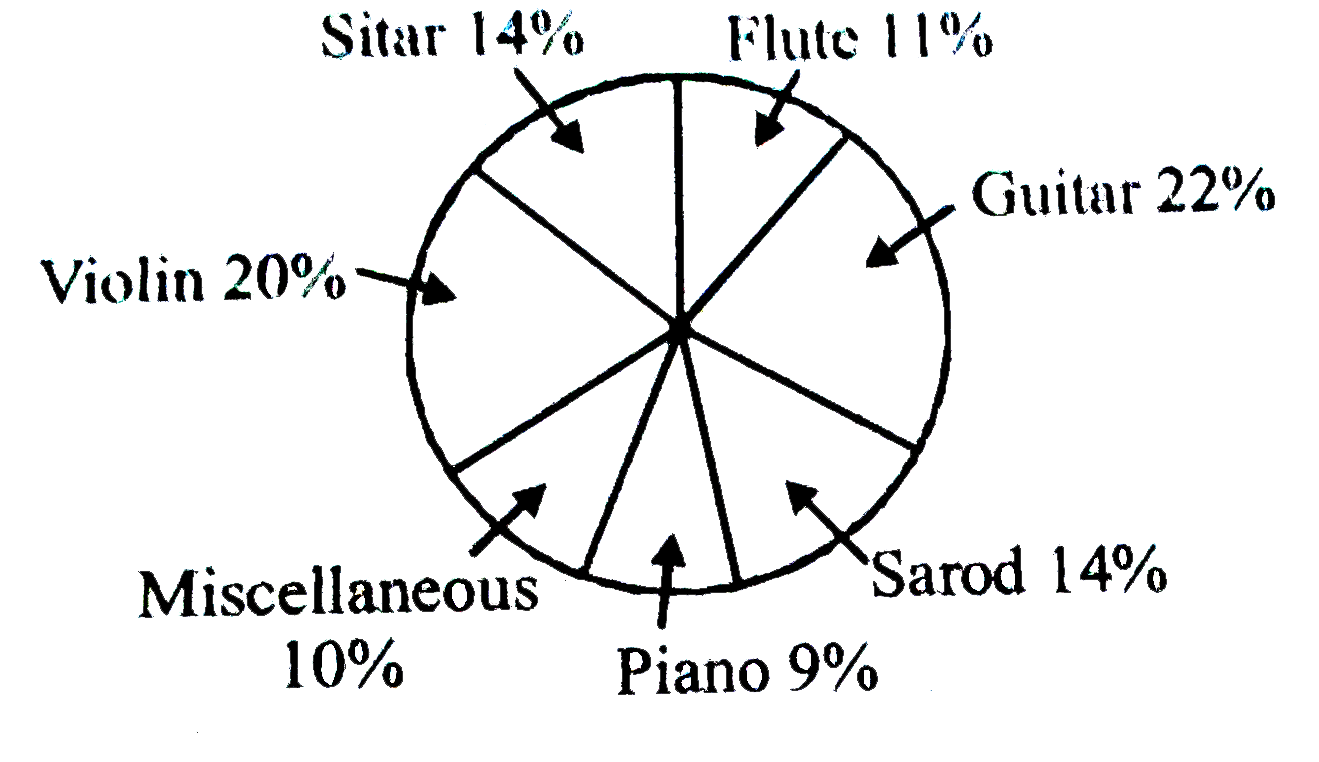
The number of people who prfer Guitar is greater than the total number of people who prefer either Flute of Piano by :
View SolutionThe given Venn diagram represents the students who can speak different languages. According to it, the total number of students who can speak exactly two languages is ______.
View SolutionIn a state with a population of 75x106, 45% of them know Hindi, 22% know English, 18% know Sanskrit, 12% know. Hindi and English, 8% know English and Sanskrit, 10% know Hindi and Sanskrit and 5% known all the three languages What is the number of people who do not know any of the above three languages? What is the number of people who know only Hindi . What is the number of people who know only Sanskrit? What is the number of people who know only English? What is the number of people who know only one language? What is the number of people who know only two languages?
View SolutionOut of 85 children playing badminton or table tennis or both, the toatl number of girls in the group is 70% of the total number of boys in the group. The number of boys playing only badminton is 50% of the number of boys and the total number of boys playing badminton is 60% of the total number of boys. The number of children playing only table tennis is 40% of the total number of children and a total of 12 children play badminton and table tennis both. The number of girls playing only badminton is
View SolutionThe following pie-chart shows the preference of musical instruments of 60,000 people surveyed over whole India. Examine the chart and answer the questions.

The number of people who prefer Guitar is greater than the total number of people who prefer either Flute or Piano by :
View Solution