There are four rods A, B, C and D of same length L but different linear mass density d, 2d, 3d & 4d respectively. These are joined to form a square frame with sides C & D along x & y axis of coordinate axes respectively. Find coordinate of centre of mass of structure. ?
The correct Answer is:C
Step by step video, text & image solution for There are four rods A, B, C and D of same length L but different linear mass density d, 2d, 3d & 4d respectively. These are joined to form a square frame with sides C & D along x & y axis of coordinate axes respectively. Find coordinate of centre of mass of structure. ? by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SAMPLE PAPER 4BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 4EXERCISE - SECTION - B
20 Videos
- SAMPLE PAPER 4BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 4EXERCISE - SECTION - C
10 Videos
- SAMPLE PAPER 3 [CBSE 2019 (TOPPER SOLVED)]BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 3 [CBSE 2019 (TOPPER SOLVED)]EXERCISE - SECTION - D
12 Videos
- SAMPLE PAPER 5BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - PART - B (SECTION - V)
4 Videos
Similar Questions
Knowledge Check
In the arrangement as shown, A and B arc two cylinders each of length L and density d1 and d2(d1<d2) respectively. They are joined together and submerged in the liquid of density d with length L/2 of A outside the liquid. If the difference in densities of two cylinders is equal to density of the liquid, find d1.
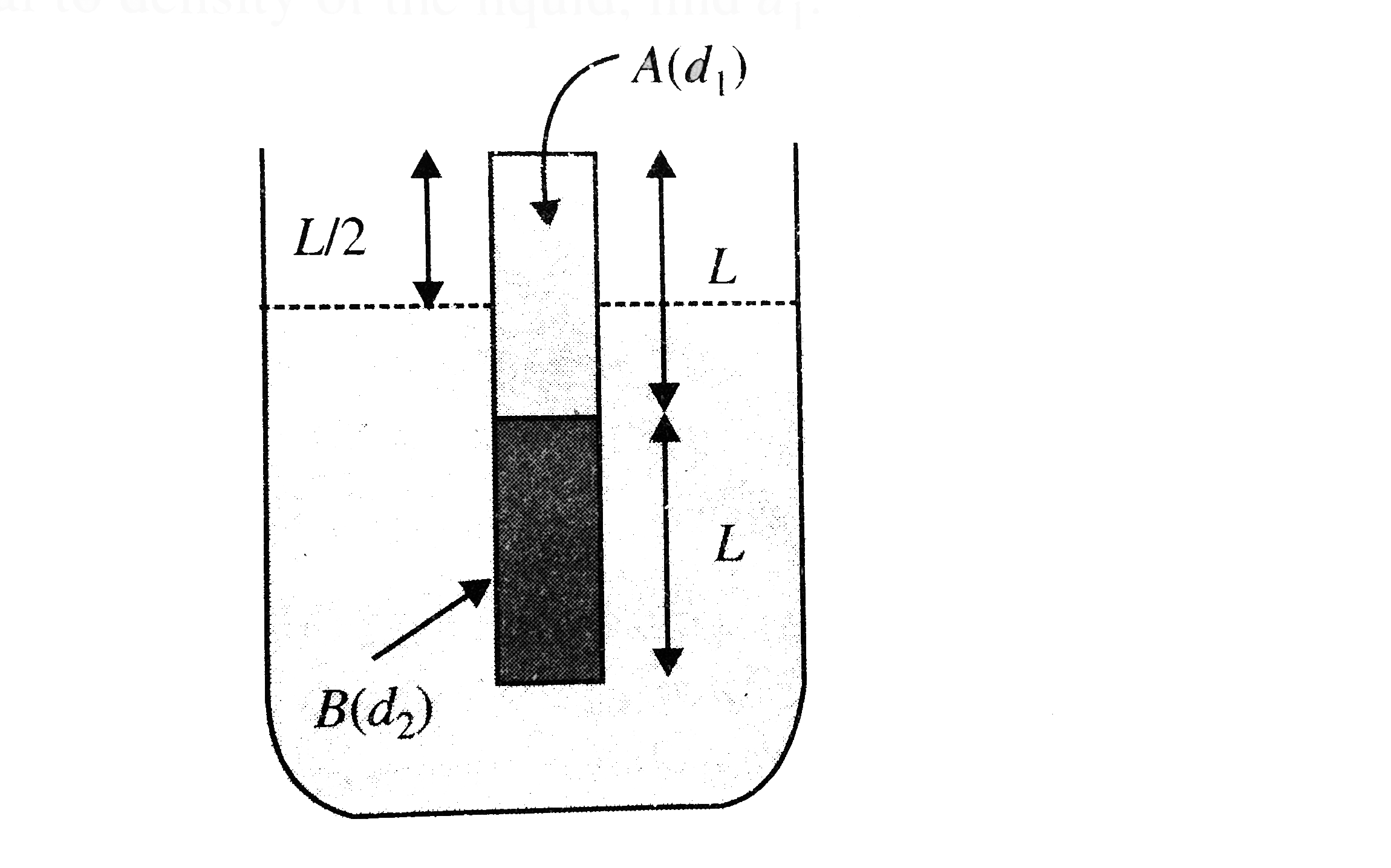 A54dBd4C34dDd2
A54dBd4C34dDd2Four particles of masses 1kg, 2kg, 3kg and 4kg are placed at the four vertices A, B, C and D of a square of side 1m. Find the position of centre of mass of the particles.
A(0.5,0.3)B(0.4,0.3)C(0.5,0.8)D(0.7,0.3)Four bodies of masses 1,2,3,4kg respectively are placed at the comers of a square of side a. Coordinates of centre of mass are (take 1kg at the origin,2kg on X-axis and 4kg on Y-axis)
A(7a10,a2)B(a2,7a10)C(a2,3a10)D(7a10,3a2)
A ,B and C are the points (2,0),(5,0)and(5,3) respectively. Find coordinates of D such that ABCD is a square.
View SolutionFour particles A,B,CandD of masses m,2m,3mand4m respectively are placed at corners of a square of side x as shown in Fig.
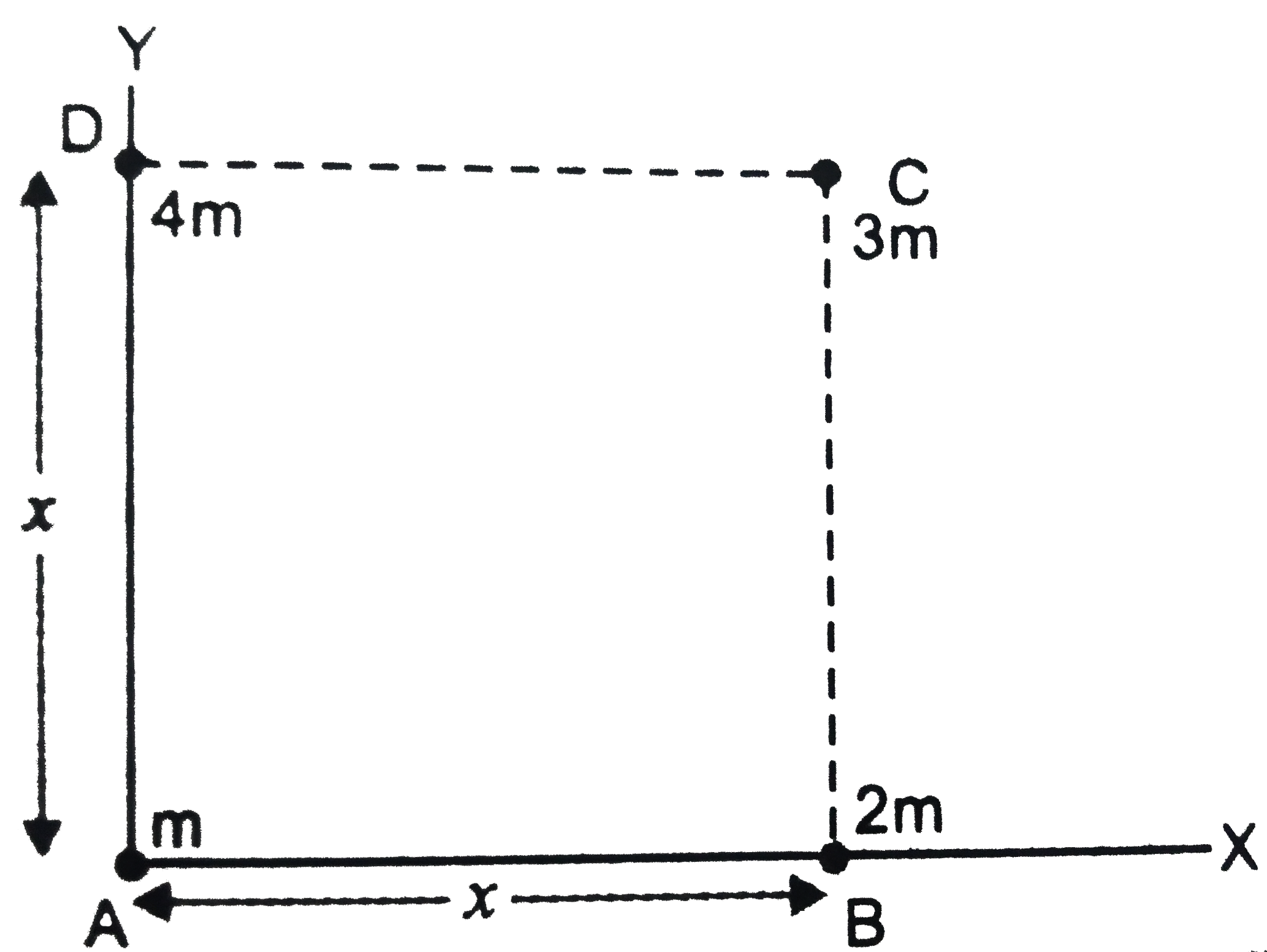
Locate the centre of mass.
View SolutionABCD is a square having length of a side 20 units. Taking the centre of the square as the origin and xandy axes parallel to AB and AD respectively, find the coordinates of A, B, C and D.
View SolutionABCD is a parallelogram. If the coordinates of A, B, C are (2,3), (1,4)and (0,−2) respectively, find the coordinates of D.
View SolutionThe position vectors of four points A, B, C, D lying in a plane are a, b, c, d respectively. They satisfy the relation |a−d|=|b−d|=|c−d| then the point D is
View Solution

