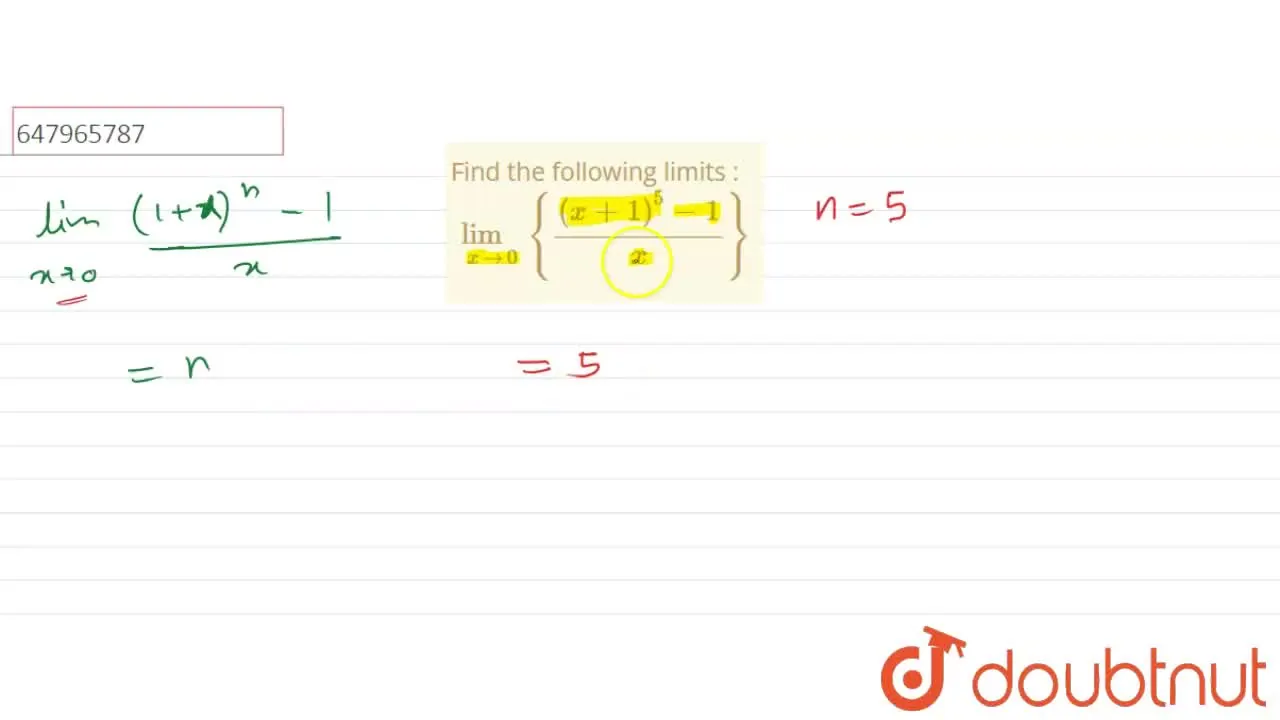
Find the following limits :
limx→0{(x+1)5−1x}
Video Solution
Text Solution
limx→0{(x+1)5−1x}
Let x+1 =y then x→0⇒y→1
The limit becomes
limx→0{(x+1)5−1x}=limy−1{y5−1y−1}=limy→1{y5−15y−1}=5.15−1=5
|
Updated on:21/07/2023
Related Playlists
Similar Questions
Find the following limits :
limx→3(x2+x+1)View SolutionFind the following limits :
limx→0{√1+x−1x}View SolutionFind the following limits :
limx→2{(x−1)(x2−x+1)}View SolutionFind the following limits :
limx→1⎧⎨⎩x15−1x1100−1⎫⎬⎭View SolutionEvaluate the following limits:
limx→2(5−x)View SolutionEvaluate the following limits:
limx→0(xcot2x)View SolutionEvaluate the following limits:
limx→0(e4x−1x)View SolutionFind the following: limx→0+({x})1/x
View SolutionEvaluate the following limits:
limx→0(2x−1x)View SolutionEvaluate the following limits:
limx→0(ex−x−1x)View Solution