Which of the following relations are functions from A to B write their domain and range if it is not a function give reason ?f={(a,b),(b,c),(c,b),(d,c)},A={a,b,c,d,e},B={b,c}
To determine whether the relation f={(a,b),(b,c),(c,b),(d,c)} is a function from set A to set B, we will follow these steps:
Step 1: Identify the sets A and B
Given:
- A={a,b,c,d,e}
- B={b,c}
Step 2: List the elements of the relation f
The relation f consists of the pairs:
- (a,b)
- (b,c)
- (c,b)
- (d,c)
Step 3: Determine the domain of the relation f
The domain of a relation consists of the first elements of each ordered pair. From the pairs in f:
- The first elements are a,b,c,d.
Thus, the domain of f is:
Domain of f={a,b,c,d}
Step 4: Determine the range of the relation f
The range of a relation consists of the second elements of each ordered pair. From the pairs in f:
- The second elements are b,c,b,c.
Thus, the range of f is:
Range of f={b,c}
Step 5: Check if f is a function from A to B
For f to be a function from A to B, each element in the domain (set A) must be associated with exactly one element in the codomain (set B).
- In set A, we have the elements a,b,c,d,e.
- In the relation f, the elements b and c are associated with multiple elements from A (for example, b is associated with c and c is associated with b).
- Additionally, the element e from set A is not associated with any element in set B.
Conclusion
Since not every element in the domain A is associated with exactly one element in B, the relation f is not a function from A to B.
Final Answer
- Domain of f: {a,b,c,d}
- Range of f: {b,c}
- Conclusion: Not a function because elements b and c are associated with multiple elements from A, and e is not associated with any element in B.
Given:
- A={a,b,c,d,e}
- B={b,c}
The relation f consists of the pairs:
- (a,b)
- (b,c)
- (c,b)
- (d,c)
The domain of a relation consists of the first elements of each ordered pair. From the pairs in f:
- The first elements are a,b,c,d.
Domain of f={a,b,c,d}
The range of a relation consists of the second elements of each ordered pair. From the pairs in f:
- The second elements are b,c,b,c.
Range of f={b,c}
For f to be a function from A to B, each element in the domain (set A) must be associated with exactly one element in the codomain (set B).
- In the relation f, the elements b and c are associated with multiple elements from A (for example, b is associated with c and c is associated with b).
- Additionally, the element e from set A is not associated with any element in set B.
Since not every element in the domain A is associated with exactly one element in B, the relation f is not a function from A to B.
- Domain of f: {a,b,c,d}
- Range of f: {b,c}
- Conclusion: Not a function because elements b and c are associated with multiple elements from A, and e is not associated with any element in B.
Topper's Solved these Questions
Similar Questions
Which of the following relations are functions from A to B write their domain and range if it is not a function give reason ? f={(a,b),(a,2),(b,3),(b,4)},A={a,b},B={b,2,3,4}
View SolutionFind the domain and range of each of the following real valued function: f(x)=ax−bcx−d .
View SolutionLabel a,b,c and d, given in Fig. Give the function of (b)

View SolutionFind which of the following are the function on set A={a,b,c}? Give reason.
(i) R1={(a,b),(b,a),(a,c)}
(ii) R2={(b,b),(c,c),(a,b)}
View SolutionLet A={a,b,c,d} and f:A→A be given by f={(a,b),(b,d),(c,a),(d,c)} , write f−1
View SolutionGiven a set A = {a, b, c, d}, then the relation:
R={(a,a),(b,b),(c,c),(d,d)} is reflexive?.
View SolutionIf A={a,b,c,d}, then a relation R={(a,b),(b,a),(a,a)} on A is
View SolutionLabel a,b,c and d. Given in figure. Give the function of (b)

View SolutionLabel A,B,C and D is the given figure. Give the function of B
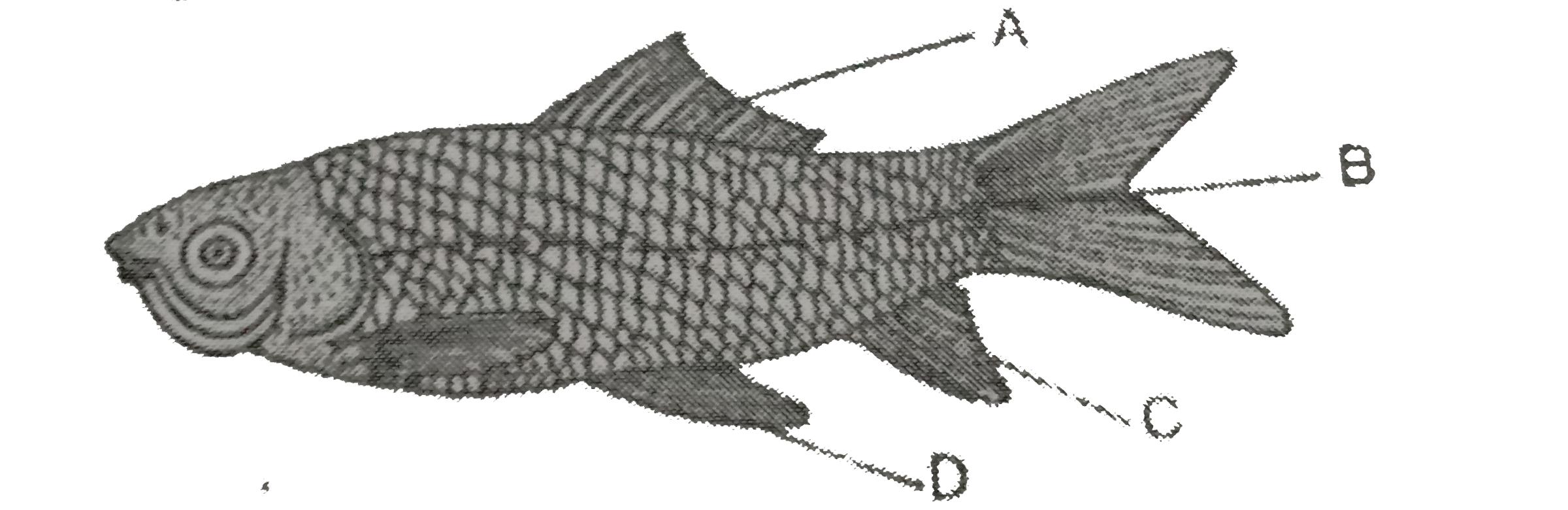
View SolutionIf A={a,b, c,d}, B={p,q,r,s} than is the following relation from A → B? Give reasons for your answer.: R1={(a,p), (b,r), (c,s)}
View Solution
