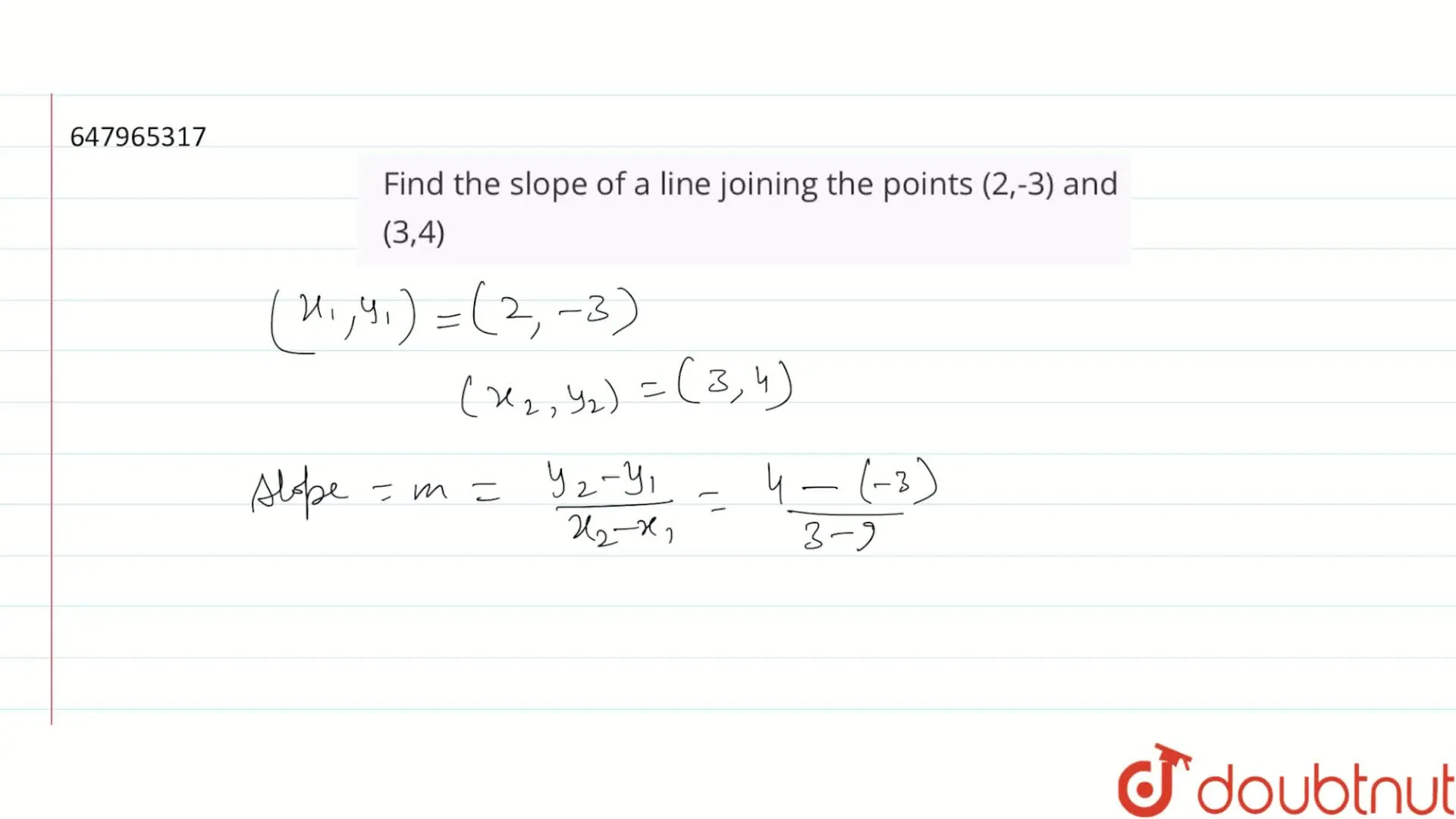
Find the slope of a line joining the points (2,-3) and (3,4)
7
Related Playlists
Similar Questions
Find the slope of the line joining the points (3,-4) and (5,2)
View SolutionFind the slope of the line joining the points (6, 3) and (8, 7) :
View SolutionFind the slope of the line joining the points (7,5) and (9,7) :
View SolutionFind the values of x ,if the slope of the line joining the points (2,5) and (x,3) is 2
View SolutionIf the slope of a line joining the points (7,3)and(k,2) is -4, then the value of k is
View SolutionIf the slope of the line joining the points (k,-3) and (-6,-8) is 54 ,then the value of k is
View SolutionThe slope of the line joining the points (2,k−3) and (4 , -7) is 3 . Find k
View SolutionThe slope of the line joining the points (3,−2) and (−1,4) is ...................
View SolutionFind the slope of the line joining points (3,8) and (−9,6) .
View SolutionIf m1 is the slope of line joining the points (−2,−1),(3,−2) and m2 is the slope of points (3,−1),(4,−6) find m1:m2
View Solution