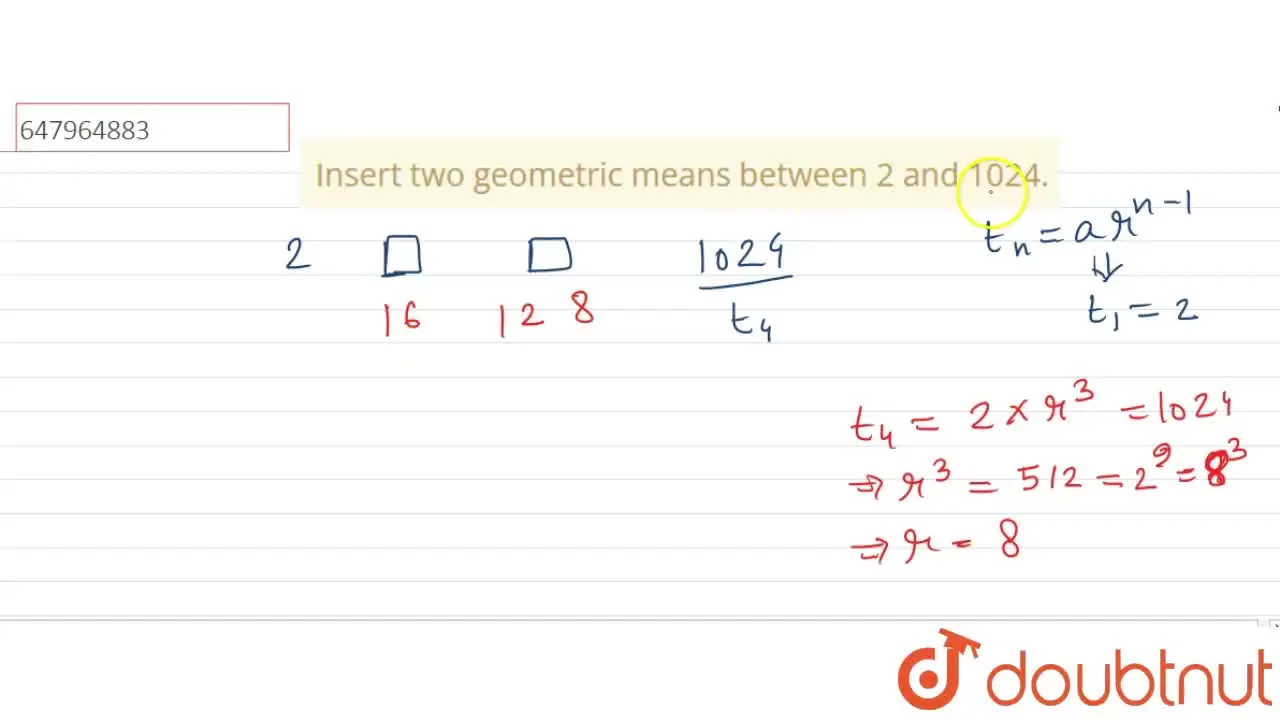
Insert two geometric means between 2 and 1024.
Video Solution
|
Answer
Step by step video & image solution for Insert two geometric means between 2 and 1024. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Related Playlists
Similar Questions
Insert two geometric means between 9 and 243.
View SolutionInsert 2 geometric means between 3 and 24
View SolutionInsert n geometric means between a and b.
View SolutionInsert four geometric means between 6 and 192.
View SolutionInsert 3 geometric means between 3 and 48.
View SolutionInsert seven geometric means between 2 and 162.
View SolutionInsert 5 geometric means between 576 and 9.
View Solution(i) Insert 4 geometric means between 256 and -8.
(ii) Insert 4 geometric means between 3 and 96.View SolutionInsert 3 geometric means between 4 and 14.
View SolutionInsert three geometric means between 13 and 432.
View Solution