Compounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
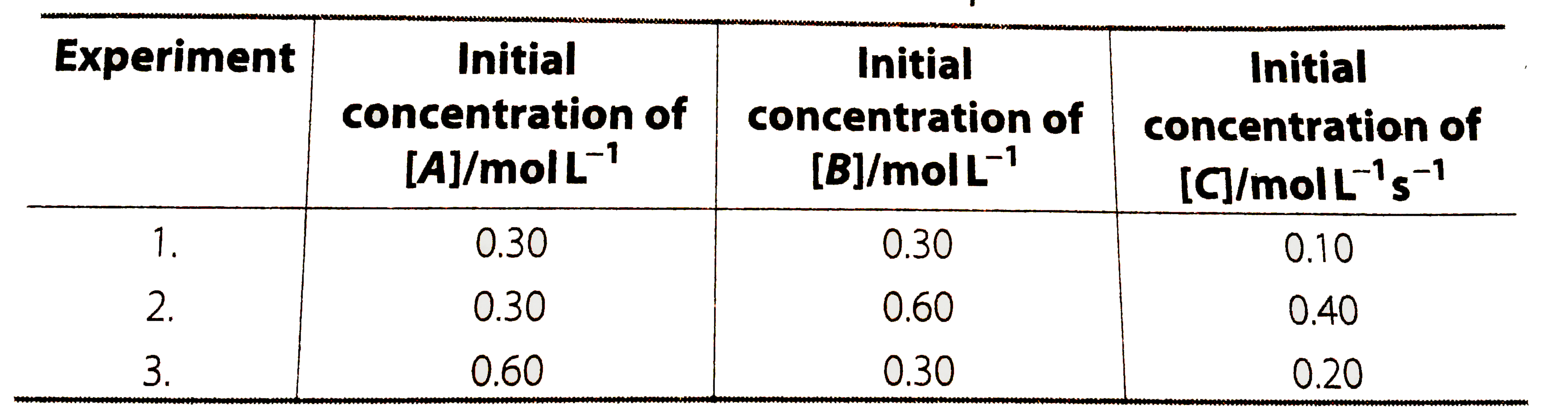
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.
Rate=k[A]2[B]
Rate=k[A][B]2
Rate=k[A][B]
Rate=k[A]2[B]0
The correct Answer is:B
Suppose order with respect to A and B are x and y respectively
Rate=k[A]x[B]y
For experiment 1,
0.1=k(0.3)x(0.3)y . . . (i)
For experiment 2,
0.4=k(0.3)x(0.6)y . . . (ii)
For experiment 3,
0.2=k(0.6)x(0.3)y . . . (iii)
Dividing equation (ii) and (i)
0.40.1=(0.6)y(0.3)y
∴y=2
Dividing equation (iii) by (i)
0.20.1=(0.6)y(0.3)y
∴x=1
Rate law
Rate=k[A][B]2
Similar Questions
For the reaction A+B→ Products, the following initial rates were obtained at various given initial concentrations.
 ltrbgt Write rate law and find the rate constant for the above reaction.
ltrbgt Write rate law and find the rate constant for the above reaction.
View SolutionCompounds A and B react according to the following chemical equation :
A(g)+2B(g)→2C(g)
Concentration of either A or B were changed keeping the concentrations of one of the reactant constants and rates were measured as a function of initial concentration, Following results were obtained.

Choose the correct option for the rate equations for this reaction.
View Solution
Knowledge Check
Compounds 'A' and 'B' react according to the following chemical equation
A(g)+2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed keeping the concentrations of one of the reactants constant and rates were measured as a function of initial concentration. Following results were obtained. Choose the correct option for the rate of equations for this reaction.
 ARate of = k[A]2[B]BRate = k[A][B]2CRate = k[A][B]DRate = k[A]2[B]−
ARate of = k[A]2[B]BRate = k[A][B]2CRate = k[A][B]DRate = k[A]2[B]−Compound 'A' and B react according to the following chemical equation.
A(g)+2B(g)→2C(g)
Concentration of either A or B were changed keeping the concentrations of one of the reactants constant and rates were mesured as a function of initial concentration. Following results were obtained. Choose the correct option for the rate equations for this reaction.
ExperimentInitial concentration of[A]/molL−1Initial concentration of[B]molL−1Initial rate of formation of[c]/molL−1s−110.300.300.1020.300.600.4030.600.300.20ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate = k[A]2[B]0Compounds A and B react according to the following chemical equation :
A(g)+2B(g)→2C(g)
Concentration of either A or B were changed keeping the concentrations of one of the reactant constants and rates were measured as a function of initial concentration, Following results were obtained.

Choose the correct option for the rate equations for this reaction.ARate = k[A]2[B]BRate = k[A][B]2CRate = k[A][B]DRate = k[A]2[B]0
Rate law for the reaction A+2B→C is found to be
Rate =k[A][B]
Concentration of reactant 'B' is doubled, keeping the concentration of 'A' constant, the value of rate constant will be ________.
View SolutionRate law for the reaction, A+2B→C is found to be
Rate =k[A][B]
Concentration of reactant 'B' is doubled keeping the concentration of 'A' constant, the value of rate constant will be _______
View SolutionRate law for the reaction, A+2B→C is found to be
Rate =k[A][B]
Concentration of reactant 'B' is doubled keeping the concentration of 'A' constant, the value of rate constant will be _______
View SolutionA(g)+3B(g)→4C(g)Initially concentration of A is equal to that of B. The equilibrium concentrations of A and C are equal. Kc is :
View SolutionFor a reaction 2A+B→2AB , it is found that doubling the concentration of both the reactants increases the rate to eight times that of initial rate but doubling the concentration of B alone doubles the rate. Then the order of the reaction with respect to A and B is
View Solution
