ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If the area of triangle ABC is , then the area of triangle BDE is equal to:
ABC और BDE दो समबाहु त्रिभुज हैं जेसे D, BC का मध्य-बिंदु है। यदि त्रिभुज ABC का क्षेत्रफल , है, तो त्रिभुज BDE का क्षेत्रफल ज्ञात करे
The correct Answer is:D
Step by step video & text solution for ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If the area of triangle ABC is 136 cm^2, then the area of triangle BDE is equal to: ABC और BDE दो समबाहु त्रिभुज हैं जेसे D, BC का मध्य-बिंदु है। यदि त्रिभुज ABC का क्षेत्रफल 136 cm^2, है, तो त्रिभुज BDE का क्षेत्रफल ज्ञात करे by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Similar Questions
ABC and BDE are two equilateral triangles such that D is the mid-point of BC . The ratio of the areas of the triangles ABC and BDE is 2:1 (b) 1:2 (c) 4:1 (d) 1:4
View SolutionIn figure, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that
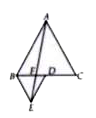
ar(ΔBDE) = 1/4 ar(ΔABC)View Solution
Knowledge Check
- Question 1 - Select One
ABC and BDE are two equilateral triangle such that D is the mid-point of BC. Ratio of the areas of triangles ABC and BDE is
A2:1B1:2C4:1D1:4 - Question 2 - Select One
ABC and BDE are two equilateral trainales, such that D is the mid-point of BC. Ratio of the area of triangles ABC and BDE is :
A1:4B1:2C2:1D4:1 - Question 3 - Select One
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then, ar(ΔBDE)=12ar(ΔABC).
ATrueBFalseCPartially trueDNone of these
In figure, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that
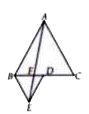
ar(ΔBDE) = 1/2 ar(ΔBAE)View SolutionIn figure, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that
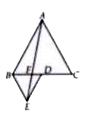
ar(ΔBFE) = 2 ar(ΔFED)View SolutionIn figure, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that
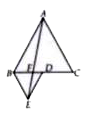
ar(ΔABC) = 2 ar(ΔBEC)View SolutionIf M is the mid point of the side BC of △ABC, and the area of △ABM is 18cm2, then the area of △ABC is:
यदि M △ABC के भुजा BC के मध्य बिंदु है, और △ABM का क्षेत्रफल 18cm2, है, तो △ABC का क्षेत्रफल है :View SolutionIn triangle ABC AD, BE, CF are medians. If the area of the triangle DGC is 20cm2 , then the area of triangle AGF + the area of triangle BGF is equal to:
त्रिभुज ABC में, AD, BE और CF मध्यरेखा हैं और G त्रिभुज का केन्द्रक है। यदि त्रिभुज DGC का क्षेत्रफल 20cm2 है, तो त्रिभुज AGF का क्षेत्रफल+ त्रिभुज BGF का क्षेत्रफल किसके बराबर है:View Solution
