A cylinder is of the height 8 m and has base radius 8 m. The maximum length of the rod that can be placed in it is
m
The correct Answer is:A
To find the maximum length of the rod that can be placed in a cylinder with a height of 8 m and a base radius of 8 m, we can follow these steps:
Step 1: Identify the dimensions of the cylinder
- Height (h) = 8 m
- Radius (r) = 8 m
- Diameter (d) = 2 * r = 2 * 8 = 16 m
Step 2: Understand the geometry
The maximum length of the rod that can be placed inside the cylinder is the length of the diagonal of the cylinder. This diagonal forms a right triangle with the height and the diameter of the base of the cylinder.
Step 3: Apply the Pythagorean theorem
According to the Pythagorean theorem:
Diagonal2=Height2+Diameter2
Let the diagonal (maximum length of the rod) be denoted as L.
Step 4: Substitute the values
Substituting the known values into the equation:
L2=h2+d2
L2=82+162
Step 5: Calculate the squares
Calculating the squares:
L2=64+256
L2=320
Step 6: Take the square root
To find L, take the square root of both sides:
L=√320
Step 7: Simplify the square root
We can simplify √320:
√320=√64×5=√64×√5=8√5
Final Answer
Thus, the maximum length of the rod that can be placed in the cylinder is:
8√5 m
- Height (h) = 8 m
- Radius (r) = 8 m
- Diameter (d) = 2 * r = 2 * 8 = 16 m
The maximum length of the rod that can be placed inside the cylinder is the length of the diagonal of the cylinder. This diagonal forms a right triangle with the height and the diameter of the base of the cylinder.
According to the Pythagorean theorem:
Diagonal2=Height2+Diameter2
Let the diagonal (maximum length of the rod) be denoted as L.
Substituting the known values into the equation:
L2=h2+d2
L2=82+162
Calculating the squares:
L2=64+256
L2=320
To find L, take the square root of both sides:
L=√320
We can simplify √320:
√320=√64×5=√64×√5=8√5
Thus, the maximum length of the rod that can be placed in the cylinder is:
8√5 m
Topper's Solved these Questions
- MENSURATIONBOOK - ARIHANT PUBLICATION PUNJABCHAPTER - MENSURATIONEXERCISE - Chapter Exercise
83 Videos
- MEASUREMENT SYSTEMBOOK - ARIHANT PUBLICATION PUNJABCHAPTER - MEASUREMENT SYSTEMEXERCISE - CHAPTER EXERCISE
111 Videos
- NUMBER SYSTEMBOOK - ARIHANT PUBLICATION PUNJABCHAPTER - NUMBER SYSTEMEXERCISE - Example
33 Videos
Similar Questions
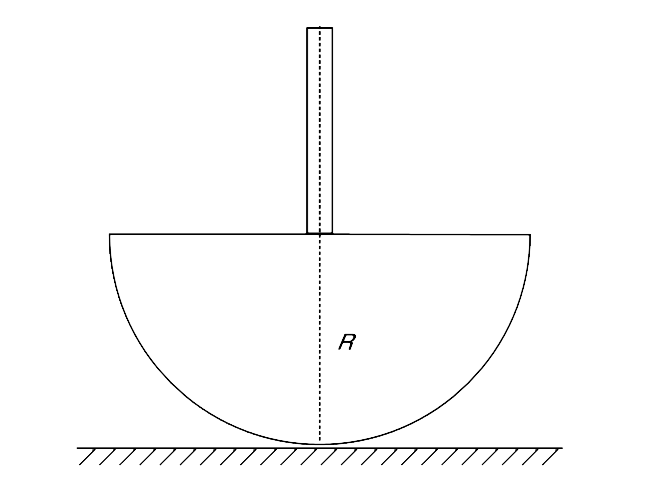
Consider the object shown in the figure. It consist of a solid hemisphere of mass M and radius R. There is a solid rod welded at its centre. The object is placed on a flat surface so that the rod is vertical. Mass of the rod per unit length is M2R. What is the maximum length of the rod that can be welded so that the object can perform oscillations about the position shown in diagram?
Note : Centre of mass of a solid hemisphere is at a distance of 3R8 from its base.
View SolutionFrom a solid cylinder whose height is 8 cm and of base radius 6 cm, a conical cavity of height 8 cm and of base radius 6 cm is formed by hollowing out. What is the inner surface area of the cavity ?
View Solution
Knowledge Check
A room is 12 m long,9 m wide and 8 m high.What is maximum length of a rod that can be kept in the room?
A17mB16mC15mD14mA hollow right circular cylinder with height 8 cm and base radius 7 cm is opened out into a rectangle What are the length and breadth of the rectangle respectively ?
A22 cm, 16 cmB44 cm, 8 cmC22 cm, 8 cmD44 cm, 16 cm
A solid iron pole consists of a cylinder of height 220 cm and base diameter 24 cm, which is surmounted by another cylinder of height 60 cm and radius 8 cm. Find the mass of the pole, given that 1 cm3of iron has approximately 8g mass.
View SolutionThe length, breadth and height of a box are respectively 14 m, 12 m and 13 m. The length of the greatest rod that can be put in it is:
View SolutionThe length and breadth of a rectangular room are 15 m and 8 m respectively:
(a) Find the perimeter of room.
(b) Find the area of the floor of room.
(c) Find the maximum possible length of the rod that can be put on the floor.
View SolutionA cone of same height and same base radius is cut from a cylinder of height 8 cm and base radius 6 cm .Find the total surface area and volume of the remaining solid.
View SolutionA solid iron pole consists of a cylinder of height 220 cm and base diameter 24 cm, which is surmounted by another cylinder of height 60 cm and radius 8 cm. Find the mass of the pole, given that 1cm3 of iron has approximately 8 gm mass. (Use ∏ = 3.14)
View SolutionThe length and breadth of a rectangular room are 15 m and 8 m respectively:
(a) Find the perimeter of room.
(b) Find the area of the floor of room.
(c) Find the maximum possible length of the rod that can be put on the floor.
View Solution
