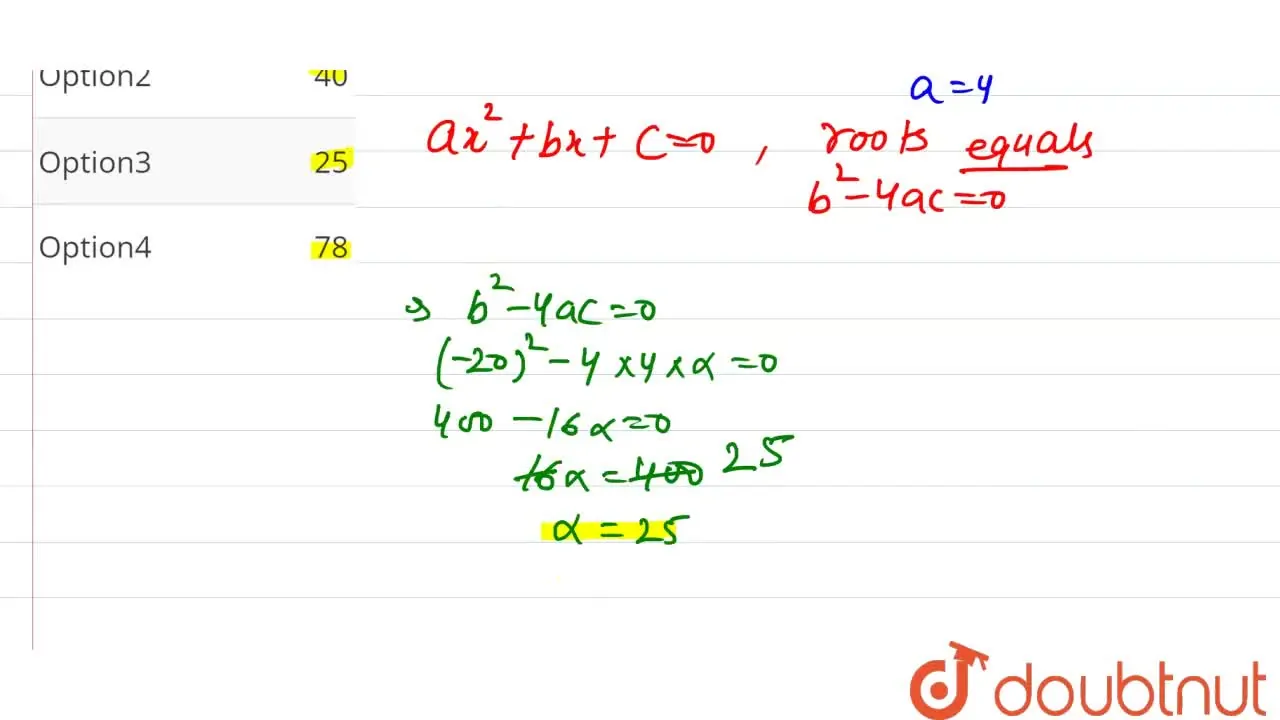
Find the value of α for which roots of equations are equal
4x2−20x+α=0
35
40
25
78
The correct Answer is:C
Step by step video, text & image solution for Find the value of alpha for which roots of equations are equal 4x ^(2) - 20 x + alpha = 0 by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
Find the values of 'α' for which 6 lies between the roots of the equation x2+2(a−3)x+9=0
View SolutionFind the value of α for which the equation (α−12)x2+2(α−12)x+2=0 has equal roots.
View SolutionLet 4x2−4(α−2)x+α−2=0(α∈R) be a quadratic equation. Find the values of α for which
One root is greater than 12 and other is smaller than 0View SolutionLet 4x2−4(α−2)x+α−2=0(α∈R) be a quadratic equation. Find the values of α for which
Both the roots are equal in magnitude but opposite in signView SolutionLet 4x2−4(α−2)x+α−2=0(α∈R) be a quadratic equation. Find the values of α for which
One root is smaller than 12 and other root is greater than 12View SolutionThe value of α for which the equation (α+5)x2−(2α+1)x+(α−1)=0 has roots equal in magnitude but opposite in sign,is
View Solutionif α,β roots of quadratic equation 2x2+3x−4=0 then find α+β
View SolutionIf α,β are the roots of the quadratic equation 4x2−4x+1=0 then α3+β3=
View SolutionLet 4x2−4(α−2)x+α−2=0(α∈R) be a quadratic equation. Find the values of α for which
Both the roots are smaller than 12View SolutionLet 4x2−4(α−2)x+α−2=0(α∈R) be a quadratic equation. Find the values of α for which
Both the roots are greater than 12View Solution