A force of 10N is applied to a stationary mass of 25kg. The work done in the 1st second is given by
10J
2J
25J
2.6J
The correct Answer is:B
To solve the problem of calculating the work done on a stationary mass of 25 kg when a force of 10 N is applied for the first second, we can follow these steps:
Step 1: Identify the given values
- Force (F) = 10 N
- Mass (m) = 25 kg
- Time (t) = 1 second
Step 2: Calculate the acceleration
Using Newton's second law of motion, we can find the acceleration (a) using the formula:
F=m⋅a
Rearranging gives:
a=Fm
Substituting the values:
a=10N25kg=0.4m/s2
Step 3: Calculate the displacement
Since the object is initially stationary, the initial velocity (u) is 0. We can use the formula for displacement (s) under constant acceleration:
s=u⋅t+12a⋅t2
Substituting the values:
s=0⋅1+12⋅0.4⋅(1)2
s=0+12⋅0.4⋅1
s=0.2m
Step 4: Calculate the work done
The work done (W) is given by the formula:
W=F⋅s⋅cos(θ)
Since the force is applied in the same direction as the displacement, θ=0 degrees, and cos(0)=1:
W=F⋅s
Substituting the values:
W=10N⋅0.2m
W=2J
Final Answer
The work done in the first second is 2 Joules.
---
- Force (F) = 10 N
- Mass (m) = 25 kg
- Time (t) = 1 second
Using Newton's second law of motion, we can find the acceleration (a) using the formula:
F=m⋅a
Rearranging gives:
a=Fm
Substituting the values:
a=10N25kg=0.4m/s2
Since the object is initially stationary, the initial velocity (u) is 0. We can use the formula for displacement (s) under constant acceleration:
s=u⋅t+12a⋅t2
Substituting the values:
s=0⋅1+12⋅0.4⋅(1)2
s=0+12⋅0.4⋅1
s=0.2m
The work done (W) is given by the formula:
W=F⋅s⋅cos(θ)
Since the force is applied in the same direction as the displacement, θ=0 degrees, and cos(0)=1:
W=F⋅s
Substituting the values:
W=10N⋅0.2m
W=2J
The work done in the first second is 2 Joules.
Similar Questions
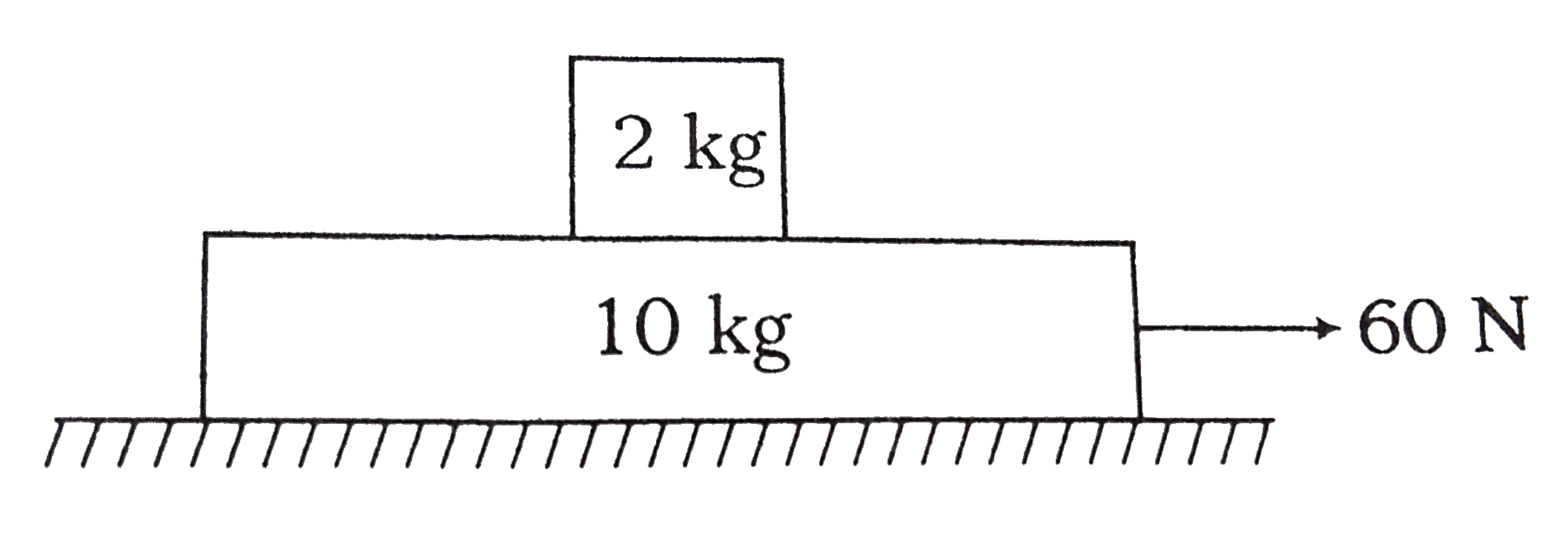
A plank of mass 10 kg and a block of mass 2 kg are placed on a horizontal plane as shown in the figure. There is no friction between plane and plank. The coefficient of friction between block and plank is 0.5 .A force of 60 N is applied on plank horizontally. In first 2 s the work done by friction on the block is
View SolutionA constant retarding force of 50 N is applied to a body of mass 10kg moving initially with a speed of 10 ms−1. The body comes to rest after
View Solution
Knowledge Check
A force of 10 N is applied to a stationary mass of 25 kg. The work done in the 1st second is given by
A10JB2JC25JD2.6JForce of 4 N is applied on a body of mass 20 kg. The work done in 3rd second is
A2 JB4 JC16 JD1.2 JForce of 4 N is applied on a body of mass 20 kg. The work done in 3rd second is
A3 jouleB2 jouleC4 jouleD1 joule
the work done by applied force is
View SolutionA block of mass 4 kg is resting on a horizontal table and a force of 10 N is applied on it in the horizontal direction. The coefficient of kinetic friction between the block and the table is 0.1. The work done in √20 s
View SolutionIf the mass of block is 1 kg and a force of 10 / √3 N is applied horizontally on the block as shown in the figure . The frictional force acting on the block is :

View SolutionIf a force of 1 N is applied on a body of 1 kg mass which is free to movement. Then, it will move with
View SolutionA cord of negligible mass is wound round the rin of a flywheel of mass 20 kg and radius 20 cm. A steady pull of 25 N is applied on the cord. The work done by the pull when 2 m of the cord is unwound is
View Solution