Consider an electron travelling with a speed V0 and entering into a uniform electric field →E which is perpendicular to →V0 as shown in the Figure.

Ignoring gravity, obtain the electron 's acceleration velocity and position as functions of time. Solution in Tamil
The speed of the electrons v0
Electric field strength =→E
Acceleration of the electrons a =?
Velocity of the electrons v=?
Position of the electrons r =?
According to Newton.s II law F=ma
The force on the electrons in an uniform electric field.
F=Ee
The e− s acceleration due to electric field
a=Fm=Eem
The acceleration of the electrons [a=Eem] is in the down direction. The horizontal velocity remains v0 as there is no acceleration in this direction.
→a=−eEm.ˆj
The downward component of the velocity of the electrons as it emerges from the field region is
v=vxˆi+vyˆj
The horizontal component of the velocity remains vx=v0. The vertical component (downward) velocity as it emerges from the field region is
vy=→at=−eEmt.ˆj
The velocity of the e−=v0ˆi−eEm.t.ˆj
The electrons starts with a velocity v0.
From equation of motion, s=ut+12at2
The position of the electrons s=r = ?
Initial velocity of the electrons u=v0
Acceleration of the electrons →a=(−eEm)t.ˆj
∴→r=v0tˆi+12.(−Eemt).ˆj
=v0tˆi−12.Eem.t2ˆj
→r=v0tˆi−12.Eem.t2ˆj.
Similar Questions
An electron (mass m)with an initial velocity →v=v0ˆi is in an electric field →E=E0ˆj .
If l,ambda0=h..mv0.it's de-Broglie wavelength at time t is given by
View SolutionElectron of mass m and intial velocity →V=v0ˆi
(V0>0) enter electric field →E=−E0ˆi(E0) constant at t=0 initial de-Broglie wavelength of electron is λ0 then at time t=t its de-Broglie wavelength will be…..
View SolutionIn a certain region of space electric field →E and magnetic field →B are perpendicular to each other and an electron enters in region perpendicular to the direction of →B and →E both and move underflected, then velocity of electron is
View SolutionA moving electron enters a uniform and perpendicular magnetic field. Inside the magnetic field, the electron travels along :
View SolutionA moving electron enters a uniform and perpendicular magnetic field. Inside the magnetic field, the electron travels along :
View SolutionAn electron projectes with velocity vecv=v_(0)hati in the electric field vecE=E_(0)hatj . There the path followed by the electron E_(0) .
View SolutionAn electron enters a chamber in which a uniform magnetic field is present as shown
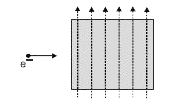
An electric field of appropriate magnitude is also applied so that the electron travels undeviated without any change in its speed thorugh the chamber. We are ignoring gravity. Then, the direction of the electric field is
View SolutionAn electron enters a chamber in which a uniform magnetic field is present as shown. Ignore gravity During its motion inside the chamber
View Solution