Compare the components for the following vector equations
(a) Tˆj−mgˆj=maˆj (b) →T+→F=→A+→B © →T−→F=→A−→B (d) Tˆj+mgˆj=maˆjSolution in Tamil
Components of the vectors
T−mg=ma
(b) ¯¯¯Tx+¯¯¯Fx+¯¯¯Bx (or) ¯¯¯Ty+¯¯¯Fy=¯¯¯Ay+¯¯¯By
© ¯¯¯Tx−¯¯¯Fx+¯¯¯Bx (or) ¯¯¯Ty−¯¯¯Fy=¯¯¯Ay+¯¯¯By
(d) T+mg=ma
Topper's Solved these Questions
- KINEMATICSBOOK - FULL MARKSCHAPTER - KINEMATICSEXERCISE - ADDITIONAL QUESTIONS SOLVED (SHORT ANSWER QUESTIONS (1 MARK))
102 Videos
- KINEMATICSBOOK - FULL MARKSCHAPTER - KINEMATICSEXERCISE - ADDITIONAL QUESTIONS SOLVED (SHORT ANSWER QUESTIONS - 1 (3 MARKS))
14 Videos
- KINEMATICSBOOK - FULL MARKSCHAPTER - KINEMATICSEXERCISE - ADDITIONAL QUESTIONS SOLVED (NUMERICAL QUESTIONS)
42 Videos
- HEAT AND THERMODYNAMICSBOOK - FULL MARKSCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - ADDITIONAL QUESTIONS SOLVED (Numerical Problems)
16 Videos
- KINETIC THEORY OF GASESBOOK - FULL MARKSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Addtional Numerical Problems
10 Videos
Similar Questions
What is meant by Frame of reference?
View SolutionIs the acceleration of a particle in circular motion not always towards the centre? Explain.
View SolutionThe V-t graphs of two objects make angle 30∘ and 60∘ with the time axis. Find the ratio of their accelerations.
View SolutionThe length of a metal wire is l1 when the tension in it is T1 and is l2 when the tension is T2. The natural length of the wire is:
View SolutionIf the rms speed of hydrogen molecules at 300K is1930ms−1. Then what is the rms speed of oxygen molecules at 1200K.
View SolutionFor two vectors →aand→b,→a,→b=|→a|∣∣→b∣∣ then (A) →a∣∣→b (B) →a⊥→b (C) →a=→b (D) none of these
View SolutionIf→a,→b,→c are three mutually perpendicular vectors, then the vector which is equally inclined to these vectors is (A) →a+→b+→c (B) →a|→a|+→b∣∣→b∣∣+→/|→c| (C) →a|→a|2+→b∣∣→b∣∣2+→c|→c|2 (D) |→a|→a−∣∣→b∣∣→b+|→c|→c
View SolutionGiven three vectors →a=ˆi−3ˆj,→b=2ˆi−tˆjand→c=−2ˆi+21ˆj such that →α=→a+→b+→c. Then the resolution of te vector →α into components with respect to →aand→b is given by (A) 3→a−2→b (B) 2→a−3→b (C) 3→b−2→a (D) none of these
View SolutionConsider three vectors →A,→Band→C as shown in fig. perform graphically the following vector additions and subtractions (a) →A+→B
(b). →A+→B+→C
(c). →A−→B
(d). →A+→B−→C
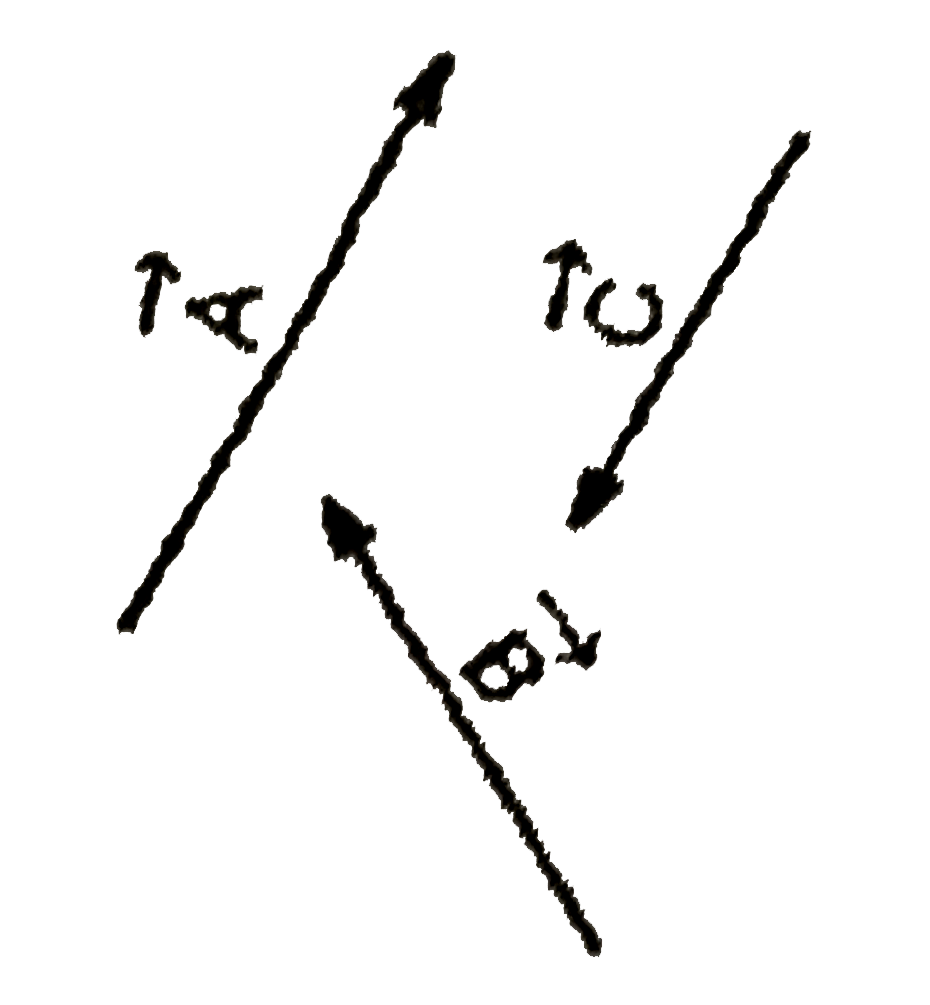
View SolutionVectors →Aand→B satisfying the vector equation →A+→B=→a,→A×→B=→band→A.→a=1. Vectors and →b are given vectosrs, are
View SolutionVectors →Aand→B satisfying the vector equation →A+→B=→a,→A×→B=→band→A.→a=1. Vectors and →b are given vectosrs, are
View SolutionIf vectors →Aand→B are such that ∣∣→A+→B∣∣=∣∣→A∣∣=∣∣→B∣∣ then ∣∣→A−→B∣∣ may be equated to
View Solutionसमीकरण →F=q(→V×→B) में निम्न के बीच कोण क्या हैं?
(a) →F तथा →v, (b) →F तथा →B , ( c) →F तथा (→v×→B)
View SolutionCompare the components for the following vector equations (a) Thatj-mghatj=mahatj (b) vecT+vecF=vecA+vecB © vecT-vecF=vecA-vecB (d) Thatj+mghatj=mahatj
View Solutionसदिश →A तथा →B इस प्रकार है कि ∣∣→A+→B∣∣=∣∣→A−→B∣∣ तो सदिश →A तथा →B के मध्य कोण होगा
View Solution[→a×→b →c×→d →e×→f] is equal to
(a)[→a→b→d][→c→e→f]−[→a→b→c][→d→e→f]
(b)[→a→b→e][→f→c→d]−[→a→b→f][→e→c→d]
(c)[→c→d→a][→b→e→f]−[→a→d→b][→a→e→f]
(d)[→a→c→e][→b→d→f]
View Solution
