Find A+BifA=[2536]andB=[7965]Solution in Kannada
Video Solution
Text Solution
The correct Answer is:(A)→(p,r);(B)→(s);(C)→(q);(D)→(s)
(A)→(p,r),(B)→(s),(C)→(q),(D)→(s)
On comparing, we get
{4f(−1)−3}a2+{4f(1)−3}a+f(2)=0
{4f(−1)−3}b2+{4f(1)−3}b+f(2)=0
and {4f(−1)−3}c2+{4f(1)−3}C+f(2)=0
It is clear that a, b, c are the roots of
{4f(−1)−3}x2+{4f(1)−3}x+f(2)=0 then
4f(−1)−3=0,4f(1)−3=0,f(2)=0
⇒f(−1)=34,f(1)=34,f(2)=0 Let f(x)=(x−2)(ax+b)
Now, f(−1)=34⇒(−3)(−a+b)=34⇒a−b=14
f(1)=34⇒(−1)(−a+b)=34⇒a+b=−34
∴a=−14,b=−12
⇒f(x)=14(4−x2)
Graph of y=f(x)
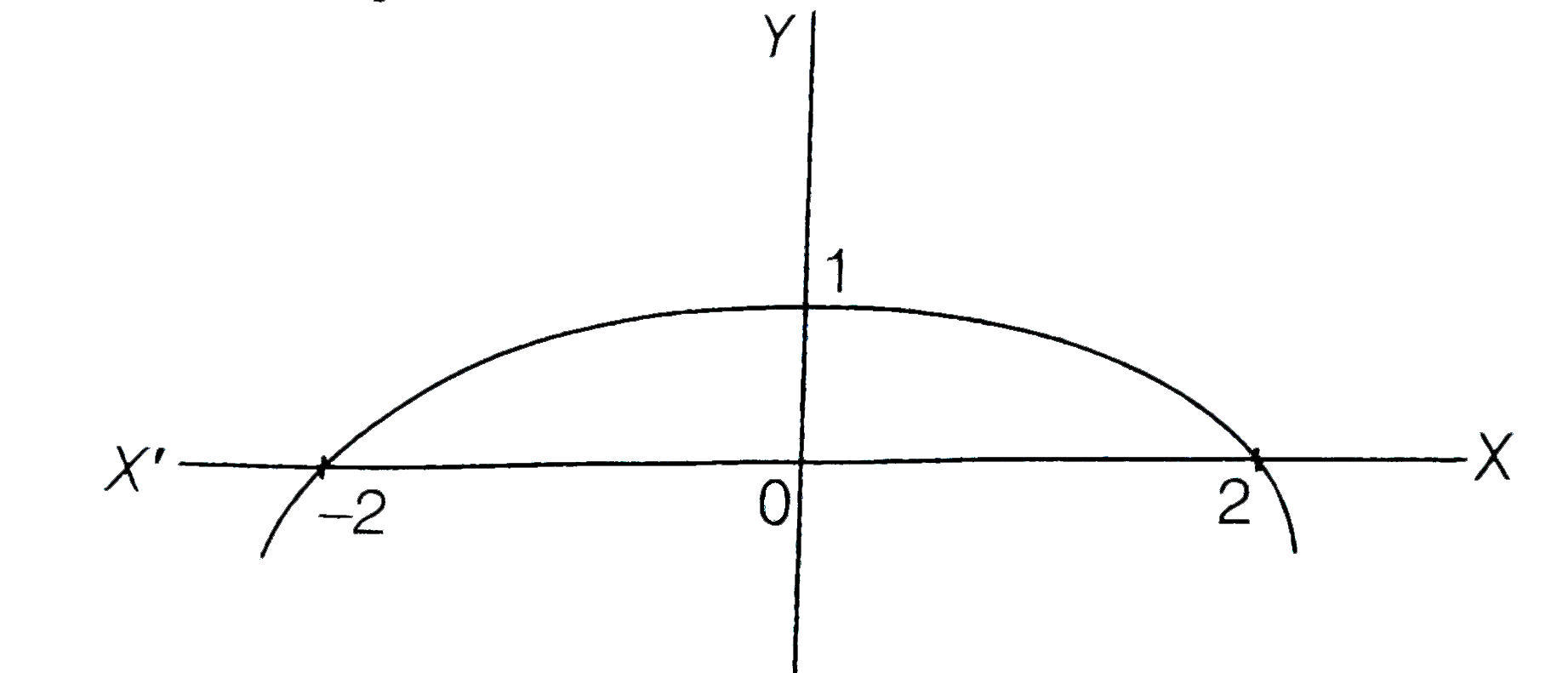
(A) x- coordinates of the point intersection of y=f(x) with
ther X- axis are - 2 and 2.
(B) Area =32∫2−214(4−x2)dx=34∫20(4−x2)dx
=34[4x−x33]20=34×163=4
(C) Maximum value of f(x) is 1.
(D) Length of intercept on the X_ axis is 4.
|
Similar Questions
If A = [[3,7],[2,5]] ਅਤੇ B = [[6,8],[7,9]] Then check that (AB)^-1 = B^-1A^-1 Is
View SolutionLet A=[3725] and B=[6879] Verify that (AB)−1=B−1A−1
View SolutionLet A =[3725] and B=[6879]Verify that (AB)−1=B−1A−1
View SolutionLet A=[3725]andB=[6879] Verify that (AB)−1=B−1A−1
View SolutionLet A={1,2,3}, B={4,5,6,7} and let f={(1,4),(2,5),(3,6)} be a function from A to B. State whether f is one-one or not.
View SolutionLet A=[(3,7),(2,5):}] and B=[(6,8),(7,9):}] then verify that (AB)^-1 =B^-1 A-1
View SolutionLet A=[3725]andB=[6879]. Verify that (AB)−1=B−1A−1.
View SolutionIf A=[1265]B=[25−34] , verify that (AB)' = B' A'
View Solution
