Suppose →a+→b+→c=0,|→a|=3,∣∣→b∣∣=5,|→c|=7, then the angle between →a&→b is Solution in Kannada
A
π2
B
π3
C
π4
D
π6
Video Solution
Text Solution
The correct Answer is:B
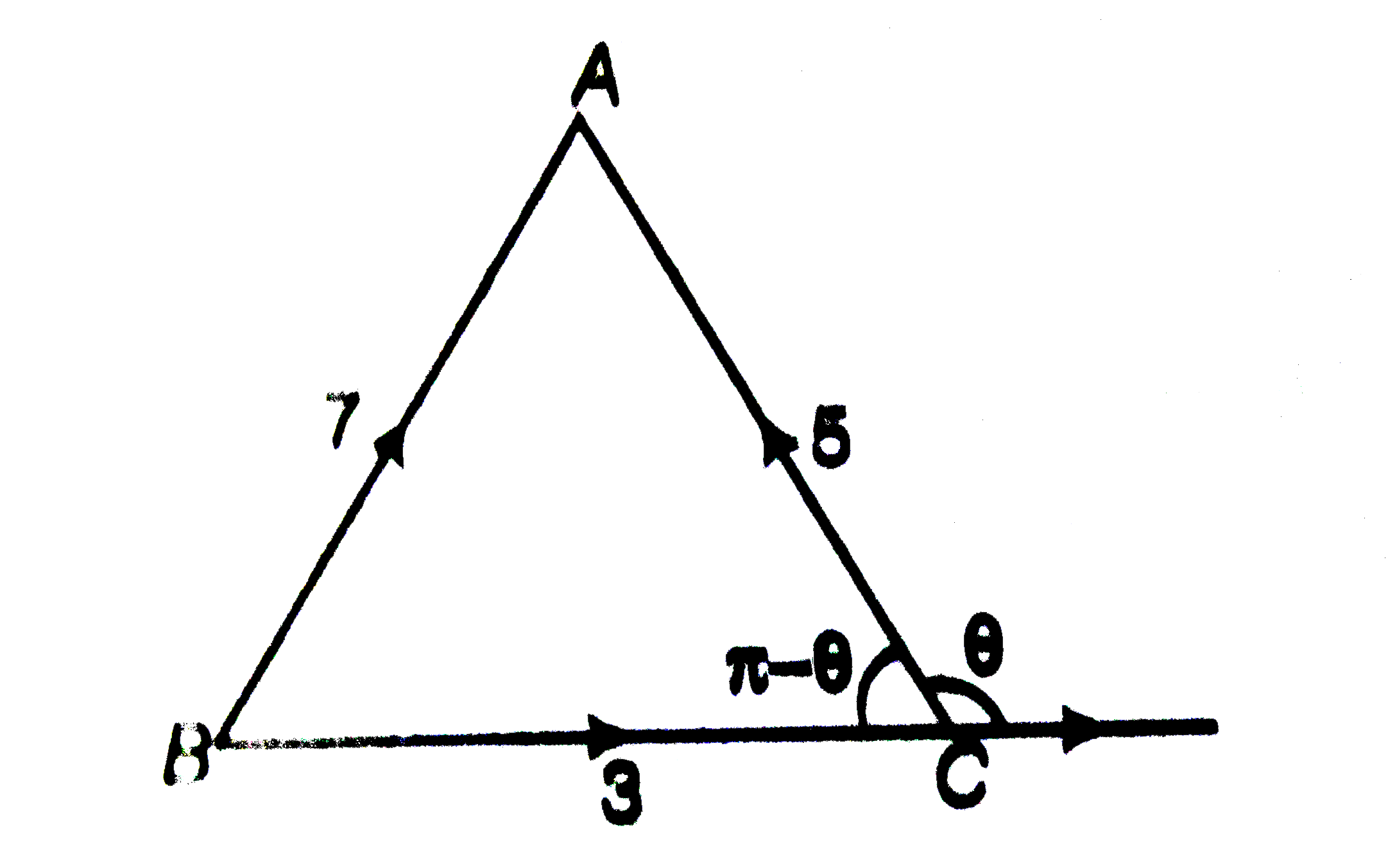
Let θ be the angle between a and b. then, ∠C=π−θ.
∴cos(π−θ)=32+52−722(3)(5)
∴−cosθ=−12
∴θ=60∘=π3
|
Similar Questions
If →a+→b+→c=0,|→a|=3,∣∣→b∣∣=5,|→c|=7 , then find the angle between →aand→b.
View Solutionयदि →a+→b+→c=0,|→a|=3,∣∣→b∣∣=5,|→c|=7 है तो →a तथा →b के मध्य कोण है
View SolutionIf →a+→b+→c = →0,|→a| = 3 , ∣∣→b∣∣ = 5 and |→c| = 7, find the angle between →a and →b.
View SolutionIf →a+→b+→c=0,|→a|=3,∣∣→b∣∣=5,|→c|=7 find the angle between →a and →b
View SolutionVectors veca,vecb, vecc are such that veca + vecb + vecc = 0 and |veca| = 3, |vecb| = 5 and |vecc]= 7 . Find the angle between veca and vecb .
View SolutionIf →a+→b+→c=→0,|→a|=3,∣∣→b∣∣=5and|→c|=7 , then the angle between →aand→b is
View SolutionIf →a+→b+→c=→0,|→a|=3,∣∣→b∣∣=5,|→c|=7, then angle between →a and →b is
View SolutionIf veca +vecb +vecc=0, |veca|=3,|vecb|=5, |vecc|=7 , then find the angle between vecb and vecc .
View Solution
