The sum of the magnitudes of two forces acting at a point is 16 N. The resultant of these forces is perpendicular to the smaller force has a magnitude of 8 N. If the smaller force is magnitude x, then the value of x is Solution in Kannada
6,10
12,6
14,4
11,7
The correct Answer is:A
We have, |P|+|Q|=18N,|R|=|P+Q|+12N
α=90∘
P+Qcosθ=0
⇒Qcosθ=−P
Now. R2=P2+Q2+2PQcosθ
⇒R2+P2+Q2+2P(−P)=Q2−P2

⇒122=(P+Q)(Q−P)=18(Q−P)
⇒Q−P=8andQ+P=18
⇒Q=13,P=5
∴ magnitude of two forces are 5 N and 13N.
Similar Questions
the sum of the magnitude of two farces acting at a point is 18 and then agnitude of their resultant is 12 N. if the resultant is at 90∘ with the smaller force of smaller magnitude what are the magnitude of forces
View SolutionThe sum of magnitudes of two forces acting at a point is 16N. If their resultant is normal to the smaller force and has a magnitude of 8N. Then the forces are
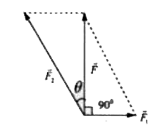
View SolutionTwo forces are such that the sum of their magnitudes is 18N and their resultant is 12 N which is perpendicular to the smaller force. Then the magnitude of the forces are
View SolutionSum of magnitudes of the two forces acting at a point is 16 N if their resultant is normal to the smaller forces and has a magnitude 8N then the forces are .
View SolutionThe sum of magnitudes of two forces acting at a point is 16 N . If the resultant force is 8 N and its direction is perpendicular to smaller force, then the forces are :-
View SolutionTwo forces are such that the sum of their magnitudes is 18N and their resultant is 12 N which is perpendicular to the smaller force. Then the magnitude of the forces are
View SolutionThe sum of the magnitudes of two forces acting at a point is 18 N and the magnitude of their resultant is 12 N. If the resultant is at 90∘ with the smaller force, the magnitude of the forces in N are
View SolutionThe sum of magnitudes of two forces acting at a point is 16 N and their resultant 8N is at 90∘ with the force of smaller magnitude. The two forces (in N) are
View Solution
