The sets S and E are defined as given below:
S={(x,y):|x−3|<1and|y−3|<1} and
E={(x,y):4x2+9y2−32x−54y+109≤0}.
Show that S⊂E. Solution in Kannada
Graph of S
∵|x−3|<1⇒−1<(x−3)<1⇒2<x<4
Similarly, |y−3|<1⇒2<y<4
So, S consists of all points inside the square (not on x≠2, 4 and y≠2, 4) bounded by the lines x = 2, y = 2, x = 4 and y = 4.
Graph of R
∵4x2+9y2−32x−54y+109≤0
⇒4(x2−8x)+9(y2−6y)+109≤0
⇒4(x−4)2+9(y−3)2≤36
⇒(x−4)232+(y−3)222≤1
So, E consists of all points inside and on the ellipse with centre (4, 3) and semi-major and semi-minor axes are 3 and 2, respectively.
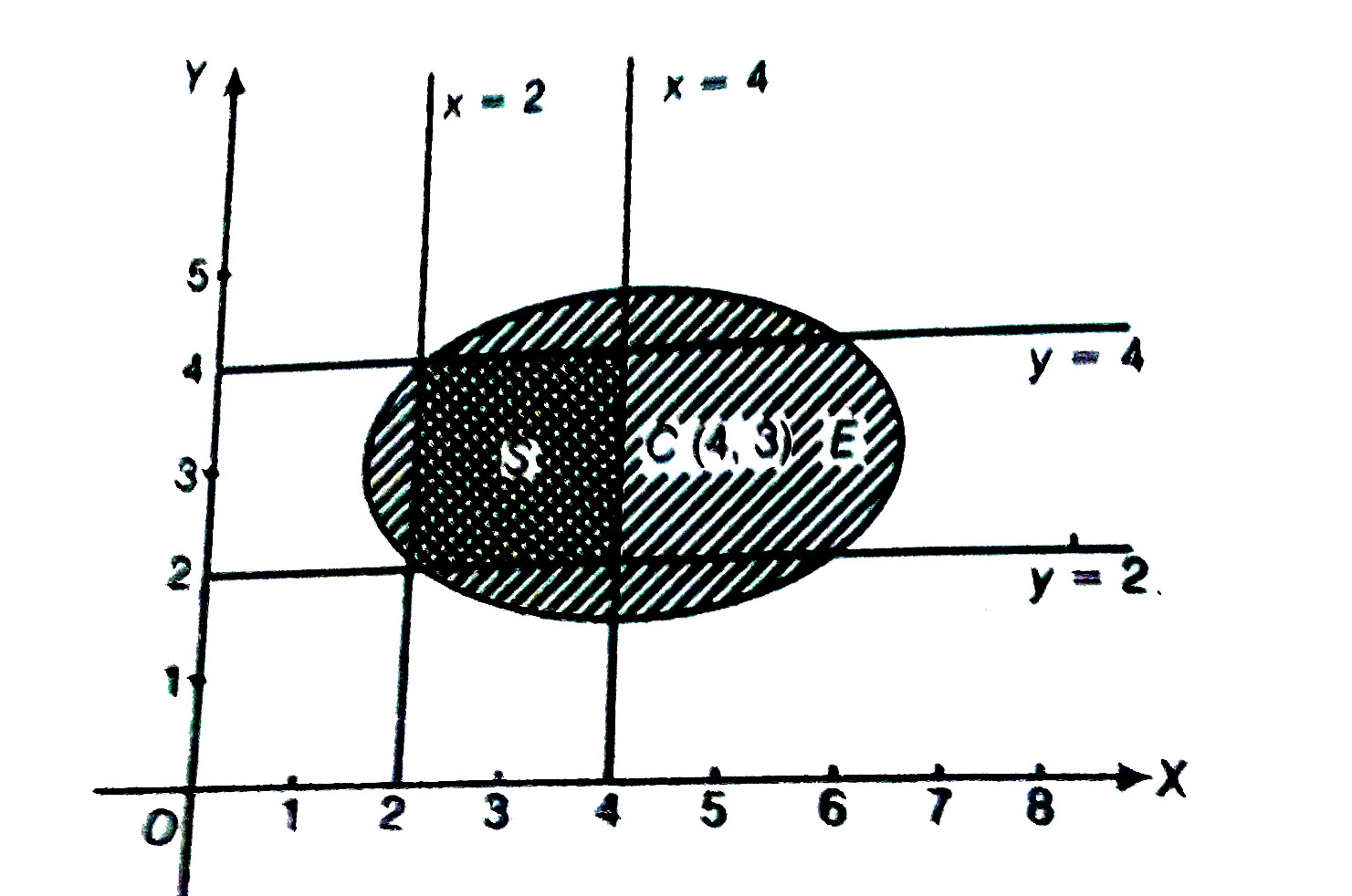
From the above graph, it is evident that the double hatched (which is S) is within the region represented by E. i.e., S⊂E
Similar Questions
ex+x=ey+y হলে দেখাও যে, d2ydx2=(ex−ey)(1−ex+y)(ey+1)3
View Solution\(CH_{3}-Mg-Br+CO_{2}\rightarrow X\xrightarrow[]{H_{2}O}\) Y In this reaction "Y' is
View SolutionIdentify the set of reagents X and Y in the following set of transformations :
CH3CH2CH2BrX−−→ProductY−−→CH3CHCH3|Br
View Solutionसमुच्चय S और E निम्नवत परिभाषित है
S={(x,y):|x−3|<1 और |y−3|<1} तथा E={(x,y):4x2+9y2−32x−54y+109≤0}, तो निम्नलिखित में से कौन-सा कथन सत्य है ?
View SolutionIf a=1,+x+x2... and b=1+y+y2+...[x∣<1and]y∣<1, then prove that 1−xy+x2y2+x3y3+....=aba+b−1
View SolutionIdentify the set of reagents/ reaction condition ‘X’ and ‘Y’ in the following set of transformations : CH_(3)-CH_(2)-CH_(2)Br overset(X)to "Product" overset(Y)to CH_(3)-underset(Br)underset(|)CH-CH_(3)
View SolutionIdentify the set ofreagent/reaction conditions X and Y, in the following set of transformations. CH_(3) -CH_(2) -CH_(2)Br overset(X) to "Product" overset(Y) to CH_(3) - underset(Br)underset(|)CH -CH_(3)
View SolutionIdentify the set of reagent and reaction conditions 'X' and 'Y' in the following set of transformation,
CH3CH2CH2BrX−−→ Product Y−−→CH3−CH−C∣BrH−CH3
View Solution
