Question

Use the principle of mathematical induction to show that $(a^n-b^n)$ is divisble by $a-b$ for all natural numbers n.

Explanation

Let `P(n)=a^n-b^n` .
Step I for `n=1`,
`P(1)=a-b` , which is divisible by `a-b`.
Therefore , the result is true for `n=1`. ,brgt Step II Assume that the result is true for `n=k` ,
i.e., `P(k)=a^k-b^k` is divisible by `a-b`.
`rArr P(k)=(a-b)r`, where r in an integer.
Step III For `n=k+1`,
`therefore P(k+1)=a^(k+1)-b^(k+1)`

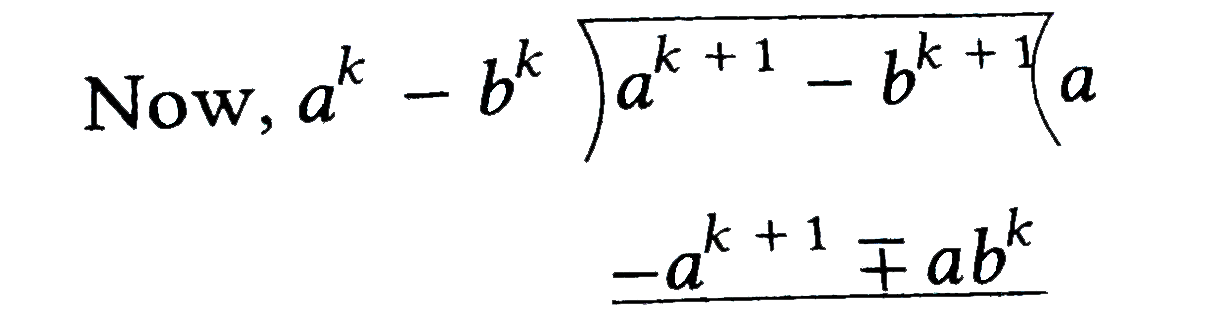
`ab6k-b^(k+1)=b^k(a-b)`
`therefore a^(k+1)-b^(k+1)=a(a^k-b^k0+b^k(a-b)`
i.e., `P(k+1)=aP(k)+b^k(a-b)`
But we know that P(k) is divisible by `a-b`. Also , `b^k(a-b)` is clearly divisible by `a-b`.
Therefore , `P(k+1)` is divisible by `a-b`.
This show that result is true for `n=k+1`.
Hence , by the principle of mathematical induction , the reuslt is true for all `n in N`.