Solve the inequality $.^{x - 1} C_{4} - .^{x - 1} C_{3} - \frac{5}{2} .^{x - 2} C_{2} < 0, x \in N$ .Solution in Kannada

Video Solution

Text Solution

Verified by Experts

We have, $.^{x - 1} C_{4} - .^{x - 1} C_{3} - \frac{5}{4} .^{x - 2} A_{2} < 0$
$\Leftrightarrow \frac{(x - 1) (x - 2) (x - 3) (x - 4)}{1 \cdot 2 \cdot 3 \cdot 4} - \frac{(x - 1) (x - 2) (x - 3)}{1 \cdot 2 \cdot 3}$
$- \frac{5}{4} \cdot (x - 2) (x - 3) < 0$
$\Leftrightarrow (x - 1) (x - 2) (x - 3) (x - 4) - 4 (x - 1) (x - 2) (x - 3) - 30 (x - 2) (x - 3) < 0$
$\Leftrightarrow (x - 2) (x - 3) {(x - 1) (x - 4) - 4 (x - 2) - 30} < 0$

$\Leftrightarrow (x - 2) (x - 3) {x^{2} - 9 x - 22} < 0$
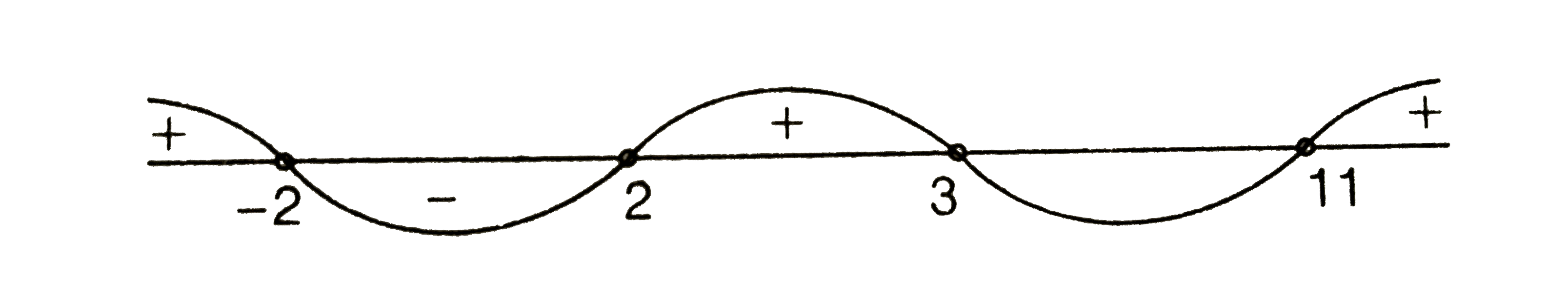
$\Leftrightarrow (x - 2) (x - 3) (x + 2) (x - 11) < 0$
From wavy curve method
$x \in (- 2, 2) \cup (3, 11)$
but $ξ n N$
$∴ x = 1, 4, 5, 6, 7, 8, 9, 10$ . . .(i)
From inequality,
$x - 1 \geq 4, x - 1 \geq 3, x - 2 \geq 2$
or $x \geq 5, x \geq 4, x \geq 4$
Hence, $x \geq 5$ . . . (ii)
From eqs.(i) and (ii) and solutions of the inequality are
$x = 5, 6, 7, 8, 9, 10$ .

Updated on:21/07/2023

|

Class 12 MATHS PERMUTATIONS AND COMBINATIONS

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile