Solve the inequation log(x−3)(2(x2−10x+24))≥log(x−3)(x2−9)Solution in Kannada
Video Solution
Text Solution
This inequation is equivalent to the collection of systems
⎧⎪⎨⎪⎩2(x2−10x+24)≥x2−9x2−9>0x−3>1
⎧⎪
⎪⎨⎪
⎪⎩2(x2−10x+24)≤x2−92(x2−10x+24)>00<x−3<1
On solving the first system, we have
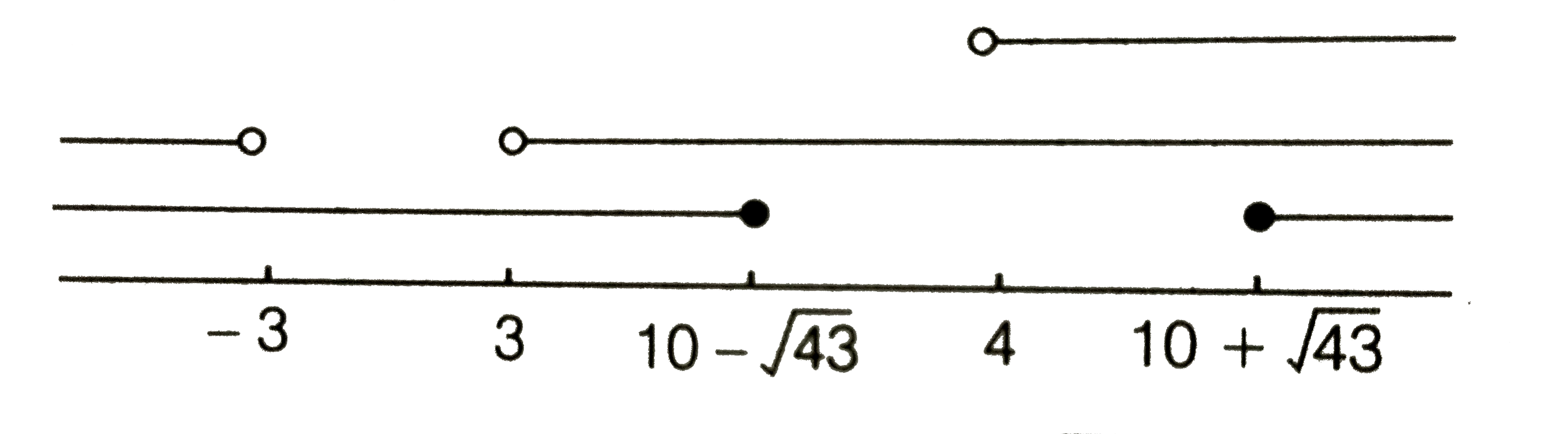
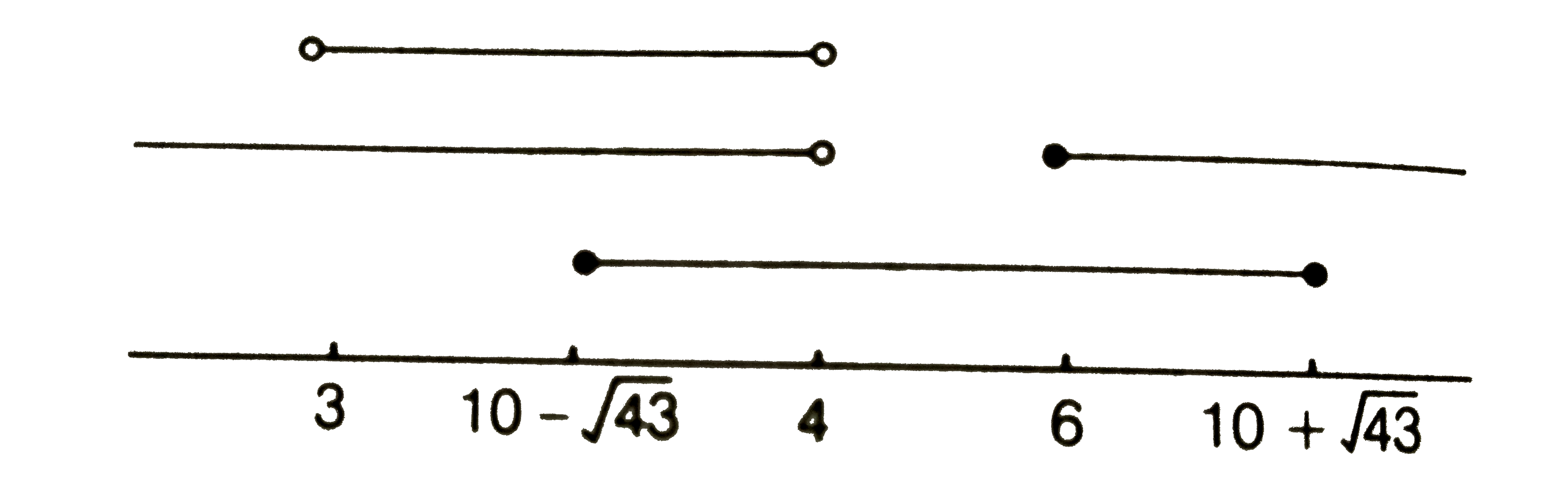
⎧⎪⎨⎪⎩x2−20x+57≥0(x+3)(x−3)>0x>4
⇔⎧⎪
⎪
⎪⎨⎪
⎪
⎪⎩xε(−∞,10−√43]∪[10+√43,∞)xε(−∞,−3)∪(3,∞)xε(4,∞)
Therefore, the system has solution
x≥10+√43
i.e. xε[10+√43,∞)
|
Similar Questions
Solve the inequation 3x+2>(19)1/x.
View SolutionSolve the inequation 22x2−10x+3+6.2x2−5x.3x2−5x≥32x2−10x+3
View SolutionIf log1/2(4−x)≥log1/22−log1/2(x−1),then x belongs to
View SolutionSolve the inequation 3x+2>(19)1/x.
View SolutionSolve the inequation 22x2−10x+3+6.2x2−5x.3x2−5x≥32x2−10x+3
View SolutionIf log_(1//2)(4-x)gelog_(1//2)2-log_(1//2)(x-1) ,then x belongs to
View SolutionSolve the inequation 3x+2>(19)1/x.
View SolutionSolve the inequation 22x2−10x+3+6.2x2−5x.3x2−5x≥32x2−10x+3
View Solution
