Solve the system of equation in x,yandz satisfying the following equations: x+[y]+{z}=3.1 {x}+y+[z]=4.3 [x]+{y}+z=5.4 (where [] denotes the greatest integer function and {} denotes the fractional part function.)Solution in Kannada
∵[x]+{x}=x,[y]+{y}=z and [z]+{z}=z
On adding all the three equations we get
2(x+y+z)=128
⇒x+y+z=6.4……i
Now adding first two equations we get
x+y+z+[y]+{x}=7.4
⇒6.4+[y]+{x}=7.4[from Eq. (i) ]
⇒[y]+{x}=1
∴[y]=1 and {x}=0..........ii
On adding last two equations, we get
x+y=z+{y}+[z]=9.7
{y}+[z]=3.3 [from Eq. (ii)]
∴[z]=3 and {y}=0.3.............iii
On adding first and last equations we get
x+y+z+[x]+{z}=8.5
⇒[x]+{z}=2.1 [from Eq. (i) ]
∴[x]=2,{z}=0.1.....iv
From Eqs i, ii and iii we get
x=[x]+{x}=2+0=2
y=[y]+{y}=1+0.3=1.3
and z=[z]+{z}=3+0.1=3.1
Similar Questions
Solve the following system of equations :
1x−1y+2z=73x+4y−5z=−52x−1y+3z=12
View SolutionUse matrix method, solve the system of linear equations :
x+y=5, y+z=3, x+z=4.
View SolutionSolve : 4{x}=x+[x](where [⋅] denotes the greatest integer function and {⋅} denotes the fractional part function.
View SolutionSolve the following system of linear equations by Cramers rule: x+y=5, y+z=3, x+z=4
View SolutionFind the value of [a] if the lines
x−23=y+42=z−15 & x+1−2=y−13=z−a4 are coplanar (where [] denotes greatest integer function)
View SolutionIf x,y,z∈R satisfies the system of equations x+(y)+(s)=12.7,[x]+{y}+z=4.1and{x}+y+[z]=2
where {.} and [.] denotes the fractional and integral parts respectively) then match the following
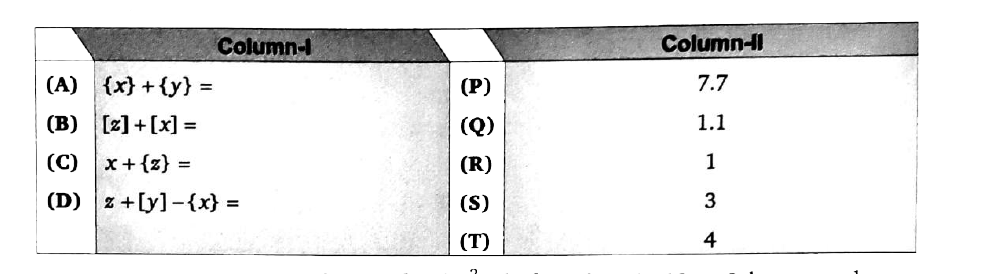
View SolutionSolve the following system of equations by using matrix inversion method.
x+y=1,y+z=53andz+x=43
View SolutionFind the value of [a] if the lines
x−23=y+42=z−15 & x+1−2=y−13=z−a4 are coplanar (where [] denotes greatest integer function)
View Solution
