Two concentric circles with radii p cm and (p+ 2) cm are drawn on a paper. The difference between their areas is 44 sq. cm What is the value of p? (Take π=227)
1.5
5
6
2.5
The correct Answer is:D
To solve the problem, we need to find the value of p given the difference in the areas of two concentric circles. Let's break it down step by step.
Step 1: Understand the areas of the circles
The area A of a circle is given by the formula:
A=πr2
For the first circle with radius p:
A1=πp2
For the second circle with radius p+2:
A2=π(p+2)2
Step 2: Calculate the area of the second circle
Using the expansion formula for (a+b)2:
(p+2)2=p2+4p+4
Thus, the area of the second circle becomes:
A2=π(p2+4p+4)=πp2+4πp+4π
Step 3: Find the difference in areas
The difference in areas between the second and first circle is given by:
A2−A1=(πp2+4πp+4π)−πp2
This simplifies to:
A2−A1=4πp+4π
Step 4: Set up the equation
According to the problem, the difference in areas is 44 sq. cm:
4πp+4π=44
Step 5: Factor out the common term
We can factor out 4π:
4π(p+1)=44
Step 6: Solve for p+1
Dividing both sides by 4π:
p+1=444π=11π
Step 7: Substitute the value of π
Given π=227:
p+1=11227=11×722=7722=72
Step 8: Solve for p
Subtracting 1 from both sides:
p=72−1=72−22=52
Thus, the value of p is:
p=2.5
Final Answer
The value of p is 2.5.
---
The area A of a circle is given by the formula:
A=πr2
For the first circle with radius p:
A1=πp2
For the second circle with radius p+2:
A2=π(p+2)2
Using the expansion formula for (a+b)2:
(p+2)2=p2+4p+4
Thus, the area of the second circle becomes:
A2=π(p2+4p+4)=πp2+4πp+4π
The difference in areas between the second and first circle is given by:
A2−A1=(πp2+4πp+4π)−πp2
This simplifies to:
A2−A1=4πp+4π
According to the problem, the difference in areas is 44 sq. cm:
4πp+4π=44
We can factor out 4π:
4π(p+1)=44
Dividing both sides by 4π:
p+1=444π=11π
Given π=227:
p+1=11227=11×722=7722=72
Subtracting 1 from both sides:
p=72−1=72−22=52
Thus, the value of p is:
p=2.5
The value of p is 2.5.
Similar Questions
Two concentric circle of radii 3 cm and 5 cm are given. Then length of chord BC which touches the inner circle at P is equal to
View SolutionIn the adjoining figure, two concentric circle with centre O have radii 21 cm and 42 cm. If ∠AOB=60∘, find the area of the shaded region. (Use π=227)

View Solution
Knowledge Check
Two concentric circle of radii 3cm and 5cm are given. Find the chord BC which touches the inner circle at P.
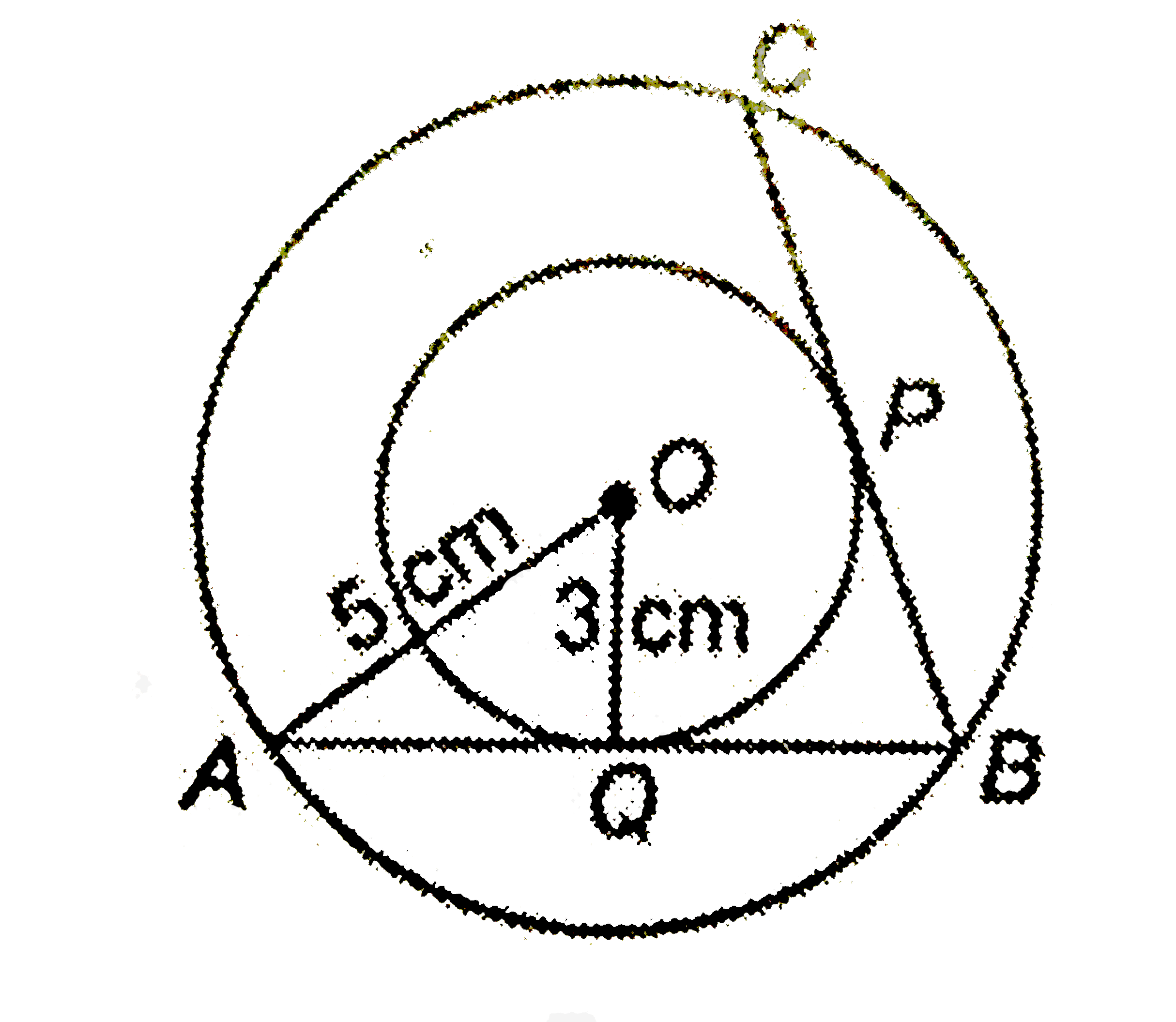 A5cmB8cmC9cmDNone
A5cmB8cmC9cmDNoneThe surface area (in sq.cm.) of a sphere with radius 2 cm is: ( Take π=227)
A352/7B350/21C352/21D350/7The radii of two concentric circles are 4 cm and 5 cm. The difference in the areas of these two circles is :
AπB7πC9πD13π
The surface area (in sq. cm) of a sphere with radius 3.5 is : (Take π=227)
View SolutionThe difference between the areas of two concentric circles is 88 cm2 If the radius of the inner circle is 6 cm. then the area (in cm2) of the larger circle is closest to: (Take π=227)
View SolutionThe surface area (in sq.cm.) of a sphere with radius 2 cm is: ( Take π=227)
View SolutionThe surface area (in sq.cm.) of a sphere with radius 4 cm is: ( Take π=227)
View SolutionTwo concentric circles are of radius 15 cm and 9 cm. What is the length of the chord of the larger circle which is tangent to the smaller circle?
त्रिज्या 15 सेमी तथा 9 सेमी वाले दो संकेंद्री वृत्त हैं | बड़े वृत्त की जीवा की लंबाई कितनी होगी जो छोटे वृत्त पर स्पर्श रेखा है ?
View Solution
