Question

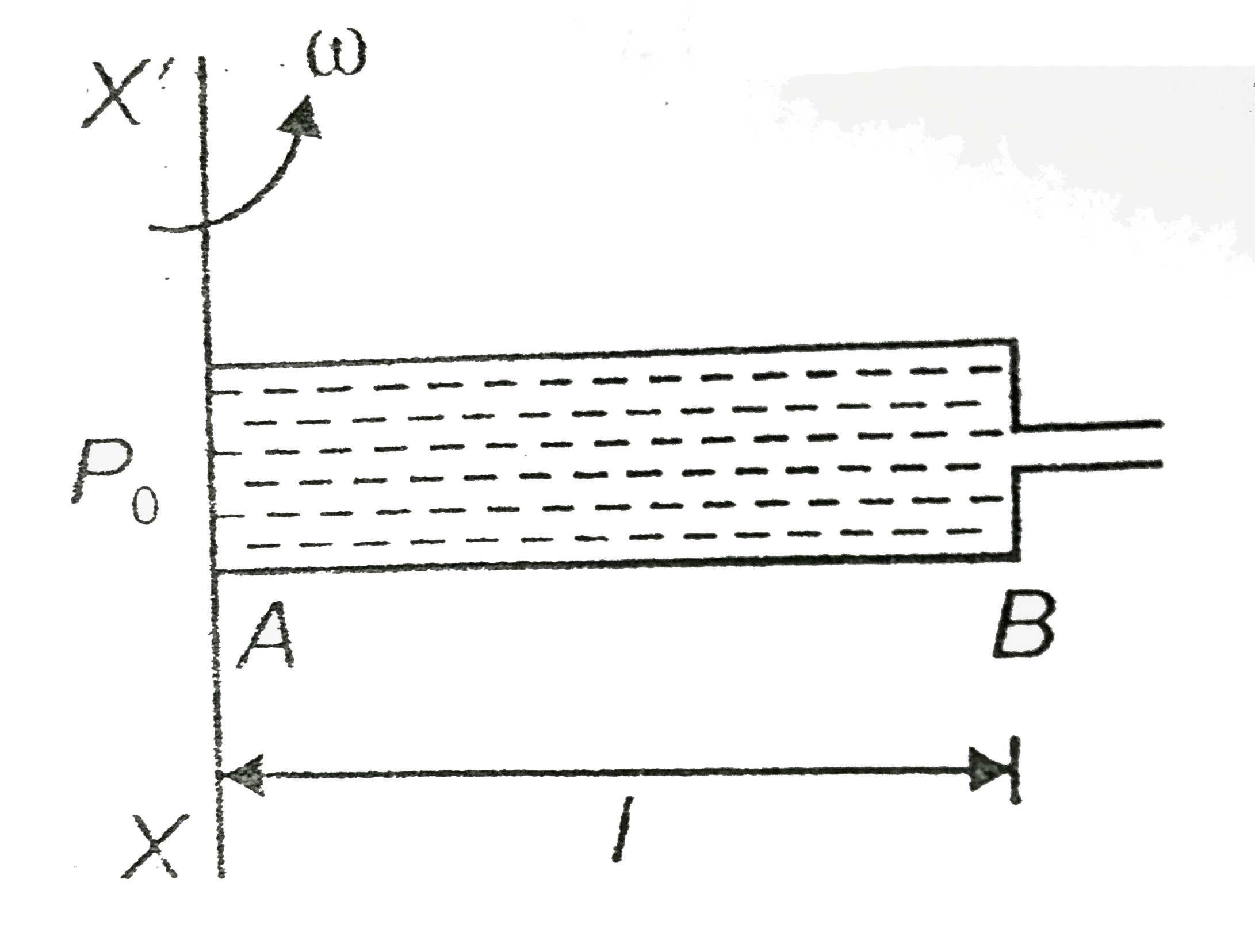
A horizontal tube of length I closed at both ends contains an ideal gas of molecular weight M, The tube is roatated at a constant angular velocity $\omega$ about a vertical axis passing through an end. Assuming the temperature to be uniforme and constant, show that
$p_2=p_1{e}^{\frac{M{\omega }^2{l}^2}{2RT},}$
where p_(2) and p_(1)denote the pressures at the free end and the fixed end respetively.

Explanation

Consider an element of the gas between the cross sections at distances x and `x+dx`from the fixed end . If p be the pressure at x and `p+dp at x+dx,`the force acting in the element towards the centre is Adp, where A is the cross sectional area. As this element is going in a circle of radius X,
`Adp=(dm)(omega^(2))x ...(i)`
where dm=mass of the element. Using `pV=nRT` on this element,
`pAdx=(dm)/(M)RT`
`or,dm=(MpA)/(RT)dx.`
Putting in (i),
`Adp=(MpA)/(RT)(omega^(2))xdx`
`or, int_(p_(1))^(p_(2)) (dp)/(p)=int_(0)^l(m(omega^(2)))/(RT)xdx`
`Inp_(2)/p_(1)=(M(omega^(2))l^(2))/(2RT)`
`(p_2 = p_1) e^((M_(omega) ^2 l^2 )/(2RT)`. `