In the triangle given below, D and E are mid points of AF and AG respectively. F and G are mid points of AB and AC respectively. If DE = 2.4 cm. then BC is equal to:
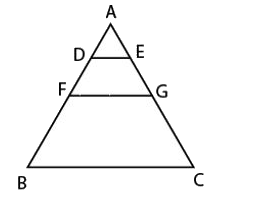
4.8 cm
3.6 cm
9.6 cm
7.2 cm
The correct Answer is:C
Step by step video, text & image solution for In the triangle given below, D and E are mid points of AF and AG respectively. F and G are mid points of AB and AC respectively. If DE = 2.4 cm. then BC is equal to: by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Similar Questions
In the given triangle, D and E is the middle point of AF and AG, respectively, F and G are the midpoint of AB and AC respectively. If DE is 2.4 cm then what is the value of BC?
नीचे दिए गए त्रिभुज में , D और E क्रमश : AF और AG के मध्य बिंदु है , F और G क्रमश : AB और AC के मध्य बिंदु है | यदि DE =2.4 cm है तो BC का मान कया है?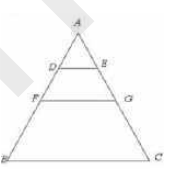
View SolutionIf in a triangle ABC and D, E are the mid-points of AB and AC respectively, then →DE is equal to
View Solution
Knowledge Check
If in a triangle AB=a,AC=b and D,E are the mid-points of AB and AC respectively, then DE is equal to
Aa4−b4Ba2−b2Cb4−a4Db2−a2Area of ΔABC=30cm2. D and E are the mid - points of BC and AB respectively. Find A(ΔBDE) :
A10cm2B7.5cm2C15cm2Dnone of theseIf D, E, F are respectively the mid-points of AB, AC and BC respectively in a ΔABC, then −−→BE+−−→AF=
A−−→DCB12−−→BFC2−−→BFD32−−→BF
In a triangle ABC,DandE are the mid-points of BC,CA respectively. If AD=5,BC=BE=4,then CA=
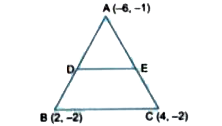
View SolutionIn the given Fig. 3, in ΔABC,DandE are the mid point of the sides AB and AC respectively. Find the length of DE. Prove that DE=12BC.
View SolutionIn the adjoining figure D, E and F are the mid - points of the sides BC, AC and AB respectively to triangle. Show that ∠EDF=∠A, ∠DEF=∠B and ∠DFE=∠C:

View SolutionArea of ΔABC=30cm2. D and E are the mid - points of BC and AB respectively. Find A(ΔBDE) :
View SolutionIn Δ ABC, DE || AC, D and E are two points on AB and CB respectively, If AB=10 cm and AD =4 cm, then BE : CE is
View Solution
