Question

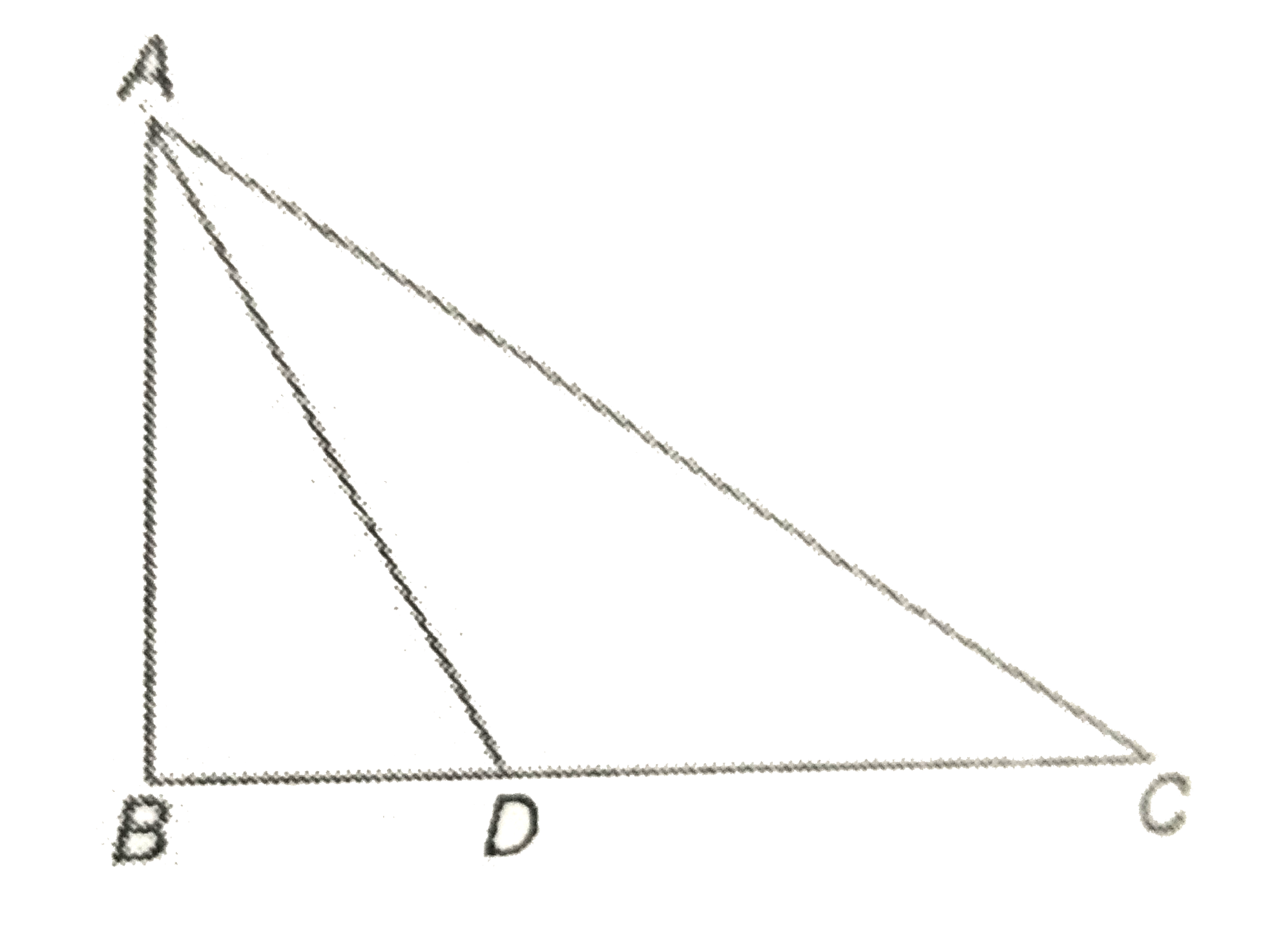
In a $\Delta ABC$, angle BAC = ${90}^{\circ }$. If BC = 25 cm, then what is the length of the median AD ?

Options

A. 24 cm
B. 12.5 cm
C. 14.5 cm
D. 10 cm

Answer

B

Explanation

To solve the problem, we need to find the length of the median AD in triangle ABC, where angle BAC is 90 degrees and the length of side BC is given as 25 cm.

1. Understand the Triangle Configuration:
- We have a right triangle ABC where angle BAC = 90 degrees.
- The sides are labeled as follows:
- A is the vertex at the right angle.
- B and C are the other two vertices.
- BC is the hypotenuse of the triangle.

2. Identify the Median:
- The median AD is the segment that connects vertex A to the midpoint D of side BC.

3. Use the Median Formula for Right Triangles:
- In a right triangle, the length of the median to the hypotenuse can be calculated using the formula:
\( \text{Median} = \frac{1}{2} \times \text{Hypotenuse} \)
- Here, the hypotenuse BC = 25 cm.

4. Calculate the Length of the Median:
- Substitute the value of the hypotenuse into the formula:
\( \text{Median AD} = \frac{1}{2} \times 25 \text{ cm} = 12.5 \text{ cm} \)

5. Conclusion:
- The length of the median AD is 12.5 cm.

Final Answer:
The length of the median AD is 12.5 cm.