दो संकेन्द्रीय वृत्ताकार चालक कुंडलियों को एक ही तल में रखा गया है, जिनकी त्रिज्याएँ व हैं। यदि, तब उनके बीच अन्योन्य प्रेरकत्व M समानुपाती होगा:
The correct Answer is:D
माना प्राथमिक कुंडली के केंद्र पर चुंबकीय क्षेत्र B है। इसे एकसमान मानते हुए, द्वितीयक कुंडली से गुजरने वाला चुंबकीय फ्लक्स है,
अब
Topper's Solved these Questions
Similar Questions
t = 0 और t=∞ पर बैटरी से प्रवाहित धारा के अनुपात की गणना कीजिए। (स्विच बंद करने का समय t = 0 है)
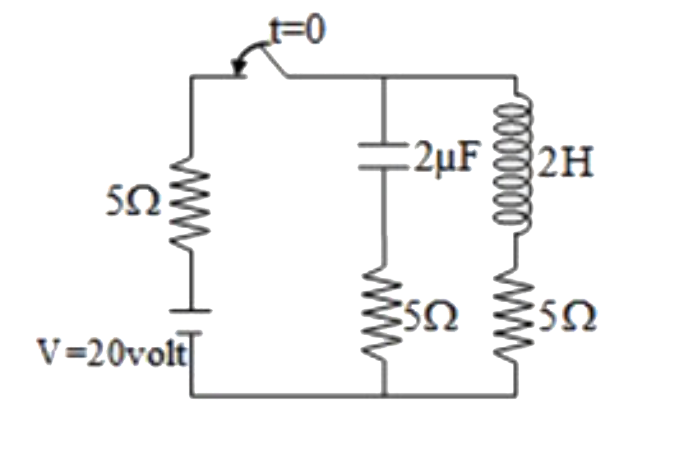
View SolutionHe4 नाभिक की त्रिज्या 3 फर्मी है। 82Pb206 नाभिक की त्रिज्या होगी:
View SolutionFor any three non-zero vectors r_1,r_2 and r_3 .|(r_1.r_1,r_1.r_2,r_1.r_3),(r_2.r_1,r_2.r_2,r_2.r_3),(r_3.r_1,r_3.r_2,r_3.r_3)|=0. Then which of the following is false
View SolutionIn ΔABC,r3+r1r2r1+r2=
View SolutionIn ΔABC,r1r2√[4R−r1−r2r1+r2]
View SolutionIn ΔABC,r2(r3+r1)√r1r2+r2r3+r3r1=
View SolutionIn the given loop the magnetic field at the centre O is 1) (mu_0I)/(4) ( (r_1 + r_2)/(r_1 r_2) ) out of the page 2) (mu_0I)/(4) ( (r_1 + r_2)/(r_1 r_2)) into the page 3) (mu_0I)/(4) ( (r_1 - r_2)/(r_1 r_2)) out of the page 4) (mu_0 I)/(4) ( (r_1 - r_2)/(r_1r_2) ) into the page
View SolutionIn a parallel arrangement if (R1>R2), then the power dissipated in resistance R1 will be
View SolutionΔ=r1r2√4R−r1−r2/r1+r2 అని చూపండి.
View Solutionr1r2+r3√r1r2+r2r3+r3r1=a అని రుజువు చేయండి.
View Solutionअन्योन प्रेरण तथा प्रेरकत्व | दो समतल वृत्ताकार कुंडलियों के बीच अन्योन प्रेरकत्व
View Solutionअन्योन प्रेरण तथा प्रेरकत्व || दो समतल वृत्ताकार कुंडलियों के बीच अन्योन प्रेरकत्व
View SolutionThe magnitudes of the gravitational fields at distances r1 and r2 from the centre of a uniform sphere of radius R and mass M are F1 and F2 respectively then
(a)F1F2=r1r2 if r1<R and r2<R
(b)F1F2=(r2r1)2 if r1>R and r2>R
(c)F1F2=r1r2 if r1>R and r2>R
(d)F1F2=(r1r2)2 if r1<R and r2<R
View Solution( r_1+ r_2) (r_2+r_3) (r_3+r_1) =
View Solutionरेडियो पदार्थ की t1 समय पर सक्रियता R1 तथा R2 समय पर R2 है (t2>t1) है तब R2R1 का अनुपात है:
View Solutionr1(r2+r3)√r1r2+r2r3+r1r3=
View Solution