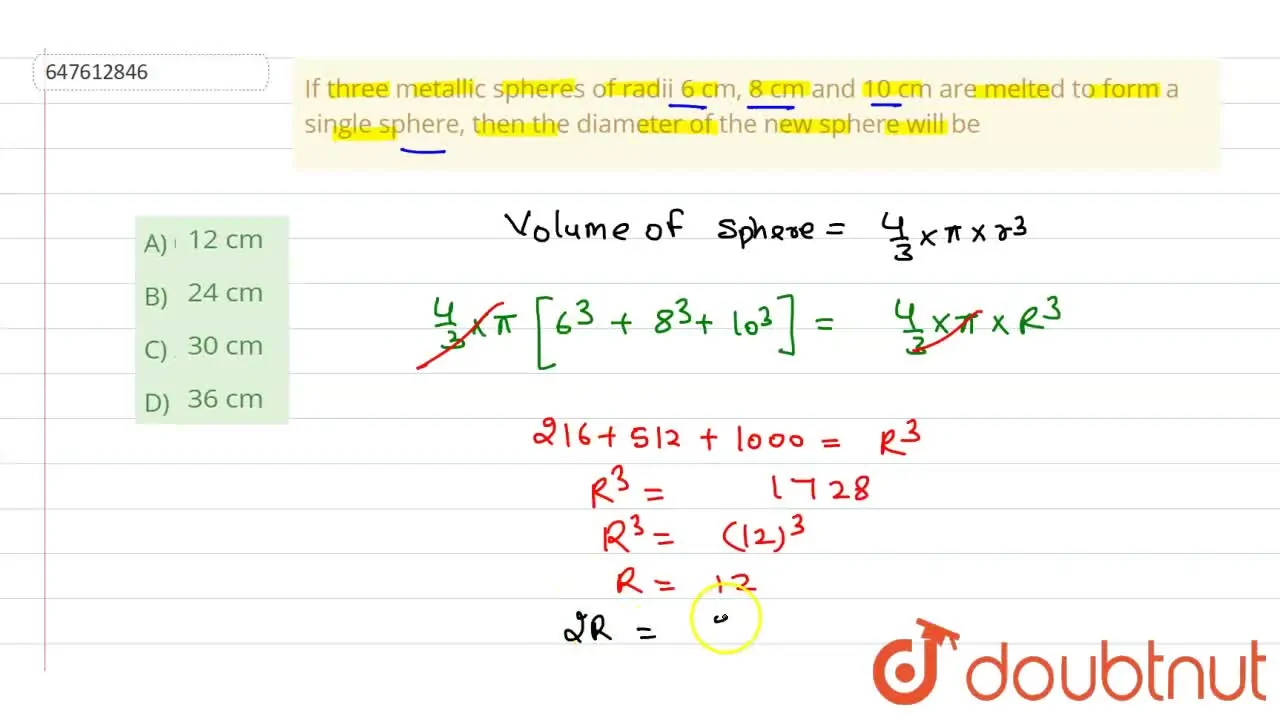
If three metallic spheres of radii 6 cm, 8 cm and 10 cm are melted to form a single sphere, then the diameter of the new sphere will be
12 cm
24 cm
30 cm
36 cm
Step by step video & image solution for If three metallic spheres of radii 6 cm, 8 cm and 10 cm are melted to form a single sphere, then the diameter of the new sphere will be by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Similar Questions
If three metallic spheres of radii 6 cms, 8 cms and 10 cms are melted to form a single sphere, the diameter of the new sphere will be (a) 12 cms (b) 24 cms (c) 30 cms (d) 36 cms
View SolutionIf three metallic spheres of radii 6 cm, 8 cm and 10 cm are melted to form a single sphere, the diameter of the sphere is (a) 12 cm (b) 24 cm (c) 30 cm (d) 36 cm
View SolutionThree solid spheres of diameters 6 cm, 8 cm and 10 cm are melted to form a single solid sphere. The diameter of the new sphere is
View SolutionThree small lead spheres of radii 3 cm, 4 cm and 5 cm respectively, are melted into a single sphere. The diameter of the new sphere is
View SolutionThree spheres of radii 3 cm, 4 cm and 5 cm are melted to form a new sphere. Find the radius of the new sphere.
View SolutionThree solid metallic spheres of diameters 6 cm, 8 cm and 10 cm are melted and recast into a new solid sphere. The diameter of the new sphere is :
View SolutionMetallic spheres of radii 6cm, 8cm and 10cm respectively are melted to form a solid sphere. Find the radius of the resulting sphere.
View SolutionThree metallic solid cubes of sides 6 cm, 8 cm and 10 cm are melted to form a single cube. The length of the edge of the new cube is
View SolutionMetallic spheres of radii 6 cm, 8 cm and 10 cm respectively, are melted to form a single solid sphere. Find the radius of the resulting sphere.
View SolutionMetallic spheres of radii 6 cm, 8 cm and 10 cm respectively, are melted to form a single solid sphere. Find the radius of the resulting sphere.
View Solution